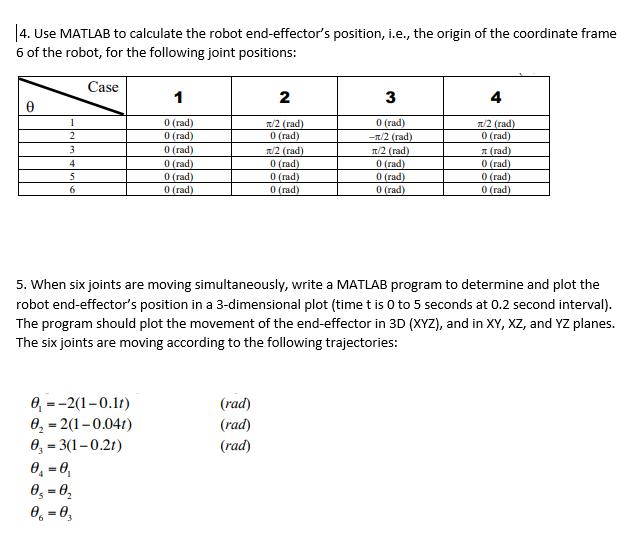
4. Use MATLAB to calculate the robot end-effector's position, i.e., the origin of the coordinate frame 6 of the robot, for the following joint positions: 0 1 2 3 4 5 6 Case 0 = -2(1-0.11) 0=2(1-0.041) 0,=3(1-0.21) 0-0 0-0 06-03 = 1 0 (rad) 0 (rad) 0 (rad) 0 (rad) 0 (rad) 0 (rad) 2 (rad) (rad) (rad) /2 (rad) 0 (rad) /2 (rad) 0 (rad) 0 (rad) 0 (rad) 3 0 (rad) -/2 (rad) 5. When six joints are moving simultaneously, write a MATLAB program to determine and plot the robot end-effector's position in a 3-dimensional plot (time t is 0 to 5 seconds at 0.2 second interval). The program should plot the movement of the end-effector in 3D (XYZ), and in XY, XZ, and YZ planes. The six joints are moving according to the following trajectories: r/2 (rad) 0 (rad) 0 (rad) 0 (rad) 4 /2 (rad) 0 (rad) (rad) 0 (rad) 0 (rad) 0 (rad)