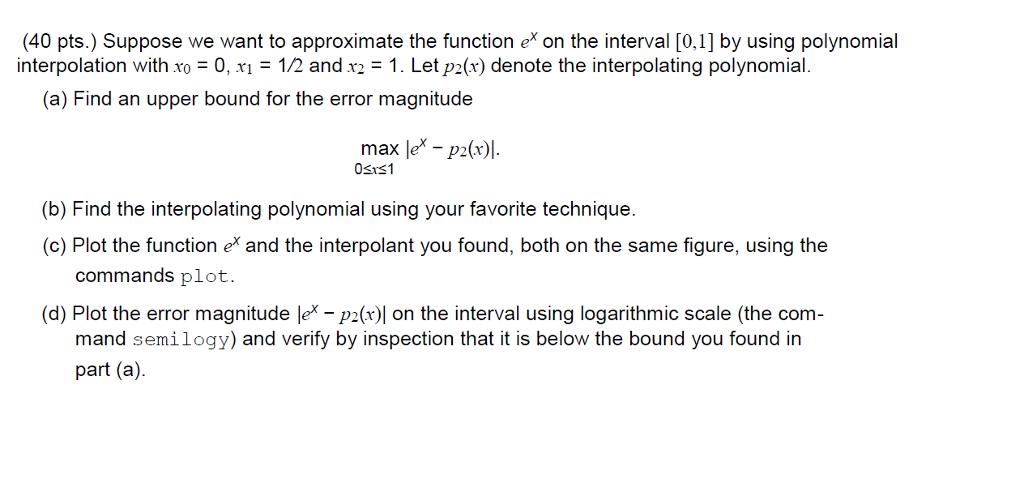
(40 pts.) Suppose we want to approximate the function ex on the interval [0,1] by using polynomial interpolation with x0 = 0, x1 = 1/2 and x2 = 1. Let p2(x) denote the interpolating polynomial. (a) Find an upper bound for the error magnitude max lex-p2(x)|. 0x1 (b) Find the interpolating polynomial using your favorite technique. (c) Plot the function ex and the interpolant you found, both on the same figure, using the commands plot. (d) Plot the error magnitude lex-p2(x) on the interval using logarithmic scale (the com- mand semilogy) and verify by inspection that it is below the bound you found in part (a).