4.1 STATISTICS
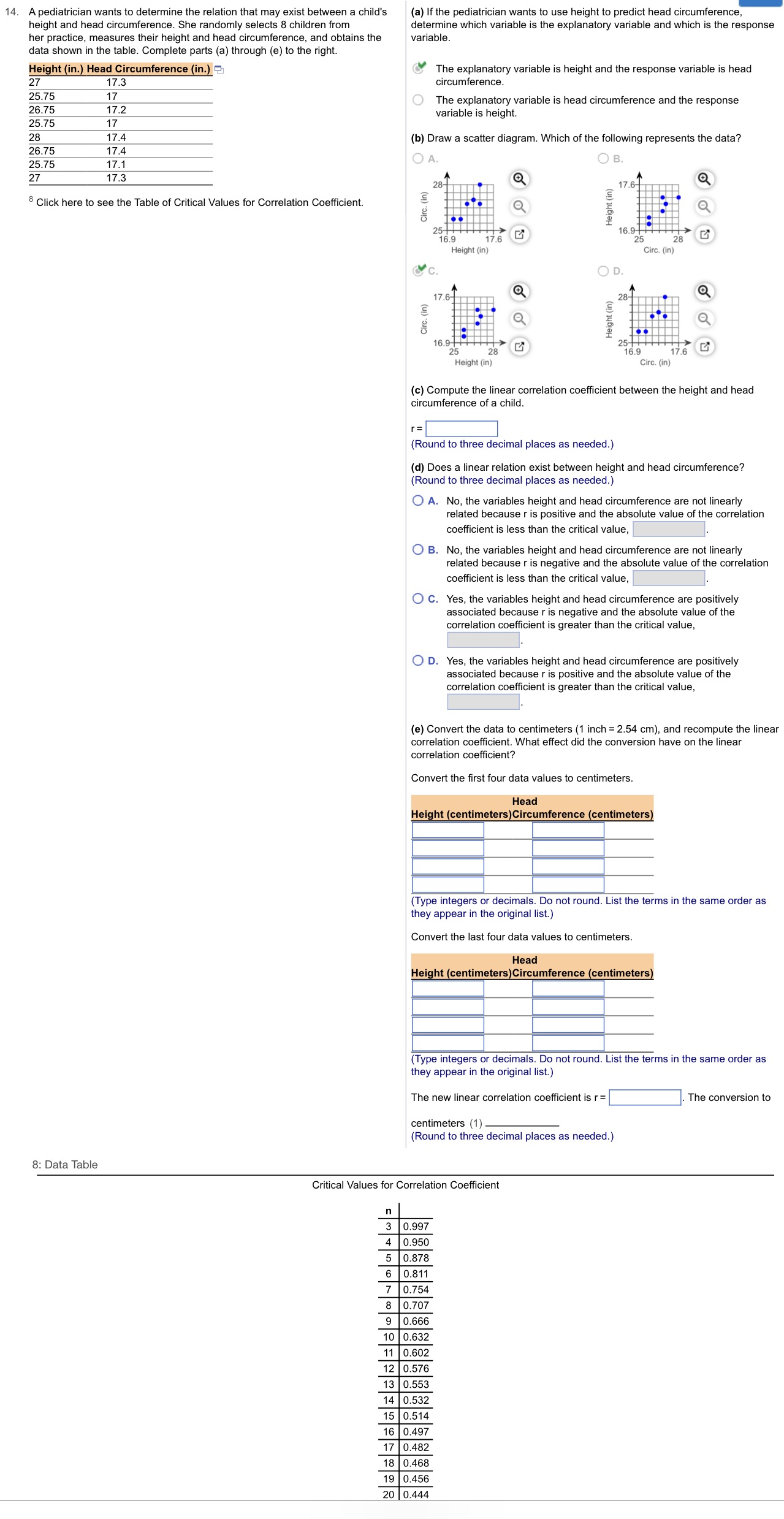
14. A pediatrician wants to determine the relation that may exist between a child's (a) If the pediatrician wants to use height to predict head circumference, height and head circumference. She randomly selects 8 children from determine which variable is the explanatory variable and which is the response her practice, measures their height and head circumference, and obtains the variable. data shown in the table. Complete parts (a) through (e) to the right. Height (in.) Head Circumference (in.) The explanatory variable is height and the response variable is head 27 17.3 circumference. 25.75 17 O The explanatory variable is head circumference and the response 26.75 17.2 variable is height. 25.75 17 28 17.4 (b) Draw a scatter diagram. Which of the following represents the data? 26.75 17.4 25.75 17.1 O A. B 27 17.3 28- 8 Click here to see the Table of Critical Values for Correlation Coefficient. c. (in Height (in) 257 6.97 16.9 17.6 Height (in) Circ. (in) VC OD. 28- Height (in 16.9 25-+ 25 16.9 17.6 C Height (in) Circ. (in) (c) Compute the linear correlation coefficient between the height and head circumference of a child. r= (Round to three decimal places as needed.) (d) Does a linear relation exist between height and head circumference? Round to three decimal places as needed.) O A. No, the variables height and head circumference are not linearly related because r is positive and the absolute value of the correlation coefficient is less than the critical value, O B. No, the variables height and head circumference are not linearly related because r is negative and the absolute value of the correlation coefficient is less than the critical value, O C. Yes, the variables height and head circumference are positively associated because r is negative and the absolute value of the correlation coefficient is greater than the critical value, O D. Yes, the variables height and head circumference are positively associated because r is positive and the absolute value of the correlation coefficient is greater than the critical value, (e) Convert the data to centimeters (1 inch = 2.54 cm), and recompute the linear correlation coefficient. What effect did the conversion have on the linear correlation coefficient? Convert the first four data values to centimeters. Head Height (centimeters) Circumference (centimeters) (Type integers or decimals. Do not round. List the terms in the same order as they appear in the original list.) Convert the last four data values to centimeters. Head Height (centimeters) Circumference (centimeters) (Type integers or decimals. Do not round. List the terms in the same order as they appear in the original list.) The new linear correlation coefficient is r= . The conversion to centimeters (1) (Round to three decimal places as needed.) 8: Data Table Critical Values for Correlation Coefficient n 3 0.997 4 0.950 5 0.878 6 0.811 7 0.754 8 0.707 9 0.666 10 0.632 11 0.602 12 0.576 13 0.553 14 0.532 15 0.514 16 0.497 17 0.482 18 0.468 19 0.456 20 0.444