Question
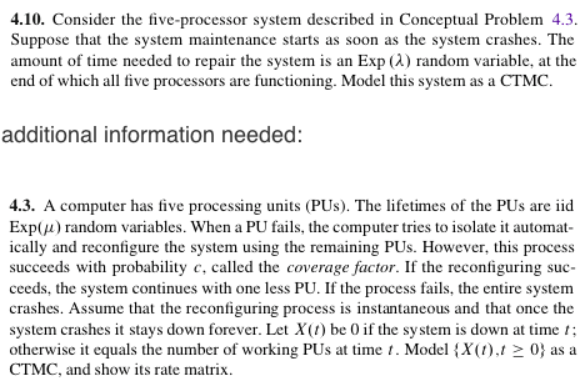
4.10. Consider the five-processor system described in Conceptual Problem 4.3. Suppose that the system maintenance starts as soon as the system crashes. The amount of
4.10. Consider the five-processor system described in Conceptual Problem 4.3.
Suppose that the system maintenance starts as soon as the system crashes. The
amount of time needed to repair the system is an Exp(lambda) random variable, at the
end of which all five processors are functioning. Model this system as a CTMC.
Using
4.3. A computer has five processing units (PUs). The lifetimes of the PUs are iid
Exp(mu) random variables.When a PU fails, the computer tries to isolate it automatically
and reconfigure the system using the remaining PUs. However, this process
succeeds with probability c, called the coverage factor. If the reconfiguring succeeds,
the system continues with one less PU. If the process fails, the entire system
crashes. Assume that the reconfiguring process is instantaneous and that once the
system crashes it stays down forever. Let X(t) be 0 if the system is down at time t ;
otherwise it equals the number of working PUs at time t . Model {X(t); t >= 0} as a
CTMC, and show its rate matrix.
Here's the question from the textbook (I'm trying to insert pictures but they just disappear, so here's a drive link to the pics):
https://drive.google.com/open?id=1Pm5lK3gDUhwZHVVRdMcDl2-fMi15w-hh
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


