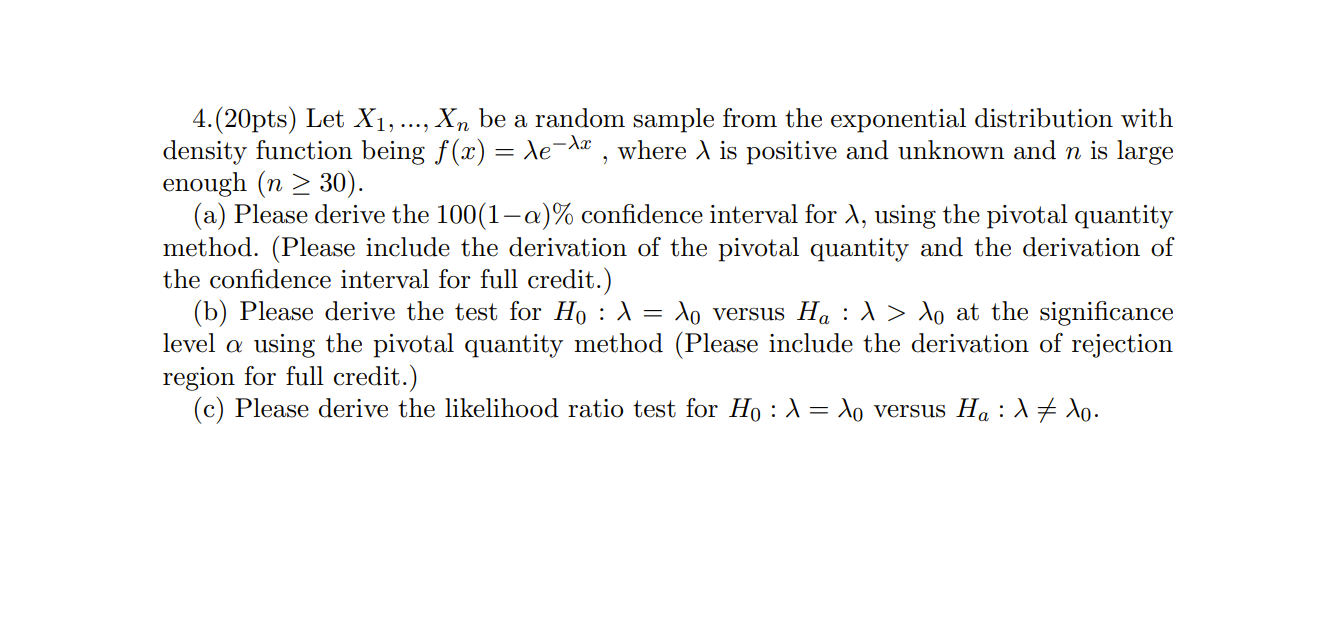
4.(20pts) Let X1, ..., Xn be a random sample from the exponential distribution with density function being f(x) = ?e??x , where ? is positive and unknown and n is large enough (n ? 30). (a) Please derive the 100(1??)% confidence interval for ?, using the pivotal quantity method. (Please include the derivation of the pivotal quantity and the derivation of the confidence interval for full credit.) (b) Please derive the test for H0 : ? = ?0 versus Ha : ? > ?0 at the significance level ? using the pivotal quantity method (Please include the derivation of rejection region for full credit.) (c) Please derive the likelihood ratio test for H0 : ? = ?0 versus Ha : ? 6= ?0.

4. (20pts) Let X1, ..., Xn be a random sample from the exponential distribution with density function being f(x) = de-, where A is positive and unknown and n is large enough (n > 30). (a) Please derive the 100(1-a)% confidence interval for 1, using the pivotal quantity method. (Please include the derivation of the pivotal quantity and the derivation of the confidence interval for full credit.) (b) Please derive the test for Ho : A = do versus Ha : > > do at the significance level a using the pivotal quantity method (Please include the derivation of rejection region for full credit.) (c) Please derive the likelihood ratio test for Ho : A = do versus Ha : > # do