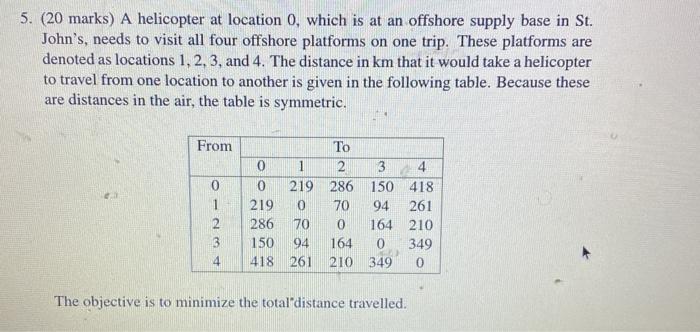
5. (20 marks) A helicopter at location 0, which is at an offshore supply base in St. John's, needs to visit all four offshore platforms on one trip. These platforms are denoted as locations 1, 2, 3, and 4. The distance in km that it would take a helicopter to travel from one location to another is given in the following table. Because these are distances in the air, the table is symmetric. From 0 1 2 0 1 2 3 4 0 219 286 150 418 219 0 70 94 261 286 70 0 164 210 150 94 164 0 349 418 261 210 349 0 4 The objective is to minimize the total distance travelled. (a) Give the algebraic model of this situation, omitting the sub-tour constraints. You are not required to submit this part, except that the list of variables must be submitted in Word or pdf, or these must appear at the top of the file in part (b). (b) Use LINGO or the Excel Solver to solve this partial) model. Show that this solution is not a tour, and state the two sub-tours. (c) Give a constraint that would, if added to the formulation of (b), prevent this specific situation from arising. Re-solve the computer model, and state whether or not this new solution is a tour. (d) If necessary, repeat (c) until a tour is obtained. 5. (20 marks) A helicopter at location 0, which is at an offshore supply base in St. John's, needs to visit all four offshore platforms on one trip. These platforms are denoted as locations 1, 2, 3, and 4. The distance in km that it would take a helicopter to travel from one location to another is given in the following table. Because these are distances in the air, the table is symmetric. From 0 1 2 0 1 2 3 4 0 219 286 150 418 219 0 70 94 261 286 70 0 164 210 150 94 164 0 349 418 261 210 349 0 4 The objective is to minimize the total distance travelled. (a) Give the algebraic model of this situation, omitting the sub-tour constraints. You are not required to submit this part, except that the list of variables must be submitted in Word or pdf, or these must appear at the top of the file in part (b). (b) Use LINGO or the Excel Solver to solve this partial) model. Show that this solution is not a tour, and state the two sub-tours. (c) Give a constraint that would, if added to the formulation of (b), prevent this specific situation from arising. Re-solve the computer model, and state whether or not this new solution is a tour. (d) If necessary, repeat (c) until a tour is obtained