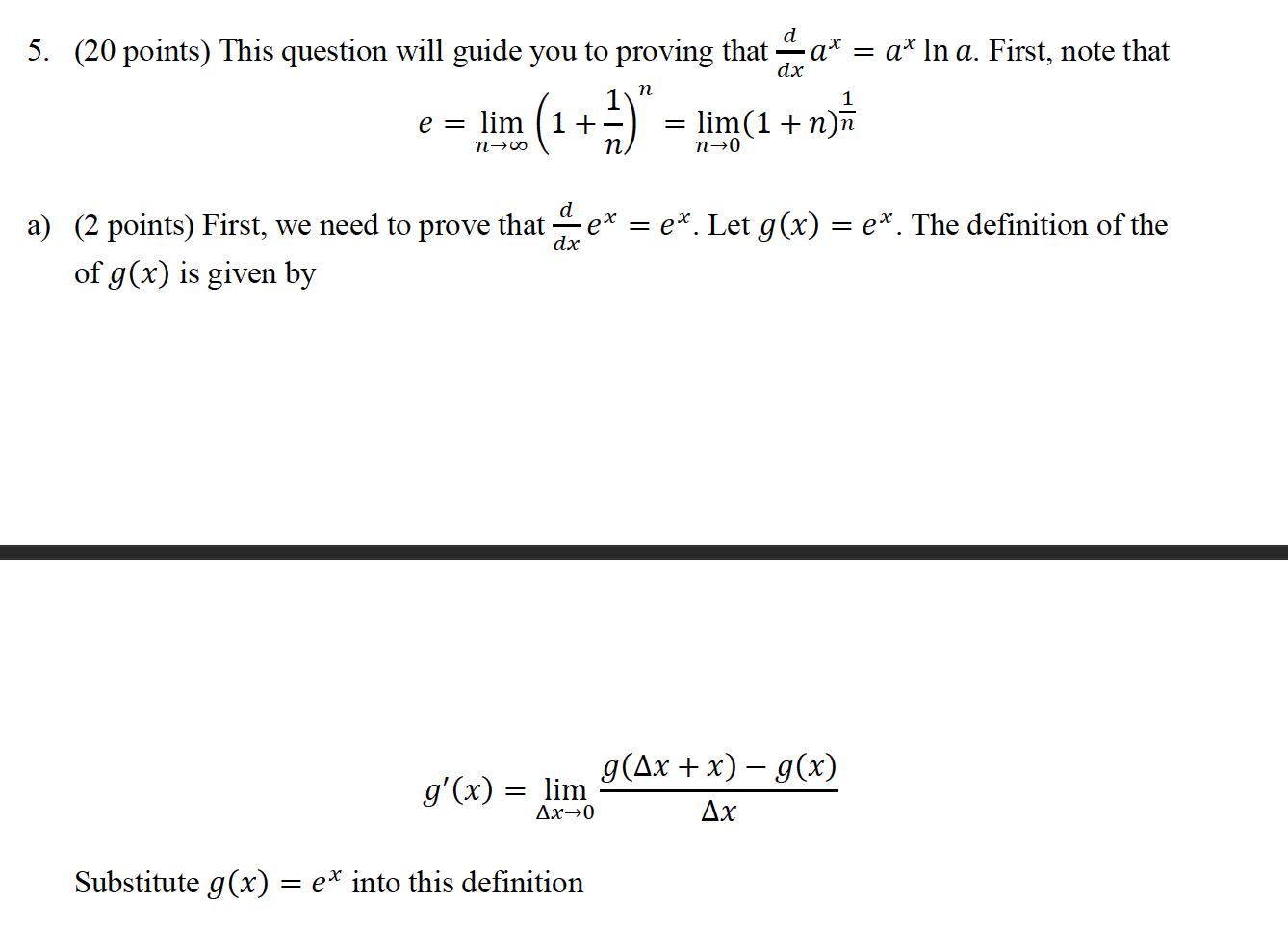
5. (20 points) This question will guide you to proving that ax dx 1) ' e = lim (1 + N0 g'(x) = lim Ax0 n Substitute g(x) = e* into this definition = lim(1 + n) n0 = d a) (2 points) First, we need to prove that e* = e*. Let g(x) = e*. The definition of the of g(x) is given by dx g(x + x) g(x) Ax 1 a* In a. First, note that b) (2 points) Using rules of exponents, factor ex out of the limit c) (4 points) We are going to apply a "change of variables" to this limit. Let n = e^x 1, thus Ax = In(n + 1). Now, rewrite the expression from part b) in terms of n. (Hint: you also need to write the limit in terms of n. To do this, think, as Ax 0, what value does n approach? You can then write the new limit as n approaches this value). d) (4 points) Divide both the numerator and denominator of your expression by n, then use rules of logarithms to write the denominator as In [(n + -1)]. (Be sure to specify which rule you are using). e) (4 points) Evaluate this limit using rules of limits and the definition of e above to show d = ex. (Be sure to specify which rules of limits you are using). dx d dx f) (2 points) Now, we can evaluate a*. Using rules of logarithms and exponents show that (be sure to specify the rules you use) ax d dx g) (2 points) Since - -ax dex In a, use the chain rule and the result from part e) to show dx d - ax = ex In a dx a* In a