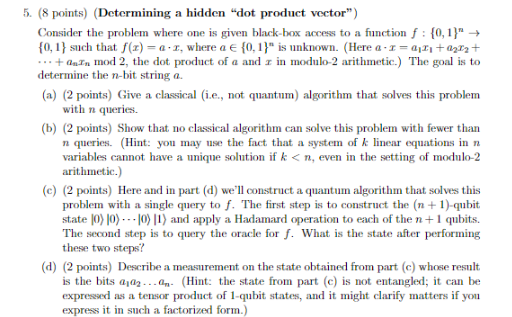
5. (8 points) (Determining a hidden "dot product vector") Consider the problem where one is given black-box access to a function f: {0, 1}" {0, 1} such that f(x) = a-z, where a {0,1}" is unknown. (Here a-1 = +2 + ...+ ann mod 2, the dot product of a and z in modulo-2 arithmetic.) The goal is to determine the n-bit string a. (a) (2 points) Give a classical (i.e., not quantum) algorithm that solves this problem with n queries. (b) (2 points) Show that no classical algorithm can solve this problem with fewer than n queries. (Hint: you may use the fact that a system of k linear equations in n variables cannot have a unique solution if k