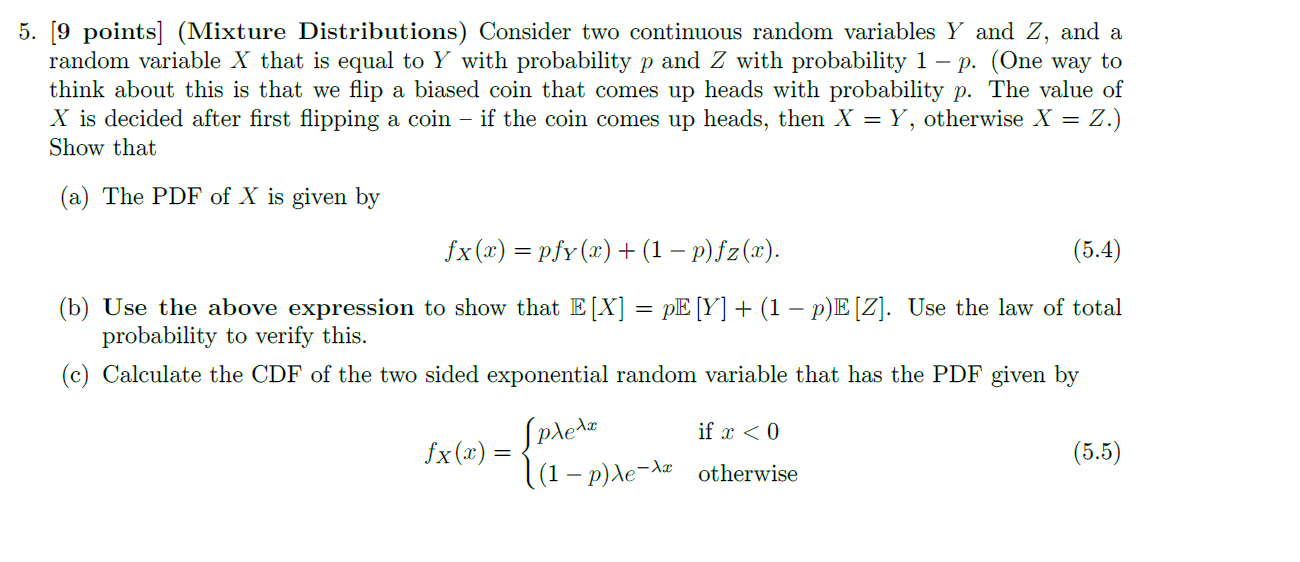
5. [9 points] (Mixture Distributions) Consider two continuous random variables Y and Z, and a random variable X that is equal to Y with probability p and Z with probability 1 - p. (One way to think about this is that we flip a biased coin that comes up heads with probability p. The value of X is decided after first flipping a coin - if the coin comes up heads, then X = Y, otherwise X = Z.) Show that (a) The PDF of X is given by fx(x) = pfy(x) + (1 p)z(x). (5.4) (b) Use the above expression to show that E[X] = pE [Y] + (1 p)E [Z]. Use the law of total probability to verify this. (c) Calculate the CDF of the two sided exponential random variable that has the PDF given by if x 0 fx(x) = [(1 - p) Ae-Ax otherwise (5.5)