Question: (5) A square matrix A is called nilpotent if there exists a positive integer k such that Ak = 0 where 0 is the

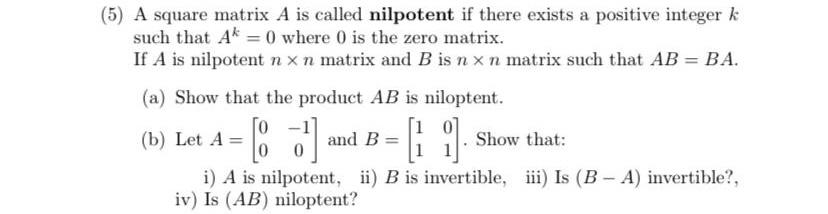
(5) A square matrix A is called nilpotent if there exists a

(5) A square matrix A is called nilpotent if there exists a positive integer k such that Ak = 0 where 0 is the zero matrix. If A is nilpotent n xn matrix and B is n x n matrix such that AB = BA. (a) Show that the product AB is niloptent. [0 (b) Let A = Show that: and B i) A is nilpotent, ii) B is invertible, ii) Is (B A) invertible?, iv) Is (AB) niloptent?

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

r det BA 1 0 BA is nol in vertibe A... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

A square matrix A is called nilpotent if Ak = O for some k ¥ 1 (a) Show that is nilpotent. (b) Show that any strictly upper triangular matrix is nilpotent. (c) Find a nilpotent matrix which is...

A square matrix A is called nilpotent if Ak = O for some k 1. (a) Prove that the only eigenvalue of a nilpotent matrix is 0. (The converse is also true; see Exercise 8.6.18.) (b) Find examples where...

A square matrix A is called idempotent if A2 = A. (The word idempotent comes from the Latin idem, meaning "same;' and potere, meaning "to have power:' thus, something that is idempotent has the "same...

For the universe of all integers, let p(x), q(x), r(x), s(x), and r(x) be the following open statements. p(x): x > 0 q(x): x is even r (x): x is a perfect square s(x): x is (exactly) divisible by 4 f...

I need answers of these questions ASAP !! PLEASE. Note: this is complete question and i have attached references 6A. How is the Pigou tax computed? What is its aim? SB. If a competitive consumer...

I need answers of these questions ASAP !! PLEASE. Note: this is complete question and i have attached references REVEALED PREFERENCE RELATIONS AND THE AXIOMS OF REVEALED PREFERENCE A pure exchange...

I need answers of these questions ASAP !! PLEASE. Note: this is complete question and i have attached referen 4A. State the "Walras Iaw' and mention (and prove) an important eon-tiller)r of it...

hey guys, i need help coding B) and C) and easy code using python please l. Nilpotent Matrices 1. A matrix MMnn is called nilpotent if there is a positive integer n such that Mn=0, where 0 is the...

Homework 3 (Extra Credit) Your job as aspiring mathematicians will be to answer similar questions for Hamiltonian vector fields. In this homework assignment you will investigate properties of...

Requirements: 1) Structure: Your application must consist of the following TWO Java classes: An AgeClientFL client class (created from your program template). A UtilsFL utilities class (created by...

What is a certificate of origin, a commercial invoice, and a shippers export declaration?

The cost of a particular private school begins with a one-time initiation fee of $1000, plus annual tuition of $6,000. This situation can be modeled by a linear function. Identify the independent and...

Evaluate the integral. ( R e m e m b e r the constant o f integration. ) x + 1 6 x 2 + 1 4 x + 5 3 d x

A client has a history of heart disease, what should the trainer request? 12 min run/ walk test Medical clearance Signed waiver PAR-Q form

1. A benefit (for a research study) is defined as __________. a. a valued or desired outcome. b. the probability of harm or injury from participation in a research study. c. financial compensation in...

A client is 45 years old and has a resting heart rate of 75 bpm. Using the Karvonen formula, what is their target heart rate at an exercise intensity of 60%? 145 155 165 135

Myofibrils are constructed of overlapping strands of protein polymers known as which of the following? Actin and tropomyosin Tropin and myosin Tropoactin and myosin Actin and myosin

Which assessment allows a trainer to understand a client's lean body and total fat mass? Body fat calculation Girth measurements Scale weight Photographs

All of the following facts about tonic receptors are true, except for which statement? Question 4 options: When a stimulus increases or decreases, the rate of action potential generation changes....

chapter 5 INTRODUCTION TO MATRIX ALGEBRA GOALS The purpose of this chapter is to introduce you to matrix algebra, which has many applications. You are already familiar with several algebras:...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

5 A square matrix is said to be doubly stochastic if its entries are all nonnegative and the entries in each row and each column sum to 1. For any ergodic, doubly stochastic matrix, show that all...

Please answer the section about scaling. I keep getting a recycled answer for just the first part. a EXERCISE 5 (5 points) Difficulty: Hard Theory: A vector with nonnegative entries is called a...

Create the following function in MATLAB: Theory: A vector with nonnegative entries is called a probability vector if the sum of its entries is 1. A square matrix is called right stochastic if its...

5 (**). Hitori is a simple puzzle game played on an N N grid of positive integers. The idea is simply to cross out integers in the grid (as few as possible) so that: Any given number appears at most...