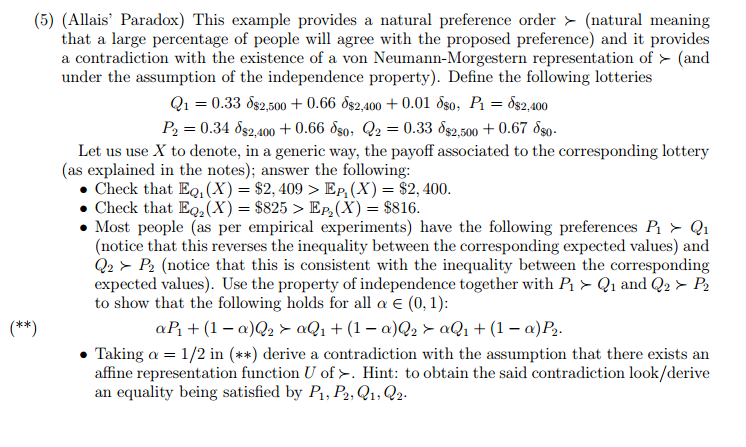
(**) (5) (Allais' Paradox) This example provides a natural preference order (natural meaning that a large percentage of people will agree with the proposed preference) and it provides a contradiction with the existence of a von Neumann-Morgestern representation of (and under the assumption of the independence property). Define the following lotteries Q1 = 0.33 $2,500+ 0.66 $2,400 + 0.01 880, P = 582,400 P2 = 0.34 $2,400 +0.66 880; Q2 = 0.33 8$2,500 + 0.67 880- Let us use X to denote, in a generic way, the payoff associated to the corresponding lottery (as explained in the notes); answer the following: Check that EQ, (X) = $2,409 > EP, (X) = $2,400. Check that EQ2(X) = $825 > Ep2(X) = $816. Most people (as per empirical experiments) have the following preferences P > Q (notice that this reverses the inequality between the corresponding expected values) and Q2 P2 (notice that this is consistent with the inequality between the corresponding expected values). Use the property of independence together with P Q and Q2 > P2 to show that the following holds for all a (0, 1): aP + (1 a)Q aQ + (1 a) Q2 > aQ + (1 a)P2. Taking a = 1/2 in (**) derive a contradiction with the assumption that there exists an affine representation function U of>. Hint: to obtain the said contradiction look/derive an equality being satisfied by P1, P2, Q1, Q2-