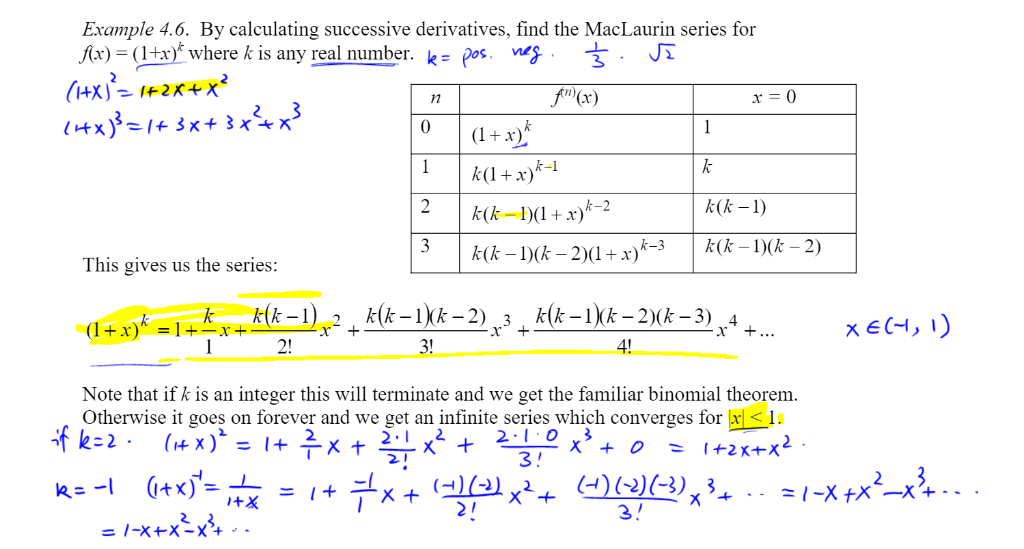
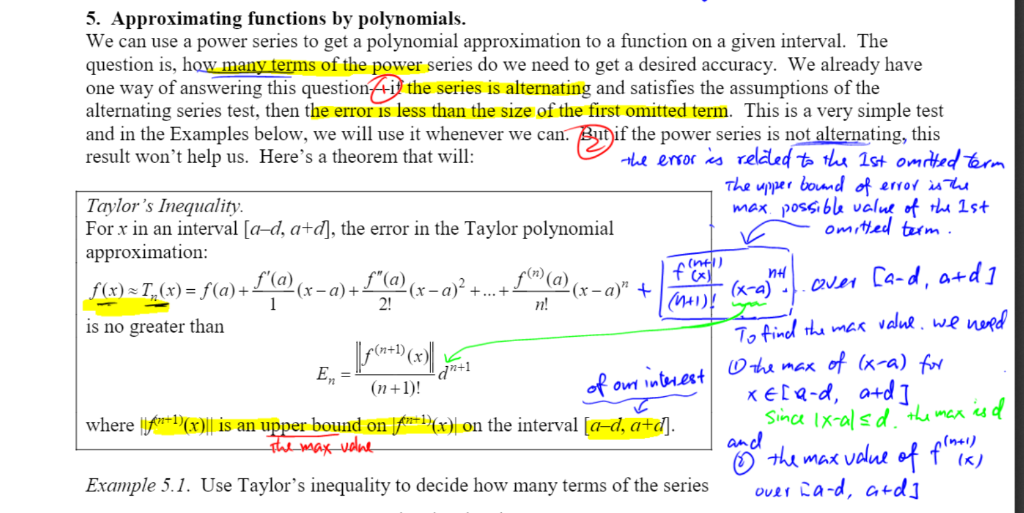
5. Approximate the function fx) v by T4(x), the Taylor polynomial of degree 4 centred atx-1. Do this in two ways: (a) Use the general formula at the top of page 59-- calculating successive derivatives of vx. (b) Change variable so you can directly use the formula of Ex 4.6. (c) How accurate is this approximation on the interva 4 [1/2, 3/2]? When x> 1, use the alternating series error estimate; when x0: 4(x-of = f(x) Note then k! and the polynomial of dse n k! is called the nth degree Taylor pohnomal for at the point x=a. The infinite series is simply called the Taylor series forfcentred at x=a Note: the special case E0 is sufficiently important it is given a MacLaurin serjes for name of its own and is called the Example 4.2. (a) Find the MacLaurin series for fc)-e 2 n-l Example 4.6. By calculating successive derivatives, find the MacLaurin series for fx) = (1+x) where k is any real number. k tos . J f" (x) 0 (d+x) 1kx) k-1 k(k-1) This gives us the series 2! 4! Note that if k is an integer this will terminate and we get the familiar binomial theorem Otherwise it goes on forever and we get an infinite series which converges for 1 2 5. Approximating functions by polynomials. We can use a power series to get a polynomial approximation to a function on a given interval. The question is, how many terms of the power series do we need to get a desired accuracy. We already have one way of answering this question4i the series is alternating and satisfies the assumptions of the alternating series test, then the error is less than the size of the first omitted term. This is a very simple test and in the Examples below, we will use it whenever we ca result won't help us. Here's a theorem that will if the power series is not alternating, this .hd erso.es redad.t-dalat omdted The upper bond of o max possibl valu of hu 1st Taylor 's Inequality For x in an interval [a-d, a+d], the error in the Taylor polynomial approximation f (a) 2! f (a) (x 71 is no greater than Kela-d, a+d Sinc Ix-as where Hrmb(X)II is an upper bound on lemnx on the interval [a d, at] 24 Example 51. Use Taylor's inequality to decide how many terms of the series a-d, a tdI oul ' 5. Approximate the function fx) v by T4(x), the Taylor polynomial of degree 4 centred atx-1. Do this in two ways: (a) Use the general formula at the top of page 59-- calculating successive derivatives of vx. (b) Change variable so you can directly use the formula of Ex 4.6. (c) How accurate is this approximation on the interva 4 [1/2, 3/2]? When x> 1, use the alternating series error estimate; when x0: 4(x-of = f(x) Note then k! and the polynomial of dse n k! is called the nth degree Taylor pohnomal for at the point x=a. The infinite series is simply called the Taylor series forfcentred at x=a Note: the special case E0 is sufficiently important it is given a MacLaurin serjes for name of its own and is called the Example 4.2. (a) Find the MacLaurin series for fc)-e 2 n-l Example 4.6. By calculating successive derivatives, find the MacLaurin series for fx) = (1+x) where k is any real number. k tos . J f" (x) 0 (d+x) 1kx) k-1 k(k-1) This gives us the series 2! 4! Note that if k is an integer this will terminate and we get the familiar binomial theorem Otherwise it goes on forever and we get an infinite series which converges for 1 2 5. Approximating functions by polynomials. We can use a power series to get a polynomial approximation to a function on a given interval. The question is, how many terms of the power series do we need to get a desired accuracy. We already have one way of answering this question4i the series is alternating and satisfies the assumptions of the alternating series test, then the error is less than the size of the first omitted term. This is a very simple test and in the Examples below, we will use it whenever we ca result won't help us. Here's a theorem that will if the power series is not alternating, this .hd erso.es redad.t-dalat omdted The upper bond of o max possibl valu of hu 1st Taylor 's Inequality For x in an interval [a-d, a+d], the error in the Taylor polynomial approximation f (a) 2! f (a) (x 71 is no greater than Kela-d, a+d Sinc Ix-as where Hrmb(X)II is an upper bound on lemnx on the interval [a d, at] 24 Example 51. Use Taylor's inequality to decide how many terms of the series a-d, a tdI oul