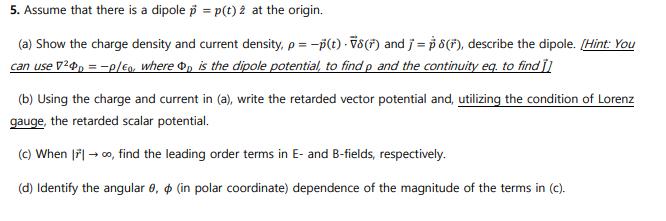
5. Assume that there is a dipole p = p(t) 2 at the origin. (a) Show the charge density and current density, p = -p(t) - V8(F) and j = p 8(F), describe the dipole. (Hint: You can use V2, = -plen, where on is the dipole potential, to find p and the continuity eq. to find ] (b) Using the charge and current in (a), write the retarded vector potential and, utilizing the condition of Lorenz gauge, the retarded scalar potential. (c) When (7| - o, find the leading order terms in E- and B-fields, respectively. (d) Identify the angular 0, (in polar coordinate) dependence of the magnitude of the terms in (c).