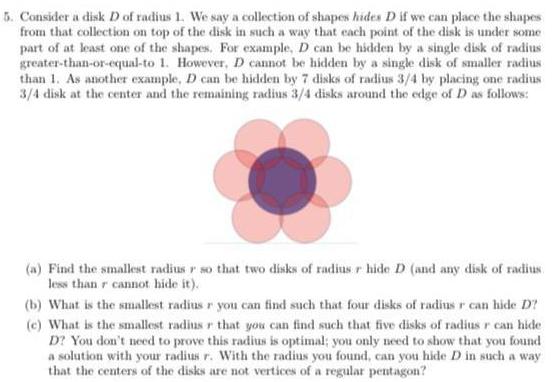
5 Consider a disk D of radius 1 We say a collection of shapes hides D if we can place the shapes from that collection on top of the disk in such a way that each point of the disk is under some part of at least one of the shapes For example D can be hidden by a single disk of radius greater than or equal to 1 However D cannot be hidden by a single disk of smaller radius than 1 As another example D can be hidden by 7 disks of radius 3 4 by placing one radius 3 4 disk at the center and the remaining radius 3 4 disks around the edge of D as follows a Find the smallest radius r so that two disks of radius r hide D and any disk of radius less than r cannot hide it b What is the smallest radius r you can find such that four disks of radius r can hide D c What is the smallest radius r that you can find such that five disks of radius r can hide D You don t need to prove this radius is optimal you only need to show that you found a solution with your radius r With the radius you found can you hide D in such a way that the centers of the disks are not vertices of a regular pentagon