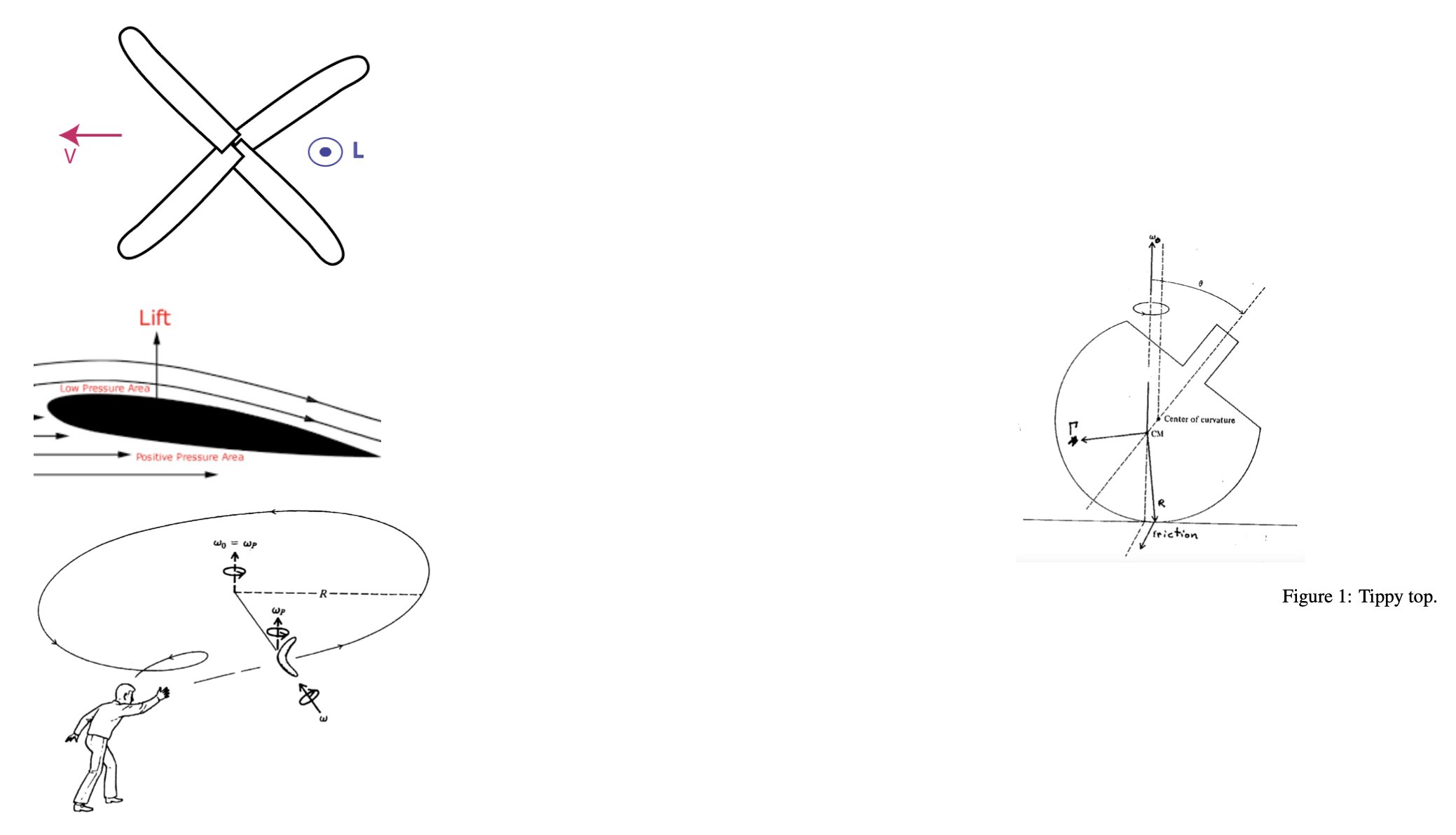
5. *Half-sphere tippy top. Set up the integrals and calculate the moment of inertia of a half-sphere tippy top about its symmetry axis. [Answer: Ma] 6. **Tippy top. A tippy top is a nearly spherical top that turns itself upside-down when spun on a smooth table. The torque that causes the top to flip is due to a small frictional force at the point of contact with the table. This frictional force is parallel to the table, opposing the velocity of slipping, as illustrated in the figure. The top itself consists of a sphere with a portion removed to expose the stem." The stem is used to spin the top. Initially the stem points up. When the top is released, the angle between the stem and the vertical steadily increases until the top flips over. View the video that comes up first in a google search for "tippe top SMU." (a) Order the moments of inertia along your three principal axes. (Which is larger, which is smaller, which is equal?) Select 3 as your symmetry axis. Select the other axes so initially w = wo (sin 0, 0, cos 0). Sketch these principal axes on top of the figure. (b) Use Euler's equation for one of your axes to show that ~ F/(Iwo). [Hint: differentiate w to get something that depends upon 0.] (c) Show that the top takes approximately t~ :2 Rwo/(5g) to flip over. (d) Based on the video, write down reasonable estimates for R, wo and and use these to estimate the predicted flipping time. How long does it take to flip in the video? Is your estimate the correct order of magnitudee? (e) As the video states, the top slows down when it flips over. Neglecting dissi- pation, use energy conservation to estimate the ratio of the angular velocity Wup to the initial angular velocity wo immediately after tipping in terms of the change in height of the top's center of mass h and other parameters. X Low Pressure Area Lift Positive Pressure Area WO = wp w * CM Center of curvature R friction Figure 1: Tippy top.