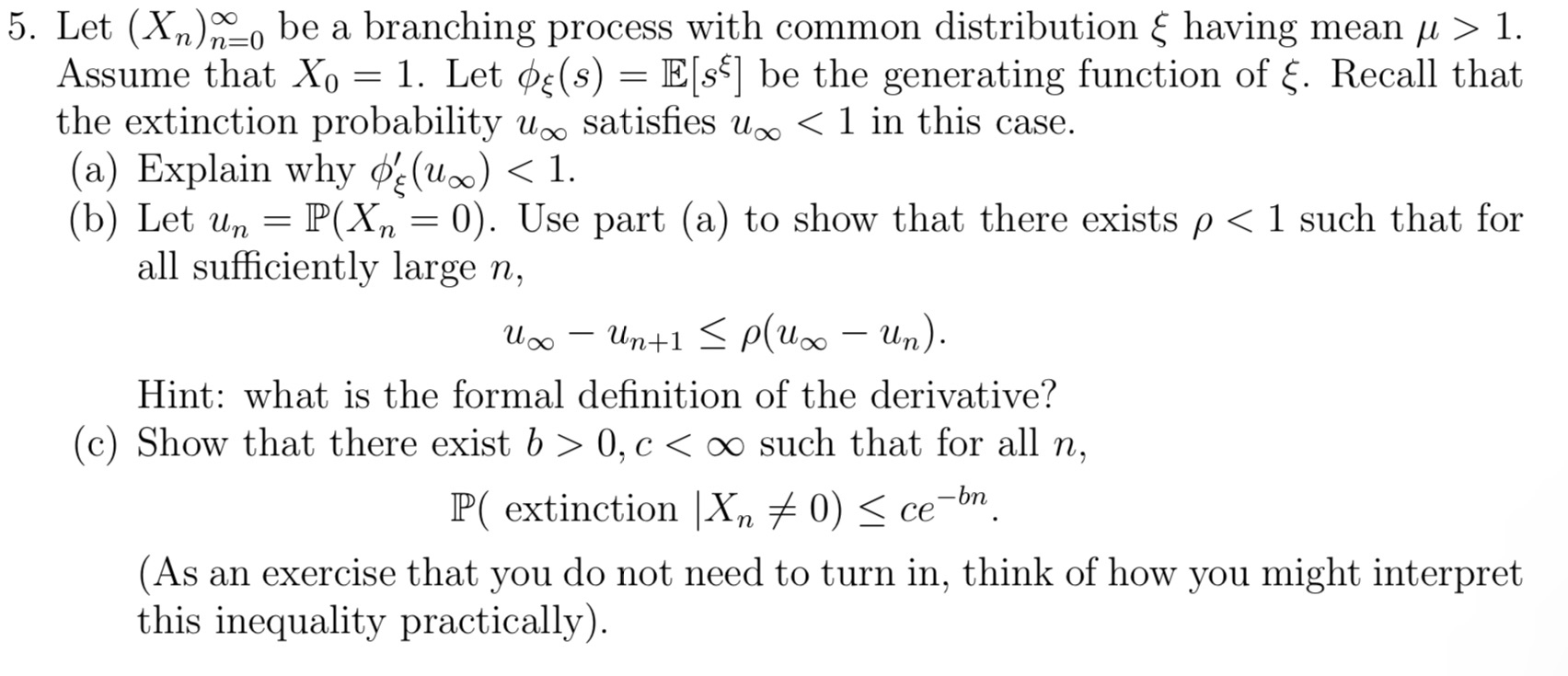
5. Let (X) be a branching process with common distribution & having mean > 1. Assume that X = 1. Let o(s) = E[s] be the generating function of . Recall that the extinction probability u satisfies u < 1 in this case. (a) Explain why o'(u) < 1. (b) Let un P(X = 0). Use part (a) to show that there exists p < 1 such that for = n all sufficiently large n, U - Un+1 p (u - Un). Hint: what is the formal definition of the derivative? (c) Show that there exist b > 0, c < such that for all n, P( extinction |n = 0) cebn. (As an exercise that you do not need to turn in, think of how you might interpret this inequality practically).