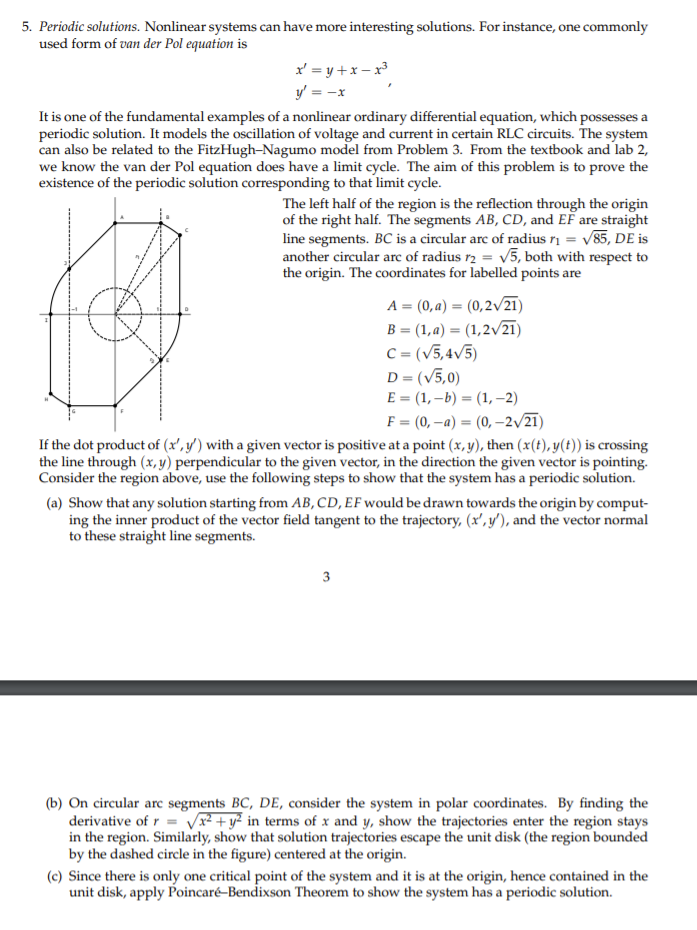
5. Periodic solutions. Nonlinear systems can have more interesting solutions. For instance, one commonly used form of van der Pol equation is x = y + x - 3 y' = - * It is one of the fundamental examples of a nonlinear ordinary differential equation, which possesses a periodic solution. It models the oscillation of voltage and current in certain RLC circuits. The system can also be related to the FitzHugh-Nagumo model from Problem 3. From the textbook and lab 2, we know the van der Pol equation does have a limit cycle. The aim of this problem is to prove the existence of the periodic solution corresponding to that limit cycle. The left half of the region is the reflection through the origin of the right half. The segments AB, CD, and EF are straight line segments. BC is a circular arc of radius 11 = 85, DE is another circular are of radius r2 = V5, both with respect to the origin. The coordinates for labelled points are A = (0,a) = (0,2721) B = (1,a) = (1,2721) C=(5,475) D=(5,0) E = (1, -b) = (1, -2) F = (0, -a) = (0, -221) If the dot product of (x,y) with a given vector is positive at a point (x,y), then (x(t), y(t)) is crossing the line through (x,y) perpendicular to the given vector, in the direction the given vector is pointing. Consider the region above, use the following steps to show that the system has a periodic solution. (a) Show that any solution starting from AB, CD, EF would be drawn towards the origin by comput- ing the inner product of the vector field tangent to the trajectory, (x',y'), and the vector normal to these straight line segments. 3 (b) On circular arc segments BC, DE, consider the system in polar coordinates. By finding the derivative of r = x2 + y2 in terms of x and y, show the trajectories enter the region stays in the region. Similarly, show that solution trajectories escape the unit disk (the region bounded by the dashed circle in the figure) centered at the origin. (c) Since there is only one critical point of the system and it is at the origin, hence contained in the unit disk, apply Poincar-Bendixson Theorem to show the system has a periodic solution