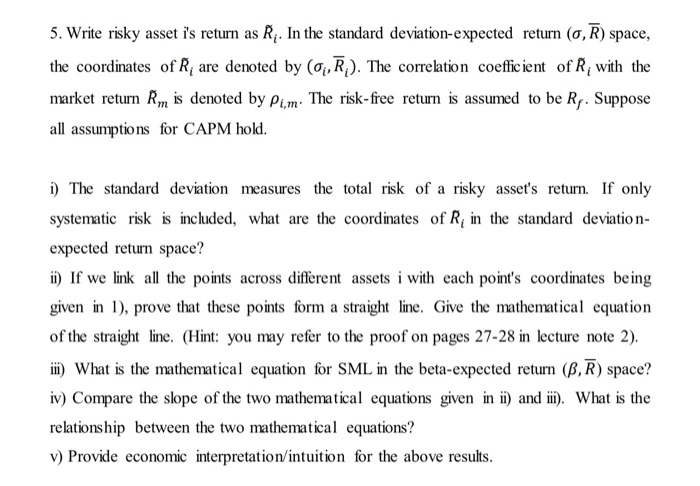


5. Write risky asset i's return as R . . In the standard deviation - expected return ( J . R ) space , the coordinates of' R, are donated by ( J . R. J. The correlation coefficient of R ; with the market return R is denoted by pim . The risk- free return is assumed to be Ry. Suppose all assumptions for CAPNT hold . 1 ) The standard deviation measures the total risk of a risky asset's return . If only* systematic risk is included , what are the coordinates of R, in the standard deviation - expected return space?" in If we link all the points across different assets i with cach point's coordinates being* given in I ), prove that these points form a straight line . Give the mathematical equation of the straight line . ( Hint : you may refer to the proof on pages 27 - 28 in lecture note 2 ) . In1 ) What is the mathematical equation for SMIL in the beta-expected return ( B , R ) space ? iv s Compare the slope of the two mathematical equations given in In ) and ill ) . What is the relationship between the two mathematical equations ! V ) Provide economic interpretation intuition for the above results .d. As the tangency portfolio T is unique, some natural questions arise, for example, what the property of T is and whether there is any direct relationship between T and other assets or portfolios in the market. Indeed, the most important property of tangency portfolio is Capital Asset Pricing Model (CAPM) equation, which states that there exists a liner relation of T's return with the return of any other asset or portfolio in the market. Specifically, E(R,) = R, +[E(RT) - R, ] COV(R,, RT ) Var(RT ) More intuitively, E(R,) -R,_ E(RT) -R, Cov(R,, RT) Var(RT) That is, E(R,) -R, is a constant. It is same across any asset or portfolio in the market. Cov(R,, RT) Further study: Proof the CAPM equation: Consider an arbitrary asset or portfolio in the market, whose return is denoted as R, . We build a new portfolio by combining R, and the tangency portfolio RT . The weights are (w, 1-w) for R, and R, respectively. Then the mean and variance of the new portfolio's return are E[w R, +(1-w) R,] and Var[w R,+(1 -w) R,] respectively. Hence, the slope of the line passing through the new portfolio and Rf in Mean- Standard Deviation space is Slope= E[w R,+(1-w) RT ] -R VVar[w R,+(1- w) RT]Now we try to maximize the slope (with respect to w): Max E[w R, + (1-w) RT ] - R. W V Var[w R,+(1- w) RT] The first order condition is E(R, )- E(RT )NV Var[w R, +(1-w) R. ]_1 2wVar(R,)+2(w-1)Var(RT)+2(1-2w) cov(R, , RT) (E[w R, +(1- w) R, ]-R.} 2 VVar[w R, +(1-w) RT ] Var[w R, + (1-w) RT ] =0 Note that tangency portfolio is the portfolio that maximizes the slope of all lines passing through Rf. So the new portfolio must be the tangency portfolio itself. That is, w=0. Substitute w=0 into the first order condition equation, we obtain [E(R,)-E(RT)]Var(RT) = [cov(R,, RT) - Var( RT)][E(RT )- E(R, )]. Rewrite the above equation, we get E(R. )- E(RT ) E(RT )- E(Rf) cov(R,, RT) - Var(RT) Var(RT) By applying the rule a _c _a+c d b+d , the equation E(R,)- E(R, ) E(RT)-E(R, ) is cov(R,, RT) Var(RT) obtained. Hence the CAPM equation is valid












