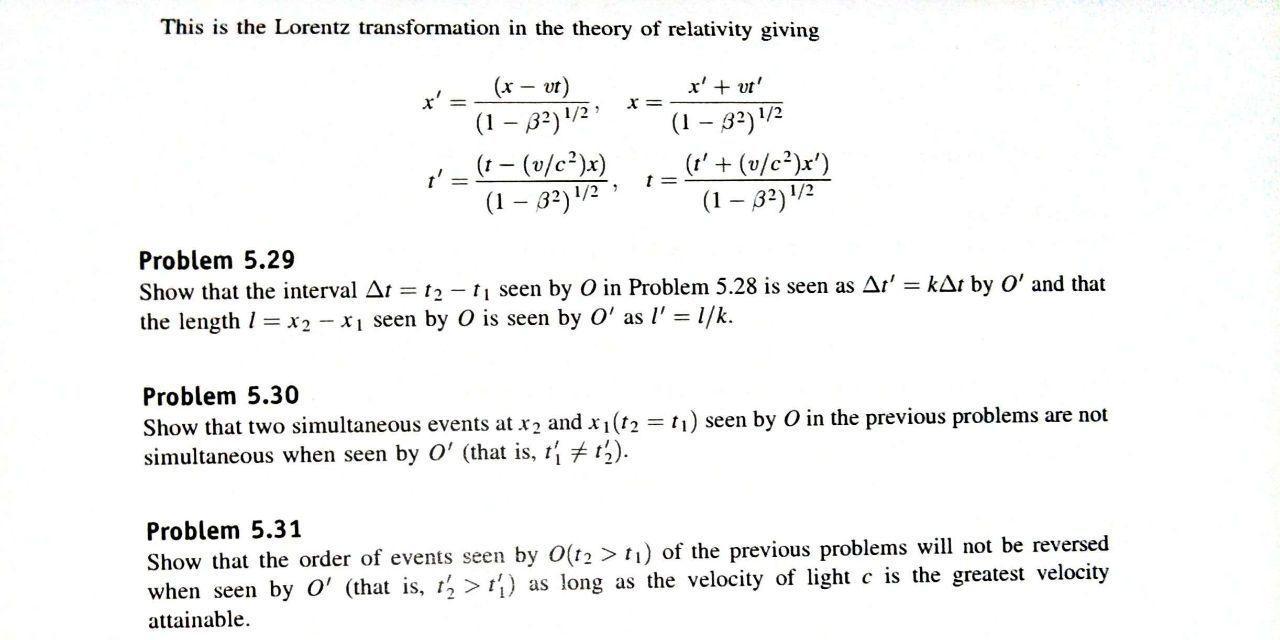
5.28 In extending the Doppler principle consider the accompanying figure where O is a stationary observer at the origin of the coordinate system O(x, t) and O' is an observer situated at the origin of the system O'(x',') which moves with a constant velocity v in the x direction relative to the system 0. When O and O' are coincident at t=t' = 0 a light source sends waves in the x direction with constant velocity c. These waves obey the relation 0=x-c(seen by O)=x2-c2(seen by O'). Since there is only one relative velocity u, the transformation x' = k(x- ut) and x = k'(x + ut') (3) must also hold. Use (2) and (3) to eliminate x' and r' from (1) and show that this identity is satisfied only by k=k' = 1/(1-32) 1/2, where 8 = v/c. (Hint-in the identity of equation (1) equate coefficients of the variables to zero.). 0 0 (xt) vt v 0' (1) 0' (x't') (2) This is the Lorentz transformation in the theory of relativity giving (x ut) (1 - 3) /' x' = (t - (v/c)x) (1 - 32) / X = x' + vt' (1-3) /2 (t' + (v/c)x') (1-3) 1/2 t= Problem 5.29 Show that the interval At = t - 1 seen by 0 in Problem 5.28 is seen as At' = kAt by O' and that the length 1 = x2 - x seen by O is seen by O' as l' = 1/k. Problem 5.30 Show that two simultaneous events at x and x(t2 = t) seen by 0 in the previous problems are not simultaneous when seen by O' (that is, th #t). Problem 5.31 Show that the order of events seen by O(t2 > t) of the previous problems will not be reversed when seen by O' (that is, t > t) as long as the velocity of light c is the greatest velocity attainable.