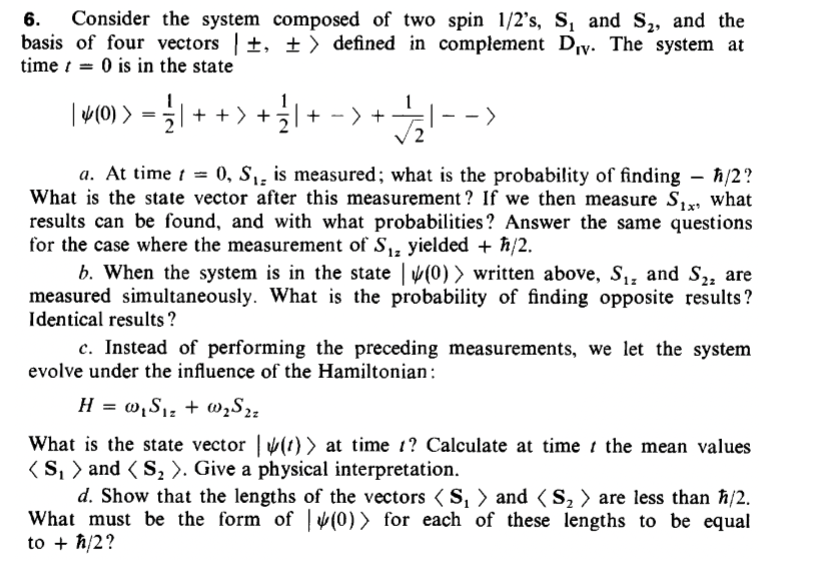
6. Consider the system composed of two spin 1/2's, S and S2, and the basis of four vectors | > defined in complement Dv. The system at time t = O is in the state |(0) > = | + + > + | + > + 2|\- a. At time 0, S is measured; what is the probability of finding - /2? What is the state vector after this measurement? If we then measure Sx, what results can be found, and with what probabilities? Answer the same questions for the case where the measurement of S yielded + /2. b. When the system is in the state | (0)> written above, S, and S2, are measured simultaneously. What is the probability of finding opposite results? Identical results? c. Instead of performing the preceding measurements, we let the system evolve under the influence of the Hamiltonian: HwSS2z = What is the state vector | (1) > at time 1? Calculate at time the mean values and . Give a physical interpretation. d. Show that the lengths of the vectors (S > and are less than /2. What must be the form of 4(0)) for each of these lengths to be equal to+h/2?