Question
6. (Hedging exotic options with vanillas). A vanilla option (also called plain vanilla) is a simple put or call (American or European style). Vanilla options
6. (Hedging exotic options with vanillas). A vanilla option (also called plain vanilla) is a simple put or call (American or European style). Vanilla options are traded in public markets (the CBOE and elsewhere). In theory, they are liquid, meaning that you can buy or sell at something like the posted price. (Many publicly traded options are not very liquid in practice, as few or no trades happen in a given day. Index options (options on major stock indices) that expire soon and are near the money (the price of the underlier) are pretty active.) An exotic option is an option with any other payout. This exercise considers a hedge of an exotic option using the underlier, cash, and a vanilla option. Suppose the underlier has price S0 today and the price tomorrow is one of the the three numbers St = uS0, or St = mS0, or St = dS0. The multipliers are u > 1 (the up move), d < 1, the down move, and m, the middle move, which may be a little up (m > 1) or a little down (m < 1). Suppose the price of the vanilla today is F0 and the price is one of the values Ft = Fu (if St = uS0), Fm (if St = mS0), or Fd (if St = dS0). The goal of this exercise is a hedging argument that leads to a formula of the form V0 = e rt (quVu + qmVm + qdVd) . (1) The risk neutral probabilities qu, qm, and qd depend on r, u, m, d, and Fu, Fm, Fd, and F0. The risk neutral probabilities should not be negative and qu + qm + qd = 1. The situation is summarized in the table time 0 t t t state u m d cash 1 e rt e rt e rt underlier S0 uS0 mS0 dS0 vanilla F0 Fu Fm Fd exotic V0 =??? Vu Vm Vd The three instruments, cash, underlier, vanilla, have values known at time t and time 0 (today). The exotic have value known at t but not today. (a) Assume that a formula of the form (1) exists. Derive three linear equations for the three unknowns qu, qm, and qd by taking the exotic to be cash, the underlier, and the vanilla option. The known prices 1 (for cash), S0 and F0 should be reproduced. 2 (b) Under what conditions do these equations have a unique solution? What do you need to know about the numbers u, m, d, Fu, Fm, and Fd. Explain how these conditions make financial sense. For example, it must be that the vanilla option payout cannot be replicated with cash and the underlier. If it could be, then the vanilla would not add anything new and would not help replicate the exotic. (c) Show that if the conditions of part (b) are satisfied, then it is possible to replicate the payout of any exotic using a portfolio of cash, the underlier, and the vanilla. The portfolio value today is 0 = + S0 + F0. The value tomorrow is t = ert + St + Ft. The portfolio weights are , , and . (The equations that determine , , and are almost the same (or exactly the same) as the equation in part (a). But the point of view is different. The portfolio weights in the CRR two state binary model were called C and .) (d) Show that if qu < 0, and the conditions of part (b) are satisfied, then there is an arbitrage. Hint: Show that there is a portfolio (of cash, underlier, and vanilla) that pays 1 if St = uS0 and zero otherwise. This portfolio would have negative cost (because qu < 0) but possibly positive payout, so it would be an arbitrage. Explain.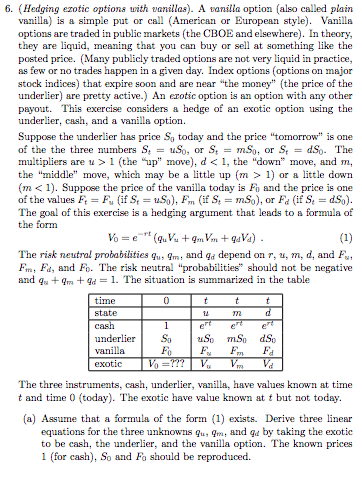
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


