(6 points) Consider the function g(:L') = cos2(12;r:) X |:r:3 + 6:3 2| >

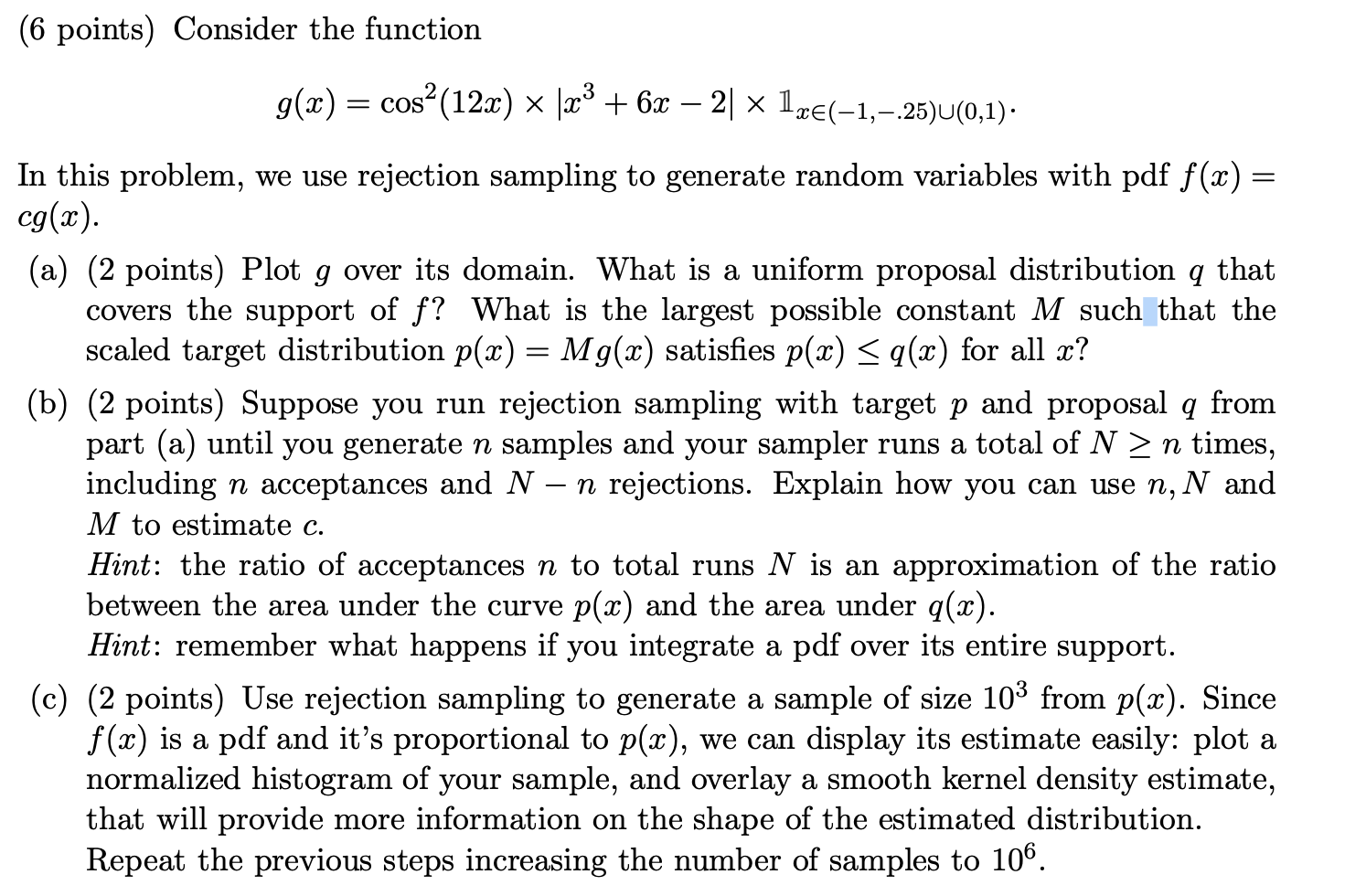
(6 points) Consider the function = COS2(12X) > < -k 6T 21 > < In this problem, we use rejection sampling to generate random variables with pdf f@) = cg@). (a) (2 points) Plot g over its domain. What is a uniform proposal distribution q that covers the support of f? What is the largest possible constant M such Ithat the scaled target distribution p@) = Mg@) satisfies p@) q@) for all x? (b) (2 points) Suppose you run rejection sampling with target p and proposal q from part (a) until you generate n samples and your sampler runs a total of N > n times, including n acceptances and N n rejections. Explain how you can use n, N and M to estimate c. Hint: the ratio of acceptances n to total runs N is an approximation of the ratio between the area under the curve p@) and the area under q@). Hint: remember what happens if you integrate a pdf over its entire support. (c) (2 points) Use rejection sampling to generate a sample of size 103 from p@). Since f@) is a pdf and it's proportional to p@), we can display its estimate easily: plot a normalized histogram of your sample, and overlay a smooth kernel density estimate, that will provide more information on the shape of the estimated distribution. Repeat the previous steps increasing the number of samples to 106.