Answered step by step
Verified Expert Solution
Question
1 Approved Answer
6. Problem 3.4.4. If Q and Q2 are orthogonal matrices, so that QTQ = I, show that Q1Q2 is also orthogonal. If Q is
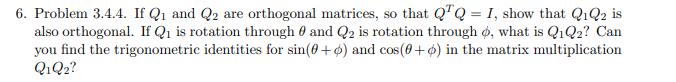
6. Problem 3.4.4. If Q and Q2 are orthogonal matrices, so that QTQ = I, show that Q1Q2 is also orthogonal. If Q is rotation through and Q2 is rotation through o, what is Q1Q2? Can you find the trigonometric identities for sin(0+) and cos(0+o) in the matrix multiplication Q1Q2?
Step by Step Solution
★★★★★
3.38 Rating (160 Votes )
There are 3 Steps involved in it
Step: 1
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Linear Algebra with Applications
Authors: Steven J. Leon
7th edition
131857851, 978-0131857858
