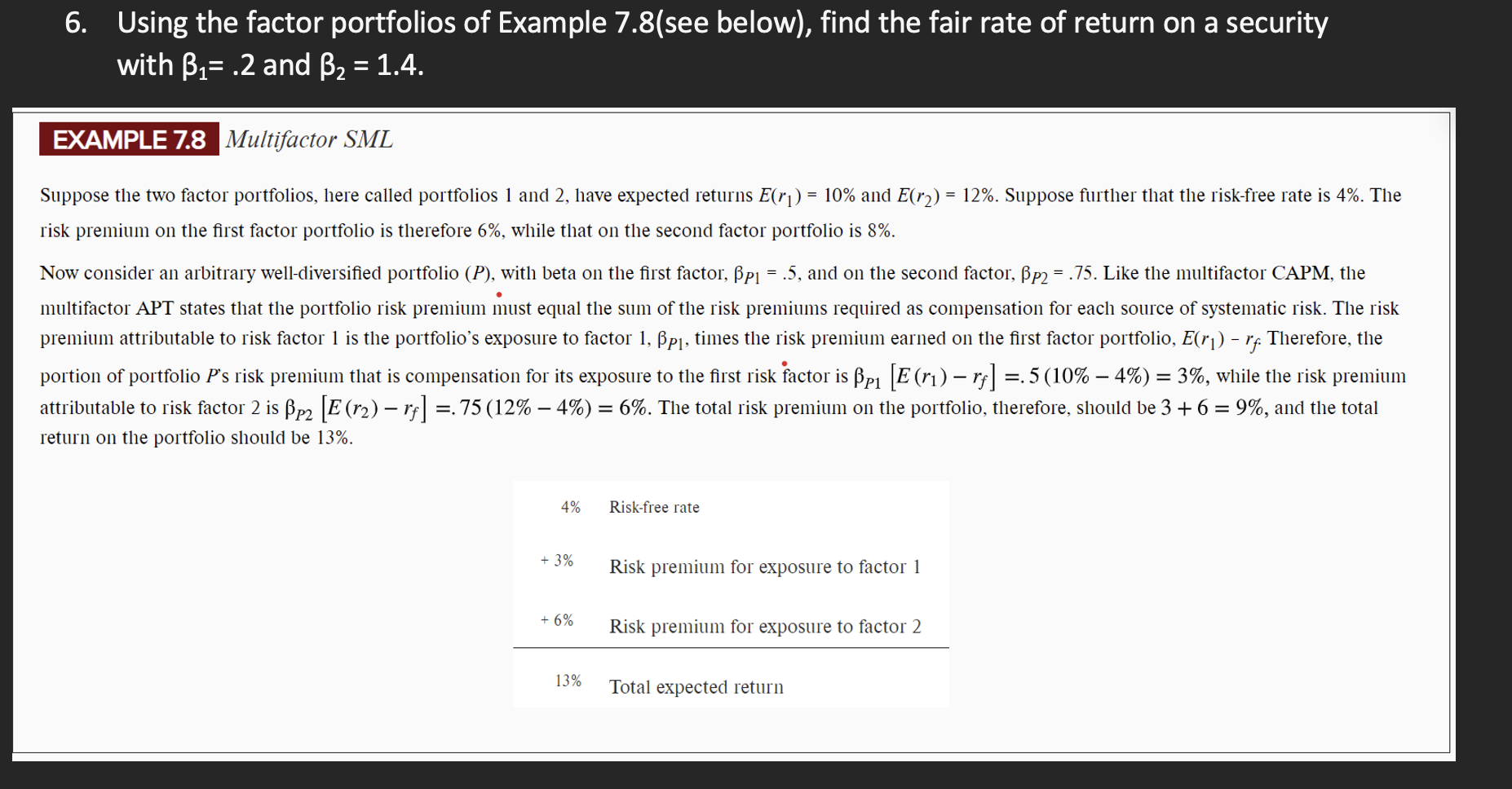
6. Using the factor portfolios of Example 7.8(see below), find the fair rate of return on a security with 1=.2 and 2=1.4. EXAMPLE 7.8 Multifactor SML Suppose the two factor portfolios, here called portfolios 1 and 2, have expected returns E(r1)=10% and E(r2)=12%. Suppose further that the risk-free rate is 4%. The risk premium on the first factor portfolio is therefore 6%, while that on the second factor portfolio is 8%. Now consider an arbitrary well-diversified portfolio (P), with beta on the first factor, P1=.5, and on the second factor, P2=.75. Like the multifactor CAPM, the multifactor APT states that the portfolio risk premium must equal the sum of the risk premiums required as compensation for each source of systematic risk. The risk premium attributable to risk factor 1 is the portfolio's exposure to factor 1,P1, times the risk premium earned on the first factor portfolio, E(r1) - rf. Therefore, the portion of portfolio P s risk premium that is compensation for its exposure to the first risk factor is P1[E(r1)rf]=.5(10%4%)=3%, while the risk premium attributable to risk factor 2 is P2[E(r2)rf]=.75(12%4%)=6%. The total risk premium on the portfolio, therefore, should be 3+6=9%, and the total return on the portfolio should be 13%. 6. Using the factor portfolios of Example 7.8(see below), find the fair rate of return on a security with 1=.2 and 2=1.4. EXAMPLE 7.8 Multifactor SML Suppose the two factor portfolios, here called portfolios 1 and 2, have expected returns E(r1)=10% and E(r2)=12%. Suppose further that the risk-free rate is 4%. The risk premium on the first factor portfolio is therefore 6%, while that on the second factor portfolio is 8%. Now consider an arbitrary well-diversified portfolio (P), with beta on the first factor, P1=.5, and on the second factor, P2=.75. Like the multifactor CAPM, the multifactor APT states that the portfolio risk premium must equal the sum of the risk premiums required as compensation for each source of systematic risk. The risk premium attributable to risk factor 1 is the portfolio's exposure to factor 1,P1, times the risk premium earned on the first factor portfolio, E(r1) - rf. Therefore, the portion of portfolio P s risk premium that is compensation for its exposure to the first risk factor is P1[E(r1)rf]=.5(10%4%)=3%, while the risk premium attributable to risk factor 2 is P2[E(r2)rf]=.75(12%4%)=6%. The total risk premium on the portfolio, therefore, should be 3+6=9%, and the total return on the portfolio should be 13%