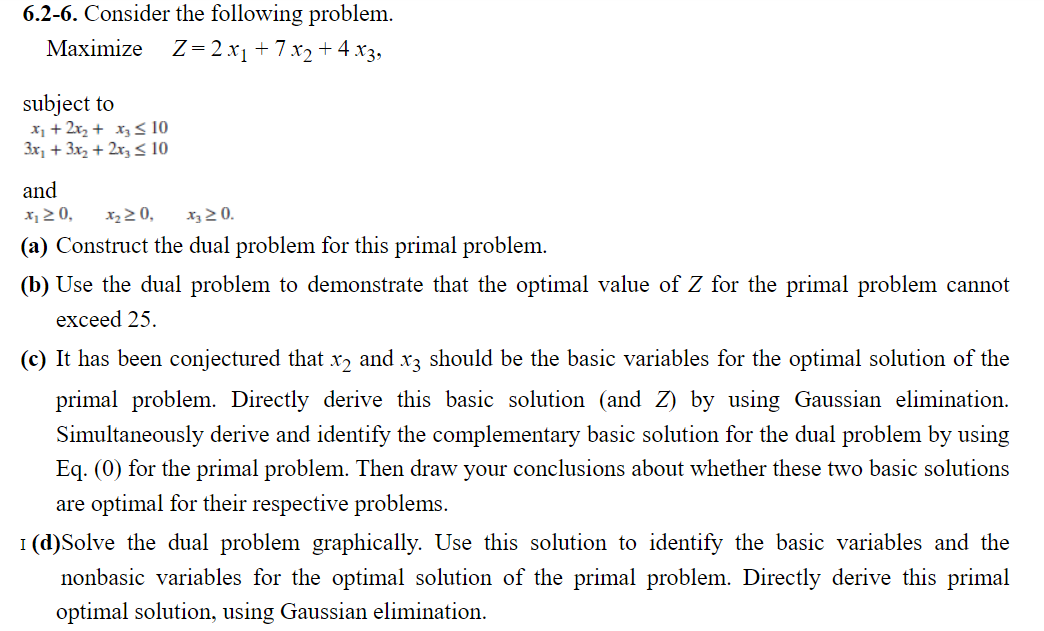
6.2-6. Consider the following problem. Maximize Z=2x +7x + 4x3, subject to x + 2x + x 10 3x + 3x + 2x 10 and x 0, x 0, * 2 0. (a) Construct the dual problem for this primal problem. (b) Use the dual problem to demonstrate that the optimal value of Z for the primal problem cannot exceed 25. (c) It has been conjectured that x and x3 should be the basic variables for the optimal solution of the primal problem. Directly derive this basic solution (and Z) by using Gaussian elimination. Simultaneously derive and identify the complementary basic solution for the dual problem by using Eq. (0) for the primal problem. Then draw your conclusions about whether these two basic solutions are optimal for their respective problems. 1 (d)Solve the dual problem graphically. Use this solution to identify the basic variables and the nonbasic variables for the optimal solution of the primal problem. Directly derive this primal optimal solution, using Gaussian elimination.