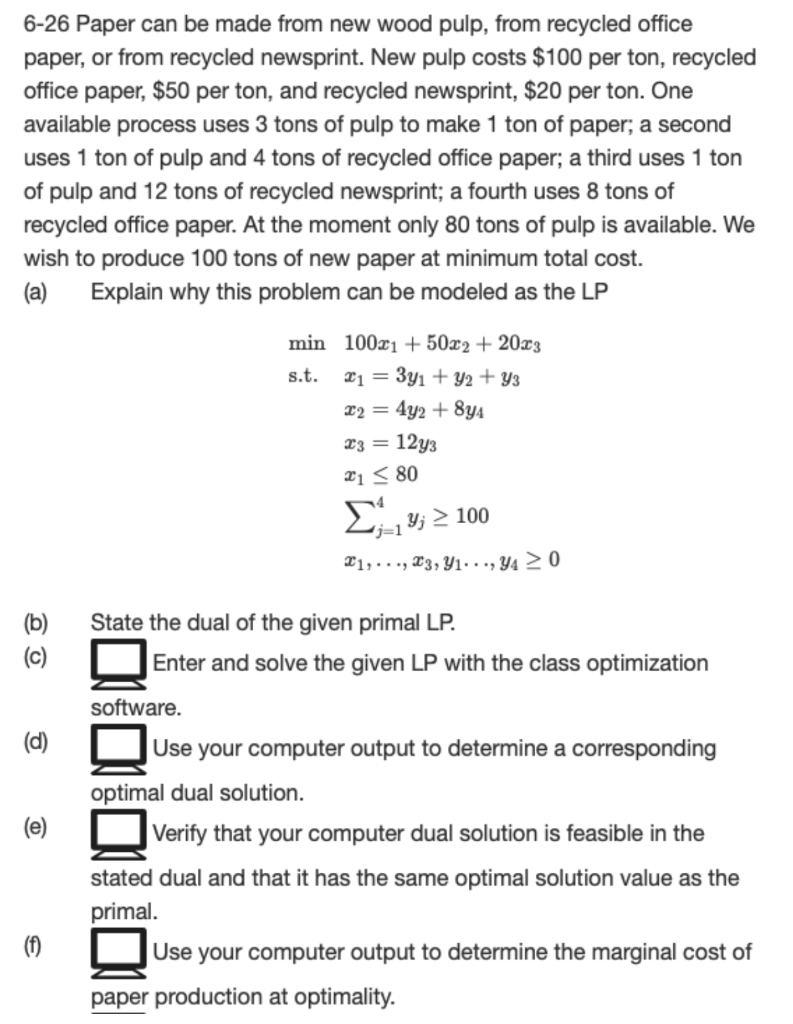

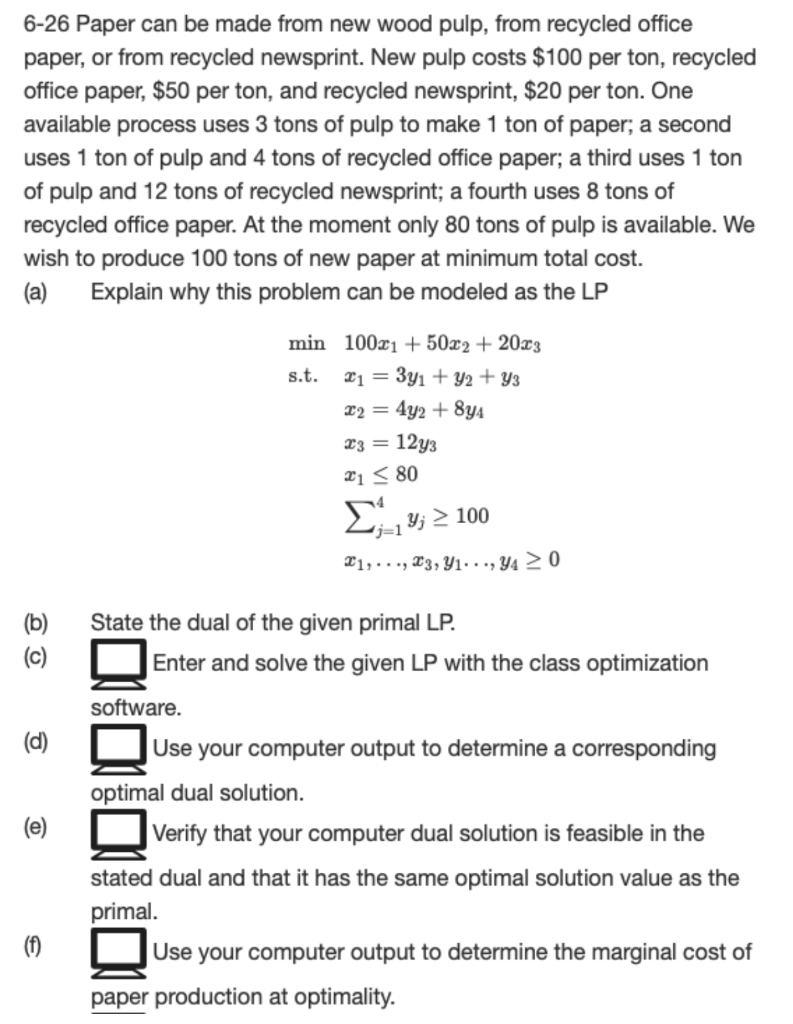
6-26 Paper can be made from new wood pulp, from recycled office paper, or from recycled newsprint. New pulp costs $100 per ton, recycled office paper, $50 per ton, and recycled newsprint, $20 per ton. One available process uses 3 tons of pulp to make 1 ton of paper, a second uses 1 ton of pulp and 4 tons of recycled office paper, a third uses 1 ton of pulp and 12 tons of recycled newsprint; a fourth uses 8 tons of recycled office paper. At the moment only 80 tons of pulp is available. We wish to produce 100 tons of new paper at minimum total cost. (a) Explain why this problem can be modeled as the LP min 100x1 + 50x2 + 2023 s.t. 21 = 3y1 + y2 + Y3 22 = 4y2 + 8y4 23 = 12y3 21 0 (b) (c) (d) State the dual of the given primal LP. Enter and solve the given LP with the class optimization software. Use your computer output to determine a corresponding optimal dual solution. Verify that your computer dual solution is feasible in the stated dual and that it has the same optimal solution value as the primal. Use your computer output to determine the marginal cost of paper production at optimality. (e) (1) (h) (0) Use your computer output to determine how much we should be willing to pay to obtain an additional ton of pulp. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of pulp increased to $150 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of recycled office paper decreased to $20 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of recycled office paper increased to $75 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the number of tons of new paper needed decreased to 60. 0) (k) 6-26 Paper can be made from new wood pulp, from recycled office paper, or from recycled newsprint. New pulp costs $100 per ton, recycled office paper, $50 per ton, and recycled newsprint, $20 per ton. One available process uses 3 tons of pulp to make 1 ton of paper, a second uses 1 ton of pulp and 4 tons of recycled office paper, a third uses 1 ton of pulp and 12 tons of recycled newsprint; a fourth uses 8 tons of recycled office paper. At the moment only 80 tons of pulp is available. We wish to produce 100 tons of new paper at minimum total cost. (a) Explain why this problem can be modeled as the LP min 100x1 + 50x2 + 2023 s.t. 21 = 3y1 + y2 + Y3 22 = 4y2 + 8y4 23 = 12y3 21 0 (b) (c) (d) State the dual of the given primal LP. Enter and solve the given LP with the class optimization software. Use your computer output to determine a corresponding optimal dual solution. Verify that your computer dual solution is feasible in the stated dual and that it has the same optimal solution value as the primal. Use your computer output to determine the marginal cost of paper production at optimality. (e) (1) 6-26 Paper can be made from new wood pulp, from recycled office paper, or from recycled newsprint. New pulp costs $100 per ton, recycled office paper, $50 per ton, and recycled newsprint, $20 per ton. One available process uses 3 tons of pulp to make 1 ton of paper, a second uses 1 ton of pulp and 4 tons of recycled office paper, a third uses 1 ton of pulp and 12 tons of recycled newsprint; a fourth uses 8 tons of recycled office paper. At the moment only 80 tons of pulp is available. We wish to produce 100 tons of new paper at minimum total cost. (a) Explain why this problem can be modeled as the LP min 100x1 + 50x2 + 2023 s.t. 21 = 3y1 + y2 + Y3 22 = 4y2 + 8y4 23 = 12y3 21 0 (b) (c) (d) State the dual of the given primal LP. Enter and solve the given LP with the class optimization software. Use your computer output to determine a corresponding optimal dual solution. Verify that your computer dual solution is feasible in the stated dual and that it has the same optimal solution value as the primal. Use your computer output to determine the marginal cost of paper production at optimality. (e) (1) (h) (0) Use your computer output to determine how much we should be willing to pay to obtain an additional ton of pulp. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of pulp increased to $150 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of recycled office paper decreased to $20 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the price of recycled office paper increased to $75 per ton. Use your computer output to determine or bound as well as possible how much optimal cost would change if the number of tons of new paper needed decreased to 60. 0) (k) 6-26 Paper can be made from new wood pulp, from recycled office paper, or from recycled newsprint. New pulp costs $100 per ton, recycled office paper, $50 per ton, and recycled newsprint, $20 per ton. One available process uses 3 tons of pulp to make 1 ton of paper, a second uses 1 ton of pulp and 4 tons of recycled office paper, a third uses 1 ton of pulp and 12 tons of recycled newsprint; a fourth uses 8 tons of recycled office paper. At the moment only 80 tons of pulp is available. We wish to produce 100 tons of new paper at minimum total cost. (a) Explain why this problem can be modeled as the LP min 100x1 + 50x2 + 2023 s.t. 21 = 3y1 + y2 + Y3 22 = 4y2 + 8y4 23 = 12y3 21 0 (b) (c) (d) State the dual of the given primal LP. Enter and solve the given LP with the class optimization software. Use your computer output to determine a corresponding optimal dual solution. Verify that your computer dual solution is feasible in the stated dual and that it has the same optimal solution value as the primal. Use your computer output to determine the marginal cost of paper production at optimality. (e) (1)









