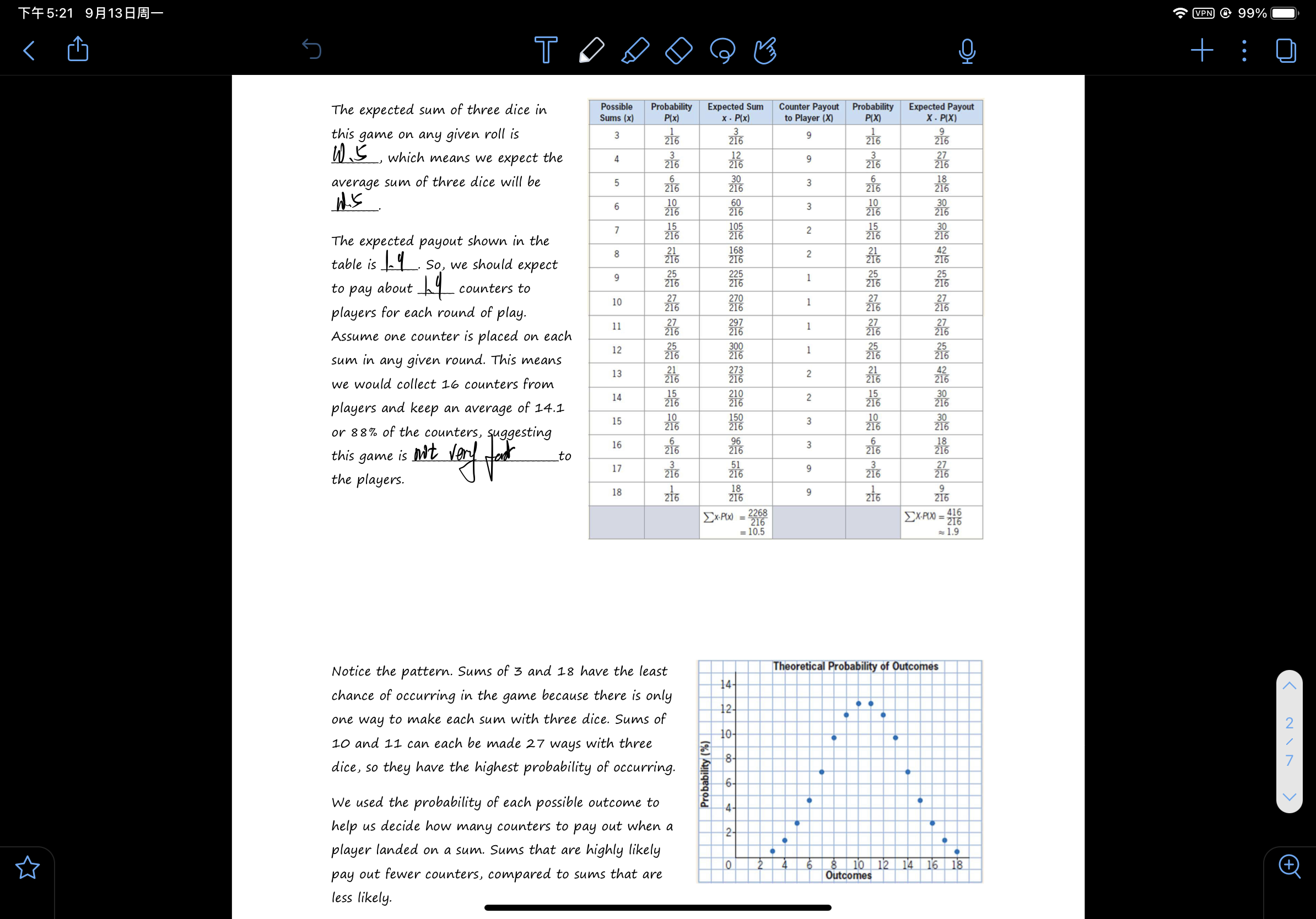
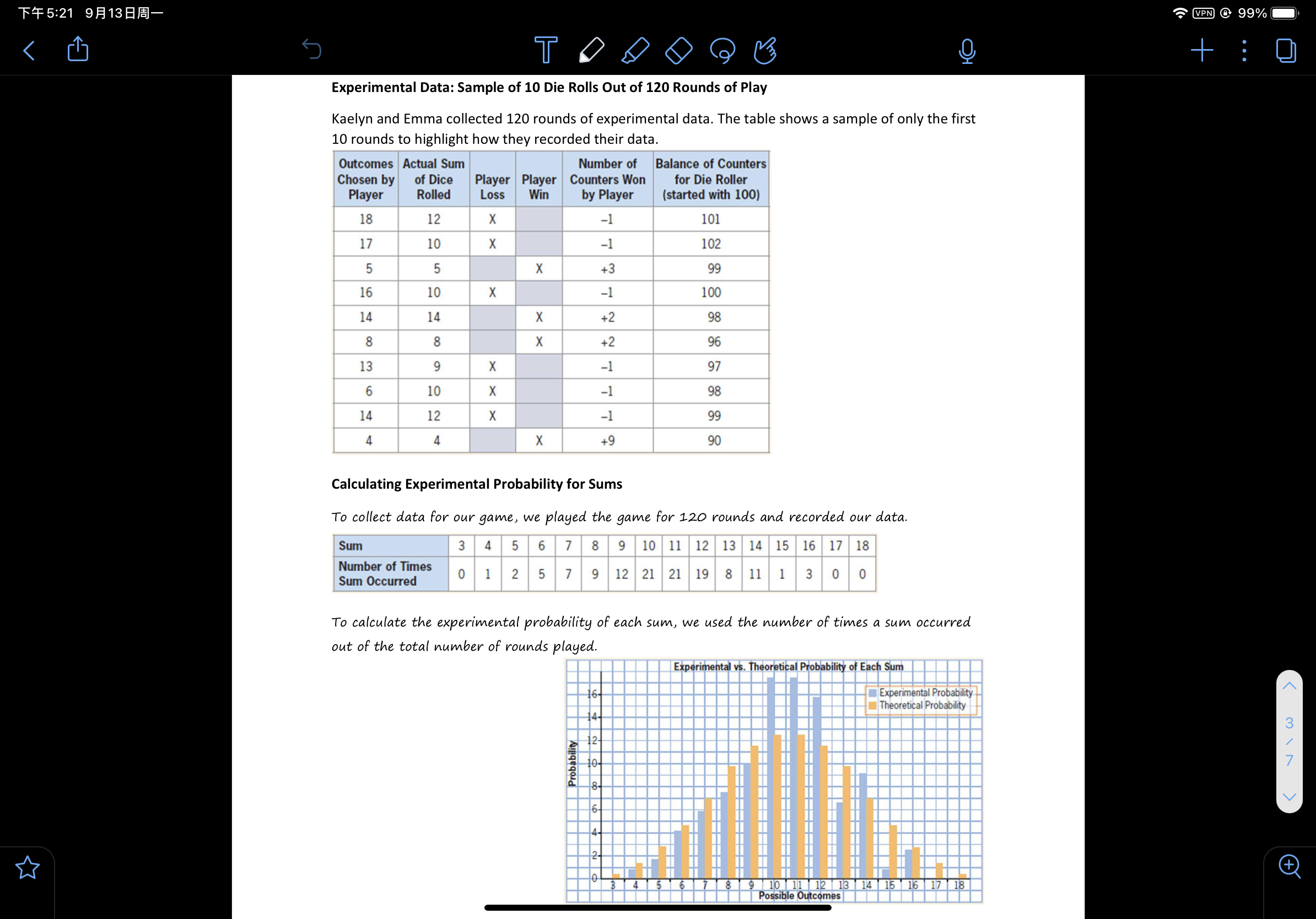
7 45:21 97138 8- UPN @ 100% K TO 9.2 Analysing a Culminating Probability Project Investigate: Analysing a Culminating Probability Project Kaelyn and Emma presented the following information about Triple Your Chances, Double Your Counters in a culminating project report. See section 9.1 for the game instructions. Read Kaelyn and Emma's report. Then, complete the questions to critique and analyse it. Consider how you might analyse your game of chance and what elements you would include in your report. Record your ideas. Triple Your Chances, Double Your Counters: Student Analysis of the Game Theoretical Distribution of the Sum of Three Dice There are 216 possible sums when three dice are rolled at once. 1 2 3 5 6 1 2 3 4 5 6 1 2 3 4 5 6 1 2 3 4 5 6 1 2 3 4 5 6 1 2 3 4 5 6 1 2 3 4 5 6 1 3 4 5 6 7 8 4 5 6 7 8 9 5 6 7 8 9 10 6 7 8 9 10 11 7 8 9 10 11 12 8 9 10|11 12 13 2 4 5 6 7 8 9 5 6 7 8 9 10 6 7 8 9 10 11 7 8 9 10 11 12 8 9 10 11 12|13 9 10|11 12|13 14 3 5 6 7 8 9 10 6 7 8 9 10 11 7 8 9 10|11 12 8 9 10|11 12|13 9 10|11 12|13|14|10|11 12|13|14 15 - 6 7 8 9 10 11 7 8 9 10 11 12 8 9 10 11 12 13 9 10 11 12 13 14 10|11 12|13|14 15|11|12|13|14 15|16 V 5 7 8 9 10 11 12 8 9 10|11 12 13 9 10|11 12|13|14|10|11 12|13|14|15 11|12|13|14|15|16|12|13|14|15|16|17 V 6 8 9 10 11 12 13 9 10|11 12 13 14|10|11 12 13 14 15|11|12|13|14 15|16|12|13|14 15|16|17|13|14|15|16|17|18 W'FF 5:21 9l3l13Ell* The expected sum of three dice in this game on any given roll is \"45; which means we ex ect the p average sum of three dice will be JJL. The expected payout shown in the table is l-\" . So, we should expect to pay about counters to players For each round of play. Assume one counter is placed on each sum in any given round. This means we would collect lb counters From players and keep an average of 14.1 or 88% of the counters, uggesting this game is the players. Notice the pattern. Sums of 5 and 18 have the least chance of occurring in the game because there is only one way to make each sum with three dice Sums of 10 and 11 can each be made 27 ways with three dice, so they have the highest probability of occurring. We used the probability of each possible outcome to help us decide how many counters to pay out when a player landed on a sum. Sums that are highly likely pay out Fewer counters, compared to sums that are less likely. 7 +5:21 9A13BA- VPN @ 99% K TODD + Experimental Data: Sample of 10 Die Rolls Out of 120 Rounds of Play Kaelyn and Emma collected 120 rounds of experimental data. The table shows a sample of only the first 10 rounds to highlight how they recorded their data Outcomes Actual Sum Number of Balance of Counters Chosen by of Dice Player Player Counters Won for Die Roller Player Rolled Loss Win by Player (started with 100) 18 12 -1 101 17 10 X -1 102 5 5 X +3 99 16 10 X -1 100 14 14 X +2 98 8 8 X +2 96 13 9 X -1 97 6 10 X -1 98 14 12 X -1 99 A X +9 90 Calculating Experimental Probability for Sums To collect data for our game, we played the game for 120 rounds and recorded our data. Sum 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 Number of Times Sum Occurred 0 1 2 5 7 9 12 21 21 19 8 11 1 3 0 0 To calculate the experimental probability of each sum, we used the number of times a sum occurred out of the total number of rounds played. Experimental vs. Theoretical Probability of Each Sum Experimental Probability Theoretical Probability Probability 12 13 14 15 16 17 18 Possible OutcomesT 45:22 9A13BA- VPN @ 99% K TOO + Calculating Counter Payout Based on Experimental Probability from 120 Rounds Sum 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 Number of Times Sum Occurred 0 1 2 5 7 9 12 21 21 19 8 11 1 3 0 0 Number of Matches With Player 0 1 1 2 5 6 0 0 0 0 6 7 0 2 0 0 Payout Factor 9 9 3 3 2 2 1 1 1 1 2 2 3 3 9 9 Total Payout 9 3 6 10 12 12 14 6 After playing 120 rounds, we had to pay out a total of 72 counters. This means we paid out 0-b liza) counters for every round played in our game. Conclusions The Experimental vs. Theoretical Probability of Each Sum graph shows which sums occurred more often than expected in our game. Sums of 9, 10, 11, and 12 occurred most often. Since these sums can be made in many ways with three dice, this is not a surprise. However, we would have expected sums 8, 13, and 15 to occur more often based on their theoretical probability. In the experimental distribution, sums of 3, 17, and 18 show a probability of zero, which is also not a surprise because each of these sums also has the lowest theoretical probability. The theoretical probability suggests many of the outcomes should have occurred more often, even if only a little more often We would expect the theoretical and experimental probabilities to become more similar with more rounds of play. In reality, the game was not very fair, as we had a significant advantage for winning counters. To make the game more fair we could alter the payout structure to provide greater payouts or allow players to put counters on more than one sum. For example, provide a grouping of {3, 4, 17, 18} with 9:1 payout ratio. If a player placed a counter on the (3, 4, 17, 18} group, the payout would be counters if One of those sums were rolled 2 Possible Frequency Total Probably Sums [Counter Probability Outcomes Expected Sum Payout POO POO Expected Payout to Player VID 3 7 Q007 0021 1000 Q.012 41 1000 Q041 49 1000 Q040