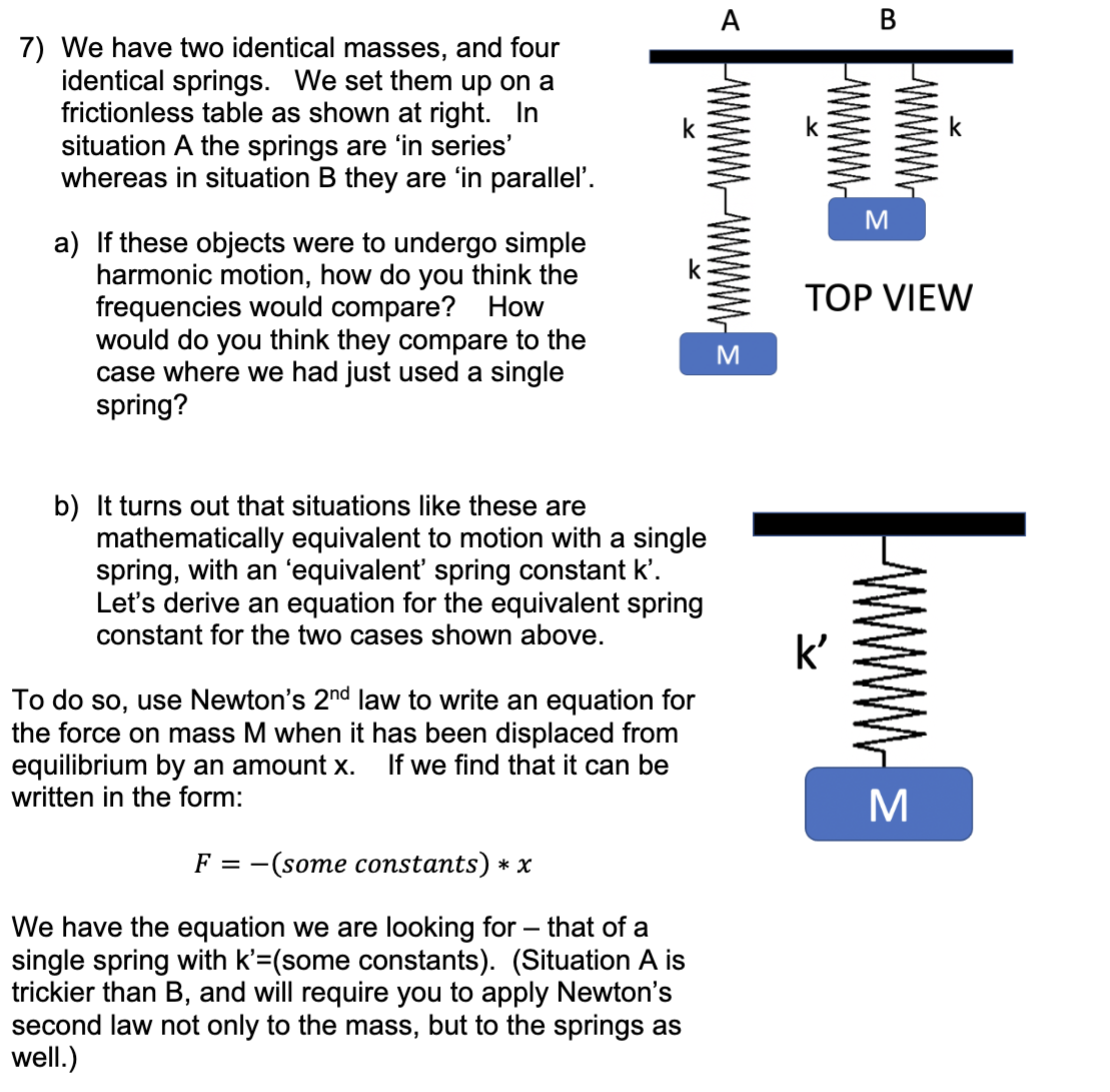
7) We have two identical masses, and four identical springs. We set them up on a frictionless table as shown at right. In situation A the springs are 'in series' whereas in situation B they are 'in parallel'. a) If these objects were to undergo simple harmonic motion, how do you think the frequencies would compare? How would do you think they compare to the case where we had just used a single spring? b) It turns out that situations like these are mathematically equivalent to motion with a single spring, with an 'equivalent' spring constant k'. Let's derive an equation for the equivalent spring constant for the two cases shown above. To do so, use Newton's 2nd law to write an equation for the force on mass M when it has been displaced from equilibrium by an amount x. If we find that it can be written in the form: F = -(some constants) * x - We have the equation we are looking for that of a single spring with k'=(some constants). (Situation A is trickier than B, and will require you to apply Newton's second law not only to the mass, but to the springs as well.) A M M TOP VIEW k' www. M