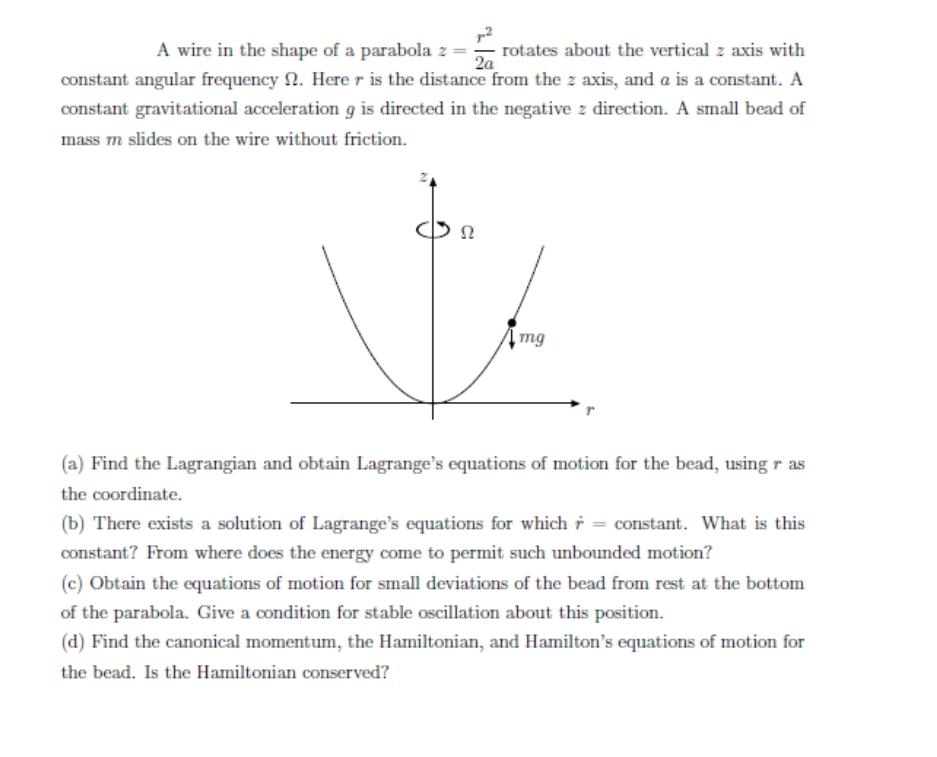
7.2 A wire in the shape of a parabola z = rotates about the vertical z axis with 2a constant angular frequency . Here r is the distance from the z axis, and a is a constant. A constant gravitational acceleration g is directed in the negative z direction. A small bead of mass m slides on the wire without friction. 52 mg (a) Find the Lagrangian and obtain Lagrange's equations of motion for the bead, using r as the coordinate. (b) There exists a solution of Lagrange's equations for which r = constant. What is this constant? From where does the energy come to permit such unbounded motion? (c) Obtain the equations of motion for small deviations of the bead from rest at the bottom of the parabola. Give a condition for stable oscillation about this position. (d) Find the canonical momentum, the Hamiltonian, and Hamilton's equations of motion for the bead. Is the Hamiltonian conserved?