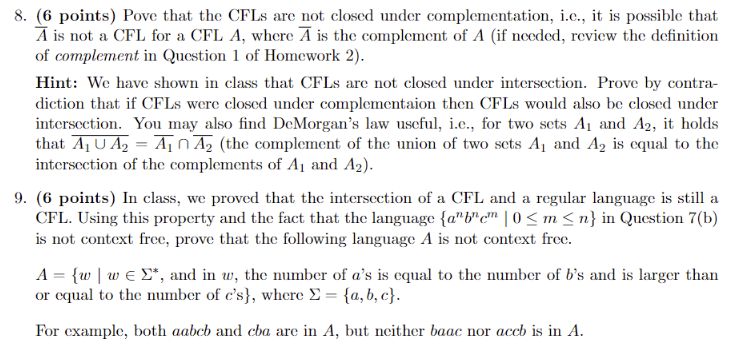
8. (6 points) Pove that the CFLs are not closed under complementation, i.c., it is possible that A is not a CFL for a CFL A, where A is the complement of A (if needed, review the definition of complement in Question 1 of Homework 2). Hint: We have shown in class that CFLs are not closed under intersection. Prove by contra- diction that if CFLs were closed under complementaion then CFLs would also be closed under intersection. You may also find DeMorgan's law useful, i.e., for two sets A and A2, it holds that A U A = A A (the complement of the union of two sets A and A is equal to the intersection of the complements of A and A). 9. (6 points) In class, we proved that the intersection of a CFL and a regular language is still a CFL. Using this property and the fact that the language {abcm |0 mn} in Question 7(b) is not context free, prove that the following language A is not context free. A = {w | w *, and in w, the number of a's is equal to the number of b's and is larger than or equal to the number of c's}, where = {a, b, c}. For example, both aabcb and cba are in A, but neither baac nor accb is in A.