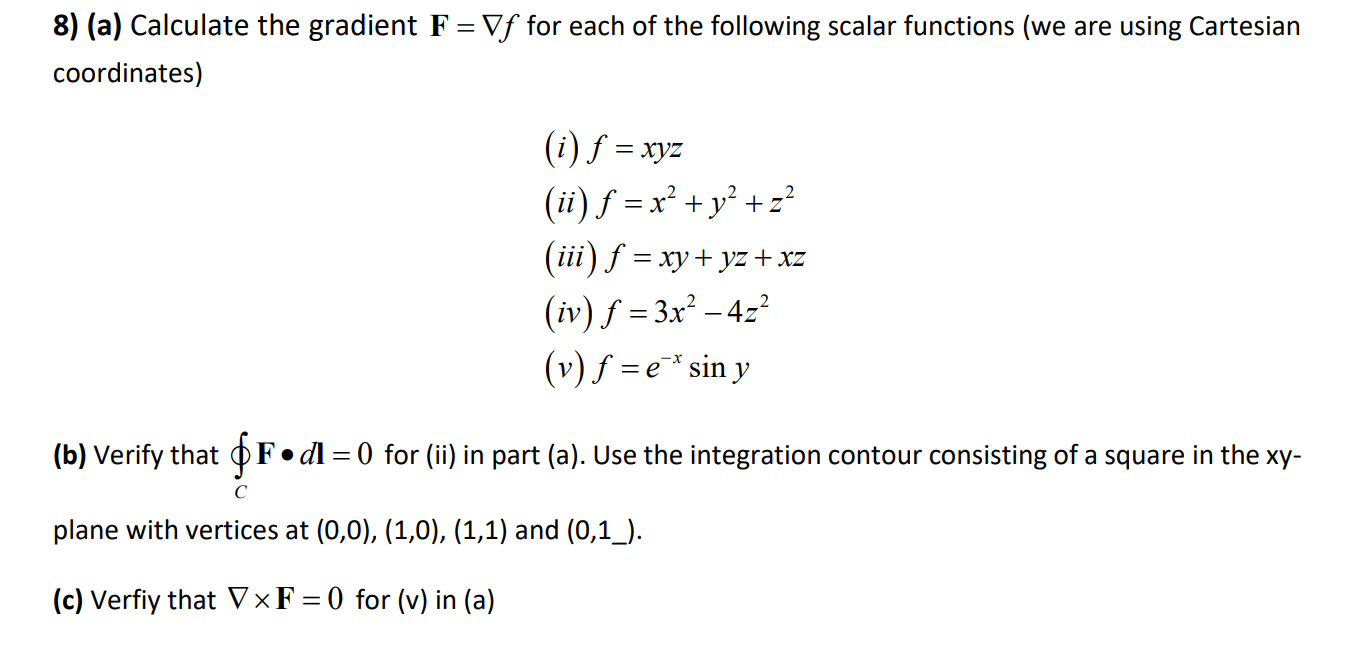
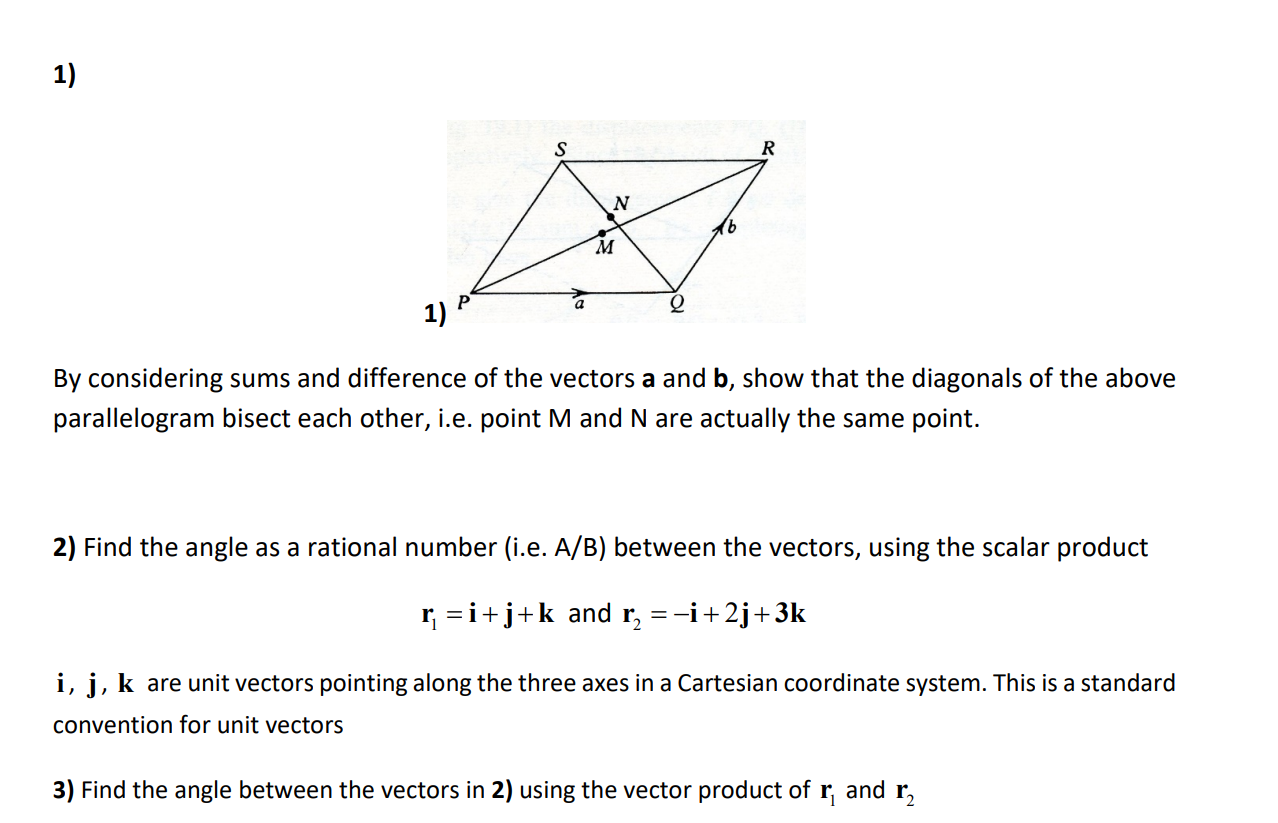
8) (a) Calculate the gradient F = V for each of the following scalar functions (we are using Cartesian coordinates) (i) f = xyz (ii) f = x + y+z (iii) f = xy + yz +xz (iv) f = 3x-4z (v) f = ex sin y (b) Verify that Fdl=0 for (ii) in part (a). Use the integration contour consisting of a square in the xy- C plane with vertices at (0,0), (1,0), (1,1) and (0,1_). (c) Verfiy that VF = 0 for (v) in (a) (9) (a) Calculate the divergence F = V f for the following vector functions. I am using the i, j, k convention for the Cartesian unit vectors (a) ix + jy+kz (b) iyz+jxz+kxy (c) ie+je+ke (d) i-3j+kz (e) (ixy+jx)/(x + y) (x, y) = 0 (f) kx + y (g) ix+jy+kz (h) (-iy+jx)/(- (x, y) 0 (b) Calculate the surface integral (Fda for the function in (a) above. Do it over the surface of a cube of sides who center is at (x, y, z) and whose faces are parallel to the Cartesian coordinate planes. (c) Divide the result in (b) by the volume of the cube and compare with the divergence in (a) above. So? (10)(a) Calculate the curl F = Vf, for the following vector functions (a) iz+jx -ky (b) 3ixz-kx (c) ie+je+ke* (d) iyz+jxz+kxy (e) iyz+jxz (f) ix+jy+k(x + y) (g) ix+jy+kyz (h) (ix+jy+kz)/(x + y + z) (x, y, z) #0 (b) Calculate the line integral of dl for the vector function in (a) above. Use a square path of side s centered at (x, y, 0) lying in the xy-plane and oriented so that each side is parallel to the x-axis, or y-axis. Compare your result with the z-component of the curl found in (a) above. So? 1) 1) N R M By considering sums and difference of the vectors a and b, show that the diagonals of the above parallelogram bisect each other, i.e. point M and N are actually the same point. 2) Find the angle as a rational number (i.e. A/B) between the vectors, using the scalar product r = i+j+k and r i+2j+3k i, j, k are unit vectors pointing along the three axes in a Cartesian coordinate system. This is a standard convention for unit vectors 3) Find the angle between the vectors in 2) using the vector product of r and r 7) 7.19 The vectors a, b and c are not coplanar. Verify that the expressions a' = bx c [ a, b, c ]' b' = c' = [ a, b, c ]' axb [ a, b, c ] define a set of reciprocal vectors a', b' and c' with the following properties: (a) a' a b' b = c' . c = 1; a etc = 0; (b) a' ba' c = b' (c) [a', b', c'] = 1/[a, b, c]; (d) a = (b' c')/[a', b', c'].