81 -X Dx. EXAMPLE 1 Evaluate X2 SOLUTION Let X = 9 Sin(?), Where-T/2.5 ?$1/2. Then Dx=19 Cos(? D? And V/ 81-X2-V 81-81 Sin2(8) = V/ 81 Cos2(9) = 9|Cos(?)-9 Cos(9) (Note That Cos(0) 0 Because -?/2 ? ?/2.) Thus The Inverse Substitution Rule Gives 9 Cos(0) Sin Cos2 ( ? Since This Is An Indefinite Integral, We Must Return To The Original Variable X. This Can Be

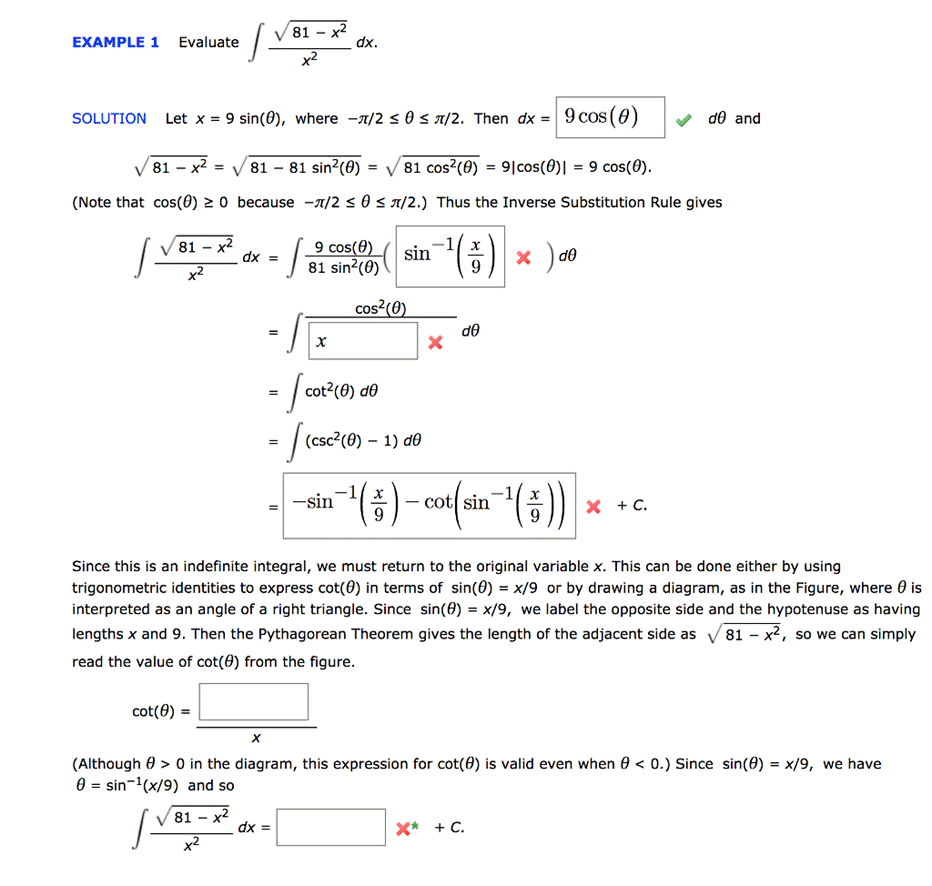0 in the diagram, this expression for cot(9) is valid even when ?

 

EXAMPLE 1 Evaluate 81x2 dx. x SOLUTION Let x = 9 sin(0), where -/2 0/2. Then dx = 9 cos(0) de and 81-x= 81 - 81 sin(0) = 81 cos (0) = 9| cos(0)| = 9 cos(0). (Note that cos(0) 0 because -/2 /2.) Thus the Inverse Substitution Rule gives 81x2 x dx = 9 cos(0) 81 sin (0) Sin -2() ) de = x cos (0) - Scot d cot(0) de / (CSC (0) (csc(0) - 1) de (+)-cot(sin()) -sin 9 * + C. Since this is an indefinite integral, we must return to the original variable x. This can be done either by using trigonometric identities to express cot(0) in terms of sin(0) = x/9 or by drawing a diagram, as in the Figure, where is interpreted as an angle of a right triangle. Since sin(0) = x/9, we label the opposite side and the hypotenuse as having lengths x and 9. Then the Pythagorean Theorem gives the length of the adjacent side as 81 - x, so we can simply read the value of cot(0) from the figure. cot(0) (Although > 0 in the diagram, this expression for cot(e) is valid even when < 0.) Since sin(0) = x/9, we have sin(x/9) and so 81 x2 x - dx = ** + C.