
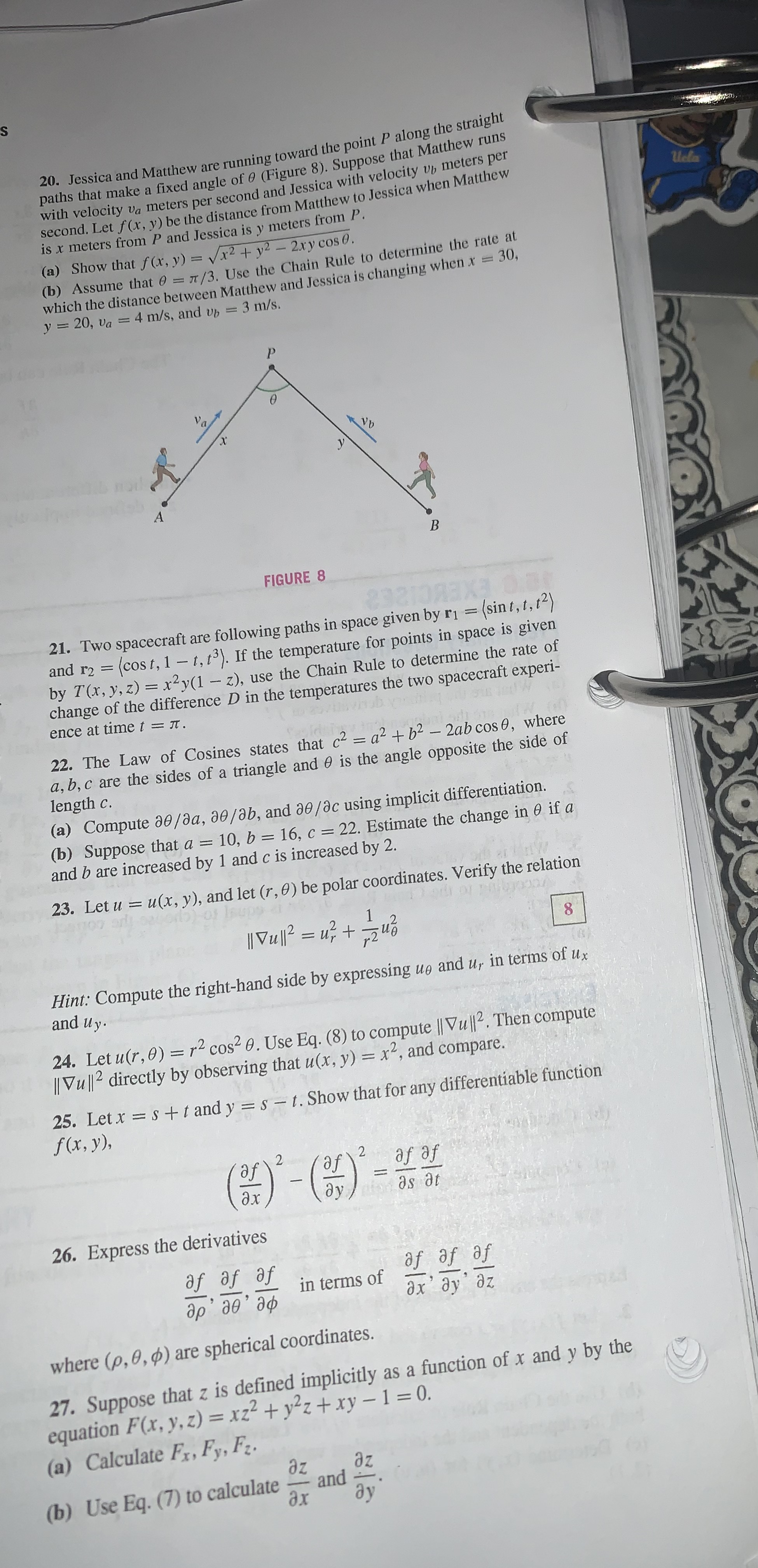


854 CHAPTER 15 DIFFERENTIATION IN SEVERAL VARIABLES 9. 20. Jessica and I at 2 oh ; h (x , y ) = -, x = 1/2, y = 1712 paths that make a with velocity va 10. "J : f ( x , y , Z ) = xy - 22, x = rcos0, y = cos2 0, z =r second. Let f (x, is x meters from In Exercises 11-16, use the Chain Rule to evaluate the partial derivative (a) Show that at the point specified. (b) Assume th 11. af/au and af/av at (u, v) = (-1, -1), where f (x, y, z) = x3 + yz2, which the distar *=u +v,y=u+ 02, z=uv y = 20, Va = 12. af/as at (r, s) = (1,0), where f(x, y) = In(xy), x = 3r + 2s, y = 5r + 3s 13. ag/20 at (r, 0) = (2V/2, #), where 8 (x, y ) = 1/ (x+ yz ) , x = r cos 0, y = r sine 14. dg/ds at s = 4, where g(x, y) = x2 - y2, x = $2 + 1, y = 1 -2s 15. ag/du at (u, v) = (0, 1), where g(x, y) = x2 - y2, x = e" cos v, y = ell sin v 16. ah at (q, r) = (3, 2), where h(u, v) = ue, u = q3, v = qr2 aq 17. Given f(x, y) and y = y(x), we can define a composite function 8 (x) = f(x, y(x)). (a) Show that g'(x) = of of y' (x ). 21. Two ax ay and r2 (b) Let f(x, y) = x3 - xy2 and y(x) = 1 - x. With g(x) = f(x, y(x)), by T(x, use the formula in (a) to determine g'(x), expressing the result in terms of change x only. ence at (c) With f(x, y) and y(x) as in (b), give an expression for g(x) in terms of x. Then compute g'(x) from g(x), and show that the result coincides 22. Th with the one from (b). a, b, c length 18. Let f(x, y) =4 - x2y2 + e2x and y(x) = . Define g(x) = f(x, y(x)). (a) C (b) s (a) Use the derivative formula from Exercise 17(a) to prove that g'(x) = 0 and b and therefore that g is a constant function. (b) Express g(x) directly in terms of x, and simplify to show that g is 23. indeed a constant function. 19. A baseball player hits the ball and then runs down the first base line at 20 ft/s. The first baseman fields the ball and then runs toward first base Hi along the second base line at 18 ft/s as in Figure 7. an 24 FIGURE 7 Determine how fast the distance between the two players is changing at a moment when the hitter is 8 ft from first base and the first baseman is 6 fi from first base.20. Jessica and Matthew are running toward the point P along the straight paths that make a fixed angle of @ (Figure 8). Suppose that Matthew runs with velocity va meters per second and Jessica with velocity up meters per second. Let f(x, y) be the distance from Matthew to Jessica when Matthew Uela is x meters from P and Jessica is y meters from P. (a) Show that f(x, y) = vx2 + y2 - 2xy cose. b) Assume that 0 = 7/3. Use the Chain Rule to determine the rate at which the distance between Matthew and Jessica is changing when x = 30, y = 20, va = 4 m/s, and vb = 3 m/s. Va y R FIGURE 8 21. Two spacecraft are following paths in space given by r1 = (sint, t, (2) and r2 = (cost, 1 - t, 13). If the temperature for points in space is given by T(x, y, z) = x2y(1 - z), use the Chain Rule to determine the rate of change of the difference D in the temperatures the two spacecraft experi- ence at time t = It. 22. The Law of Cosines states that c2 = a2 + b2 - 2ab cos0, where a, b, c are the sides of a triangle and 0 is the angle opposite the side of length C. (a) Compute 20/da, 30/ab, and 30 /ac using implicit differentiation. (b) Suppose that a = 10, b = 16, c = 22. Estimate the change in 0 if a and b are increased by 1 and c is increased by 2. 23. Let u = u(x, y), and let (r, 0) be polar coordinates. Verify the relation 11 Dull? = 17+ 7240 8 Hint: Compute the right-hand side by expressing up and ur in terms of ux and uy. 24. Let u(r, 0) = r2 cos2 0. Use Eq. (8) to compute || Vul|2. Then compute |/ Vul/2 directly by observing that u(x, y) = x2, and compare. 25. Let x = s + t and y = s - t. Show that for any differentiable function f (x, y), of of as at 26. Express the derivatives af af of of of af in terms of ap' 20' ad ax' ay' az where (p, 0, ) are spherical coordinates. 27. Suppose that z is defined implicitly as a function of x and y by the equation F(x, y, z) = xz2 + y2z + xy - 1=0. (a) Calculate Fx, Fy, Fz. az az (b) Use Eq. (7) to calculate and ax aySEC 28. Calculate az/ax and az/By at the points (3. 2, 1) and (3.2, -1). where z is defined implicitly by the equation 24 + z2 x2 - y - 8 =0. entiation. In Exercises 29-34, calculate the partial derivative using implicit differ- 29. az ax ' x2 y + y2z +x22 =10 30. aw az x2w + w3 + wz2+3yz=0 31. az av' exy + sin(xz) +y =0 32. ar at and at FIG ar ' 12 = teslr 33. aw 40. For a ay W2 + x2 + w 2 + 2 = 1 at (x, y, w) = (1, 1, 1) y' + 4xy (a) Show 34. au /aT and aT /aU, (TU - V)2 In(W - UV) = In2 at (b) Let (T, U, V, W) = (1, 1, 2, 4) 35. Let r = (x, y, z) and er = r/Irl|. Show that if a function f (x, y, z) = F(r) depends' Use the ends only on the distance from the origin r = Irll = (1, 2) s Vx2 + y2 + 22, then 41. T with n endT .naomi e lo es of = F'(r)er 9 eA niamob navig 36. Let f(x, y, z) = e-x2-12-22 = e-12 , with r as in Exercise 35. Com- pute Vf directly and using Eq. (9). when 37. Use Eq. (9) to compute () 42. time ",)\\ novonet A essieV smocha tion 38. Use Eq. (9) to compute V(Inr). doue (1,90)0) laib nooo merix the (a) 39. Figure 9 shows the graph of the equation s no daily .7)7 :mun F(x, y, z) = x2 + y2 - z2 - 12x -82 - 4=0 (a) Use the quadratic formula to solve for z as a function of x and y. This gives two formulas, depending on the choice of sign. aurb brew inlog lesbi I (b) Which formula defines the portion of the surface satisfying z > -4? Which formula defines the portion satisfying z 0, there is a unique value y = r(x) that solves the equation y' + 4xy = 16. (a) Show that dy/dx = -4y/(3y2 + 4x). (b) Let g(x) = f(x, r(x)), where f(x, y) is a function satisfying fx (1, 2) = 8, fy(1, 2) = 10 Use the Chain Rule to calculate g'(1). Note that r(1) = 2 because (x, y) = (1, 2) satisfies y' + 4xy = 16. 41. The pressure P, volume V, and temperature T of a van der Waals gas with n molecules (n constant) are related by the equation ( P + V 2 ) ( V - nb ) = nRT where a, b, and R are constant. Calculate a P/aT and aV/aP. 42. When x, y, and z are related by an equation F(x, y, z) = 0, we some- times write (@z/ax),, in place of az/ax to indicate that in the differentia- tion, z is treated as a function of x with y held constant (and similarly for the other variables). (a) Use Eq. (7) to prove the cyclic relation az ax 10 az (b) Verify Eq. (10) for F(x, y, z) = x + y + z =0. (c) Verify the cyclic relation for the variables P, V, T in the Ideal Gas Law PV - nRT = 0 (n and R are constants). 43. Show that if f(x) is differentiable and c # 0 is a constant, then mu(x, t) = f(x - ct) satisfies the so-called advection equation au au + C- at ax anig lessons 45. Prove that if f(x, y, z) is homogeneous of degree n, then fx(x, y, z) is homogeneous of degree n - 1. Hint: Either use the limit definition or apply the Chain Rule to f(Ax, Ay, Az). 46. Prove that if f (x, y, z) is homogeneous of degree n, then of of 11 + =nf x ay az 10 0 ax Hint: Let F(1) = f(tx, ty, tz) and calculate F'(1) using the Chain Rule














