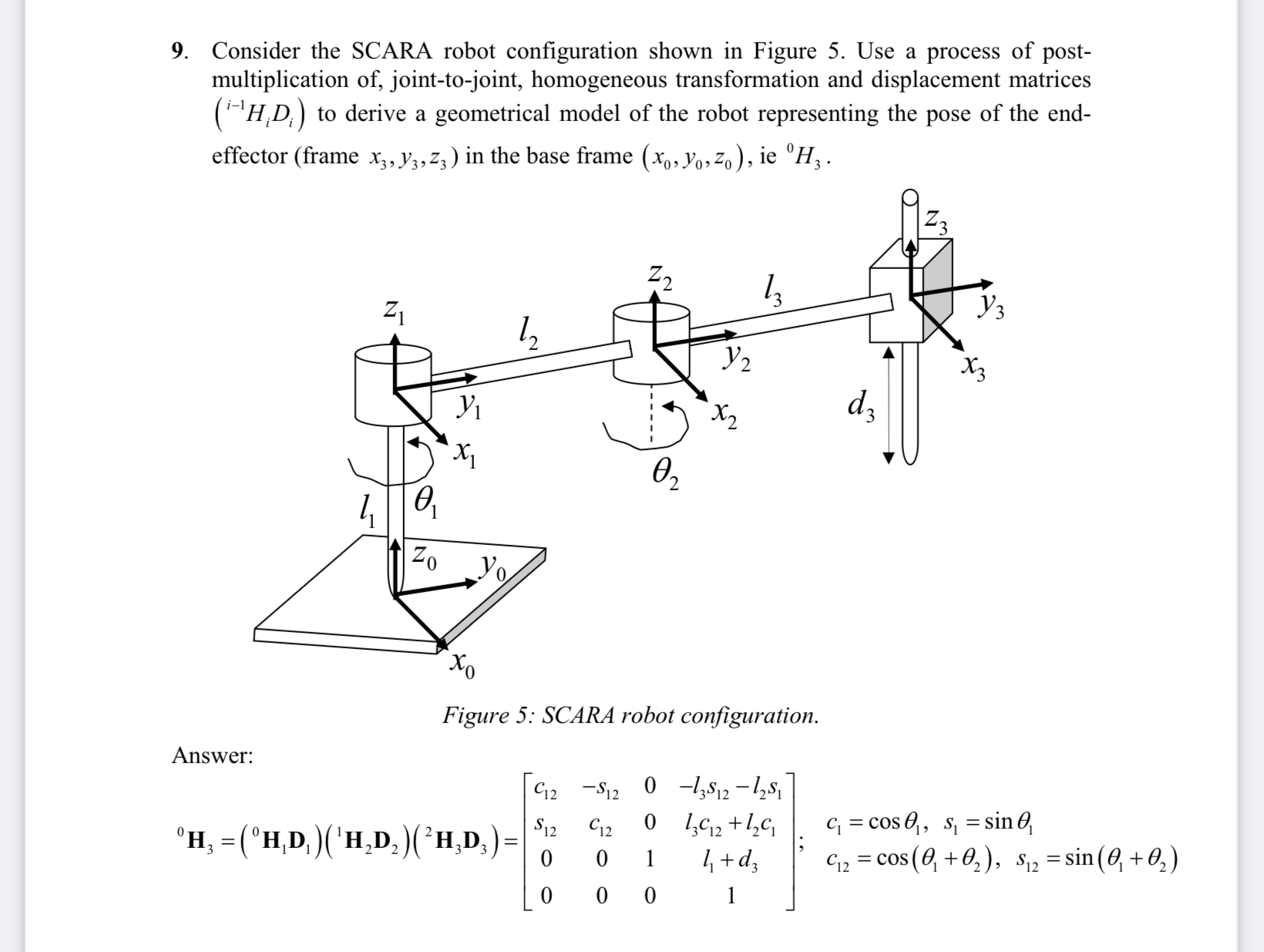
9. Consider the SCARA robot configuration shown in Figure 5. Use a process of post- multiplication of, joint-to-joint, homogeneous transformation and displacement matrices (i-H,D,) to derive a geometrical model of the robot representing the pose of the end- effector (frame x3, y3, Z3) in the base frame (x, y, z), ie H Z2 1, N Z Y2 y x2 d3 x 02 1, Zo 0 Answer: Figure 5: SCARA robot configuration. -S120-312-25 C12 H = (HD )( 'HD )( HD ) = $12 C12 0 1 013+12c 000 c = cos, s = sin 4+d3 C = cos(+0), S = sin (0+02) 1