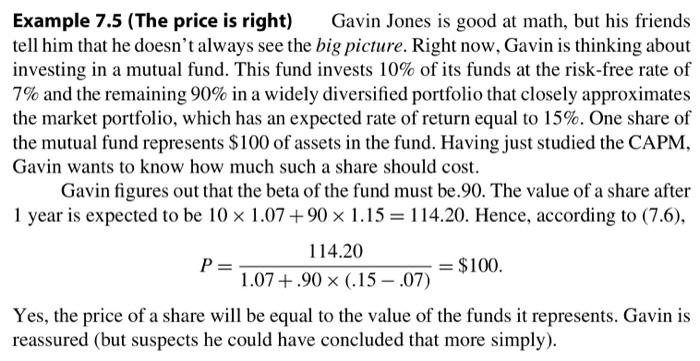
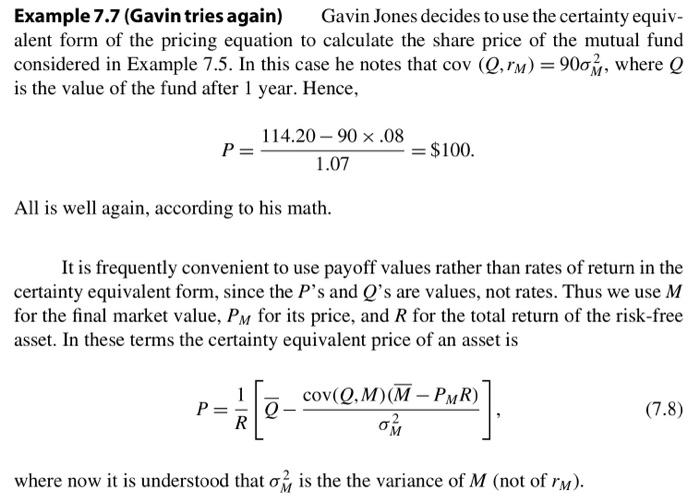
9. (Gavin's problem) Prove to Gavin Jones that the results he obtained in Examples 7.5 and 7.7 were not accidents. Specifically, for a fund with return arf + (1 -a)rm, show that both CAPM pricing formulas give the price of $100 worth of fund assets as $100. Example 7.5 (The price is right) Gavin Jones is good at math, but his friends tell him that he doesn't always see the big picture. Right now, Gavin is thinking about investing in a mutual fund. This fund invests 10% of its funds at the risk-free rate of 7% and the remaining 90% in a widely diversified portfolio that closely approximates the market portfolio, which has an expected rate of return equal to 15%. One share of the mutual fund represents $100 of assets in the fund. Having just studied the CAPM, Gavin wants to know how much such a share should cost. Gavin figures out that the beta of the fund must be.90. The value of a share after 1 year is expected to be 10 x 1.07+90 x 1.15 = 114.20. Hence, according to (7.6), 114.20 P= = $100. 1.07+.90 (.15 -.07) Yes, the price of a share will be equal to the value of the funds it represents. Gavin is reassured (but suspects he could have concluded that more simply). Example 7.7 (Gavin tries again) Gavin Jones decides to use the certainty equiv- alent form of the pricing equation to calculate the share price of the mutual fund considered in Example 7.5. In this case he notes that cov (Q, rm) = 900, where Q is the value of the fund after 1 year. Hence, 114.20 - 90 x .08 P= =$100. 1.07 All is well again, according to his math. It is frequently convenient to use payoff values rather than rates of return in the certainty equivalent form, since the P's and Q's are values, not rates. Thus we use M for the final market value, Pm for its price, and R for the total return of the risk-free asset. In these terms the certainty equivalent price of an asset is cov(Q,M)(M - PMR) lo =PqR)] (7.8) R where now it is understood that o is the the variance of M (not of rm). 9. (Gavin's problem) Prove to Gavin Jones that the results he obtained in Examples 7.5 and 7.7 were not accidents. Specifically, for a fund with return arf + (1 -a)rm, show that both CAPM pricing formulas give the price of $100 worth of fund assets as $100. Example 7.5 (The price is right) Gavin Jones is good at math, but his friends tell him that he doesn't always see the big picture. Right now, Gavin is thinking about investing in a mutual fund. This fund invests 10% of its funds at the risk-free rate of 7% and the remaining 90% in a widely diversified portfolio that closely approximates the market portfolio, which has an expected rate of return equal to 15%. One share of the mutual fund represents $100 of assets in the fund. Having just studied the CAPM, Gavin wants to know how much such a share should cost. Gavin figures out that the beta of the fund must be.90. The value of a share after 1 year is expected to be 10 x 1.07+90 x 1.15 = 114.20. Hence, according to (7.6), 114.20 P= = $100. 1.07+.90 (.15 -.07) Yes, the price of a share will be equal to the value of the funds it represents. Gavin is reassured (but suspects he could have concluded that more simply). Example 7.7 (Gavin tries again) Gavin Jones decides to use the certainty equiv- alent form of the pricing equation to calculate the share price of the mutual fund considered in Example 7.5. In this case he notes that cov (Q, rm) = 900, where Q is the value of the fund after 1 year. Hence, 114.20 - 90 x .08 P= =$100. 1.07 All is well again, according to his math. It is frequently convenient to use payoff values rather than rates of return in the certainty equivalent form, since the P's and Q's are values, not rates. Thus we use M for the final market value, Pm for its price, and R for the total return of the risk-free asset. In these terms the certainty equivalent price of an asset is cov(Q,M)(M - PMR) lo =PqR)] (7.8) R where now it is understood that o is the the variance of M (not of rm)









