A 2DOF system, has a modal matrix and corresponsing EOM in principal coordinates as follows: \[ \begin{array}{c} {[\phi]=\left\{\begin{array}{cc} 1 & 1 \\ 1 & -1 / 2 \end{array} ight\}} \\ {\left[\begin{array}{cc} 15 & 0 \\ 0 & 15 / 2 \end{array} ight]\left\{\begin{array}{l} \ddot{\eta}_{1} \\ \ddot{\eta}_{2} \end{array} ight\}+\left[\begin{array}{cc} 6 & 0 \\ 0 & 15 / 2 \end{array} ight]\left\{\begin{array}{l} \eta_{1} \\ \eta_{2} \end{array} ight\}=\left[\begin{array}{cc} 1 & 1 \\ 1 & -1 / 2 \end{array} ight]\left\{\begin{array}{l} F_{1} \\ F_{2} \end{array} ight\}} \end{array} \]

The steady state solutions can be written as \[ \begin{array}{l} x_{1}(t)=X_{1} \sin 15 t \\ x_{2}(t)=X_{2} \sin 15 t \end{array} \]

If \( \mathrm{F} 1=\mathrm{F} 2=\mathbf{2 5 9} \sin 15 \mathrm{t} \), calculate the steady state amplitude of X1. Write your answer in four decimal points.

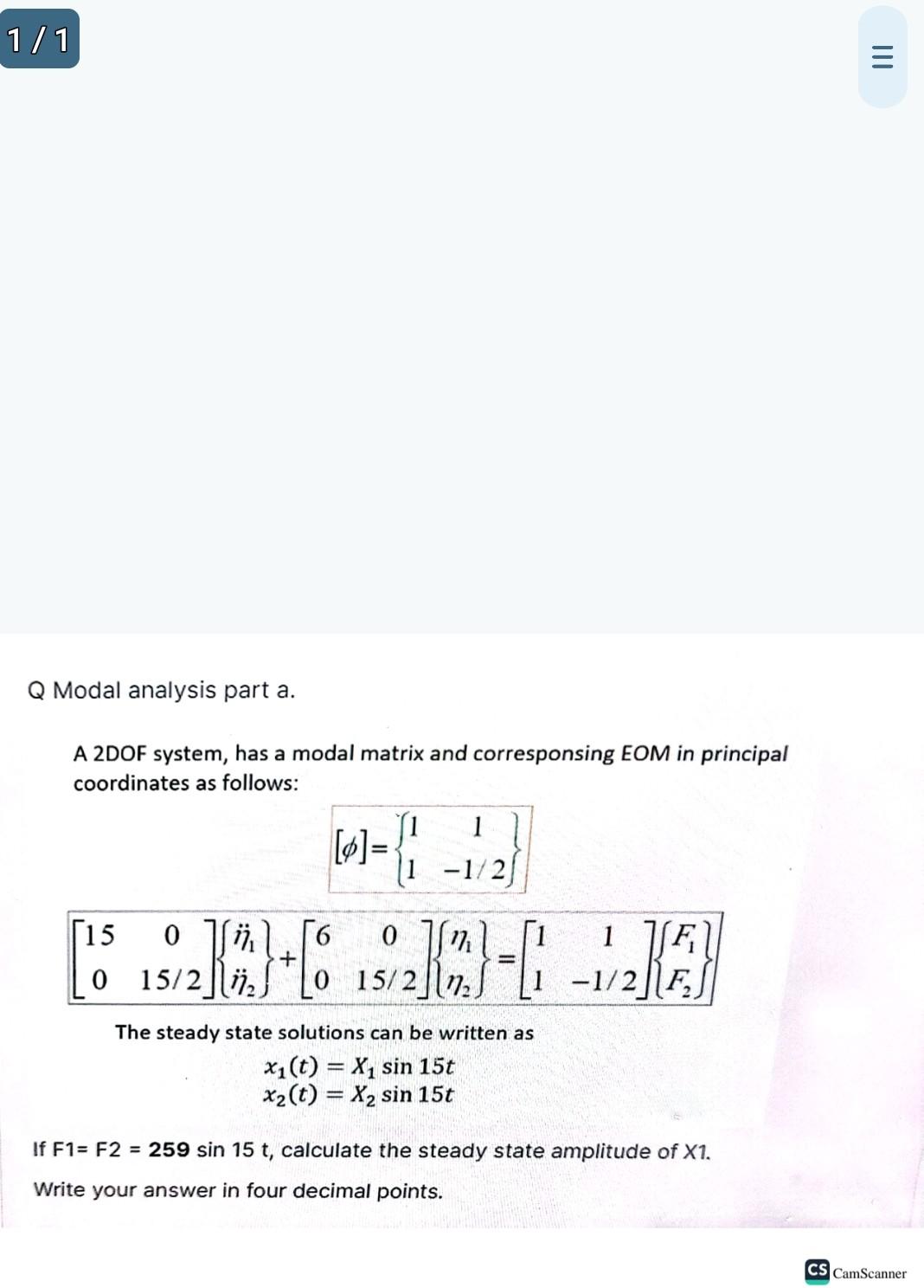
A 2DOF system, has a modal matrix and corresponsing EOM in principal coordinates as follows: []={1111/2} [150015/2]{12}+[60015/2]{12}=[1111/2]{F1F2} The steady state solutions can be written as x1(t)=X1sin15tx2(t)=X2sin15t If F1=F2=259sin15t, calculate the steady state amplitude of X1. Write your answer in four decimal points