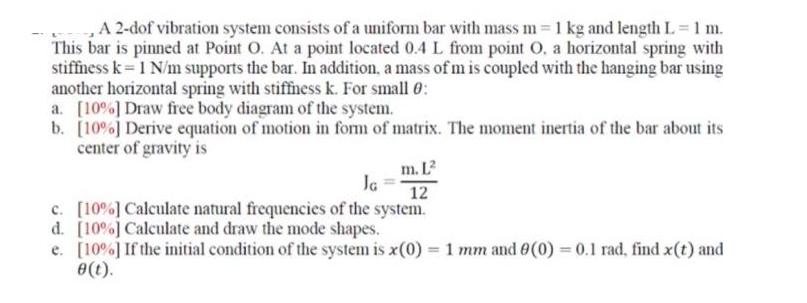
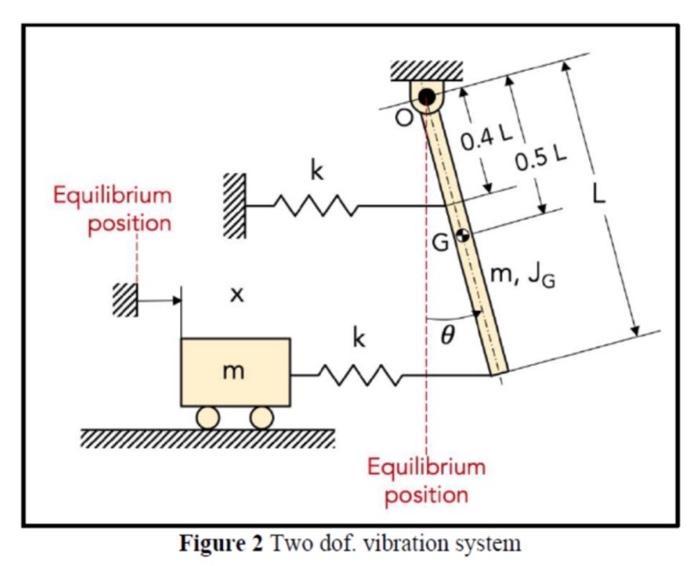
A 2-dof vibration system consists of a uniform bar with mass m= 1 kg and length L = 1 m. This bar is pinned at Point O. At a point located 0.4 L from point O. a horizontal spring with stiffness k = 1 N/m supports the bar. In addition, a mass of m is coupled with the hanging bar using another horizontal spring with stiffness k. For small 0: a. [10% ] Draw free body diagram of the system. b. [10% ] Derive equation of motion in form of matrix. The moment inertia of the bar about its center of gravity is JG m. L 12 c. [10% ] Calculate natural frequencies of the system. d. [10% ] Calculate and draw the mode shapes. e. [10% ] If the initial condition of the system is x(0) = 1 mm and 0 (0) = 0.1 rad, find x(t) and 0 (t). Equilibrium position Xx m k k G 0 0.4 L 0.5 L m, JG Equilibrium position Figure 2 Two dof. vibration system