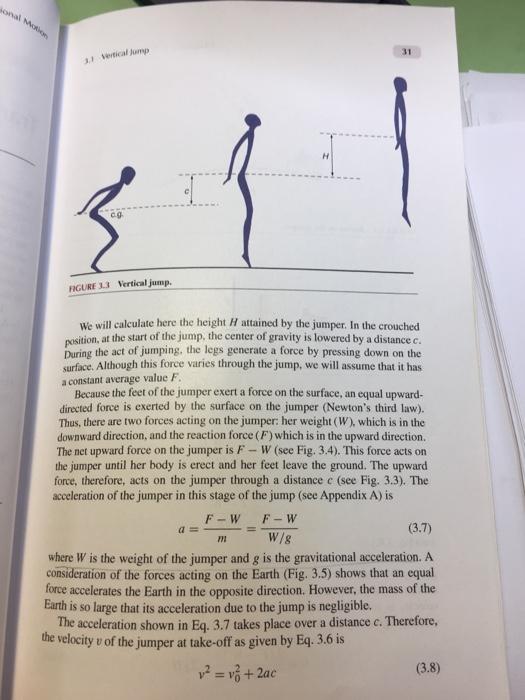
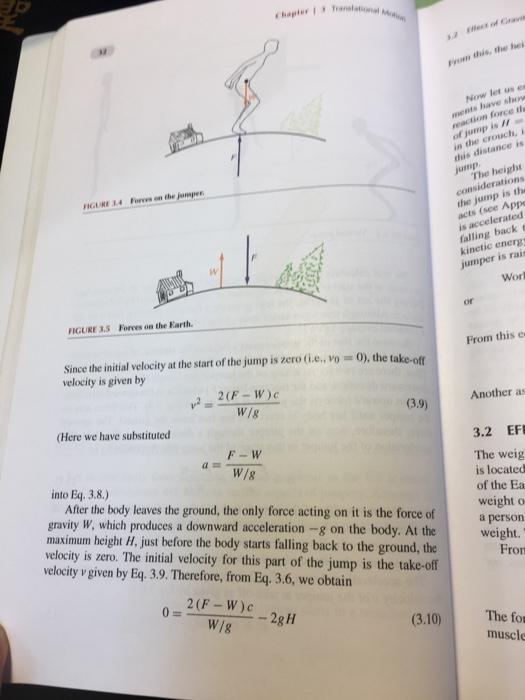
A 70-kg astronaut is loaded so heavily with equipment that on Earth he can jump only to a height of 10 cm. How high can he jump on the Moon? (Use the assumptions related to Eq. 3.11 in the text. As in the text, assume that the force generated by the legs is twice the unloaded weight of the person and the gravitational constant on the moon is 1/6 that on Earth.) 3-2. onal Motion 31 31 etical Jump FIGURE LI Vertical jump. He will calculate here the height H attained by the jumper. In the crouched piition, at the start of the jump. the center of gravity is lowered by a distance c. During the act of jumping, the legs generate a force by pressing down on the surface. Although this force varies through the jump, we will assume that it has a constant average value F. Because the feet of the jumper exert a force on the surface, an equal upward- directed force is exerted by the surface on the jumper (Newton's third law). Thus, there are two forces acting on the jumper: her weight (W), which is in the downward direction, and the reaction force (F) which is in the upward direction. The net upward force on the jumper is F - W (see Fig. 3.4). This force acts on the jumper until her body is erect and her feet leave the ground. The upward force, therefore, acts on the jumper through a distance c (see Fig. 3.3). The acceleration of the jumper in this stage of the jump (see Appendix A) is F-W F-W a (3.7) W/g where W is the weight of the jumper and g is the gravitational acceleration. A consideration of the forces acting on the Earth (Fig. 3.5) shows that an equal force accelerates the Earth in the opposite direction. However, the mass of the Earth is so large that its acceleration due to the jump is negligible. The acceleration shown in Eq. 3.7 takes place over a distance e. Therefore, the velocity v of the jumper at take-off as given by Eq. 3.6 is v = v + 2ac (3.8) %3D Chapter 3 Translational M 13 ect of Gv From this, the he Now let us ee ments have stue reaction force of jump is H in che crouch, i this distance is jump. The height considerations the jump is the acts (see Appe is accelerated falling back e kinetic energS jumper is rain Worl FNGURE 14 Fre en the jumper IGURE 15 Forces on the Earth. or Since the initial velocity at the start of the jump is zero (i.e., vo 0), the take-ofr velocity is given by From this e 2(F- W)c W/g (3.9) Another as (Here we have substituted F-W 3.2 EFE W/8 The weig into Eq. 3.8.) After the body leaves the ground, the only force acting on it is the force of gravity W, which produces a downward acceleration -g on the body. At the maximum height H. just before the body starts falling back to the ground, the velocity is zero. The initial velocity for this part of the jump is the take-off velocity v given by Eq. 3.9. Therefore, from Eq. 3.6, we obtain is located of the Ea weight o a person weight. From 2(F-W)c - 2gH W/g (3.10) The fom muscle Nertu 33 32 Effect of Gravity on the Vertical Jump From this, the height of the jump is (F - W)e H (3.11) W Now let us estimate the numerical value for the height of the jump. Experi- ments have shown that in a good jump a well-built person generates an average reaction force that is twice his/her weight (i.e., F = 2W). In that case, the height of jump is H = c. The distance c, which is the lowering of the center of gravity in the crouch, is proportional to the length of the legs. For an average person, this distance is about 60cm, which is our estimate for the height of a vertical jump. The height of a vertical jump can also be computed very simply from energy considerations. The work done on the body of the jumper by the force F during the jump is the product of the force F and the distance c over which this force acts (see Appendix A). This work is converted to kinetic energy as the jumper is accelerated upward. At the full height of the jump H (before the jumper starts falling back to ground), the velocity of the jumper is zero. At this point, the kinetic energy is fully converted to potential energy as the center of mass of the jumper is raised to a height (c + H). Therefore, from conservation of energy, Work done on the body = Potential energy at maximum height or Fc = W(c+ H) (3.12) From this equation the height of the jump is, as before, (F-W)c H W Ahother aspect of the vertical jump is examined in Exercise 3-1. 3.2 EFFECT OF GRAVITY ON THE VERTICAL JUMP fiha planet on which it