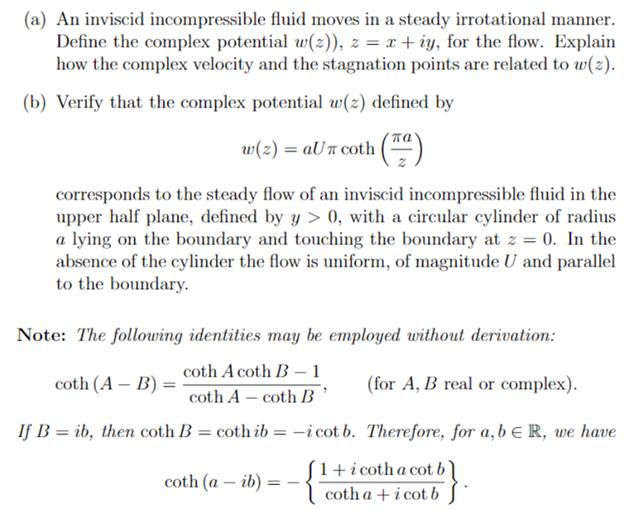
(a) An inviscid incompressible fluid moves in a steady irrotational manner. Define the complex potential w(2)), z = x+ iy, for the flow. Explain how the complex velocity and the stagnation points are related to w(z). (b) Verify that the complex potential w(z) defined by w(z) = aUn coth () corresponds to the steady flow of an inviscid incompressible fluid in the upper half plane, defined by y > 0, with a circular cylinder of radius a lying on the boundary and touching the boundary at z = 0. In the absence of the cylinder the flow is uniform, of magnitude U and parallel to the boundary. Note: The following identities may be employed without derivation: coth A coth B 1 coth (A B) = (for A, B real or complex). coth A coth B If B = ib, then coth B = coth ib = -i cot b. Therefore, for a,beR, we have %3D (1+i coth a cot b' I coth a +i cot b coth (a ib) %3D