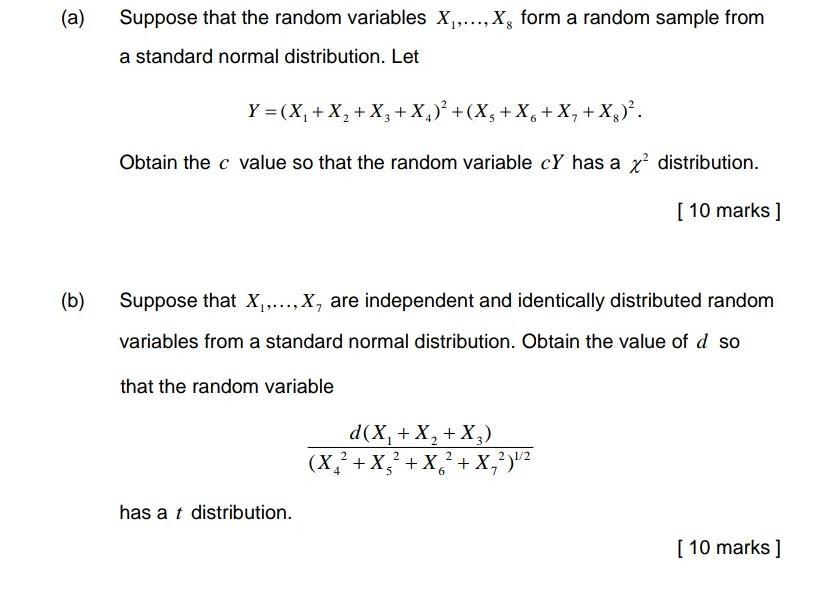
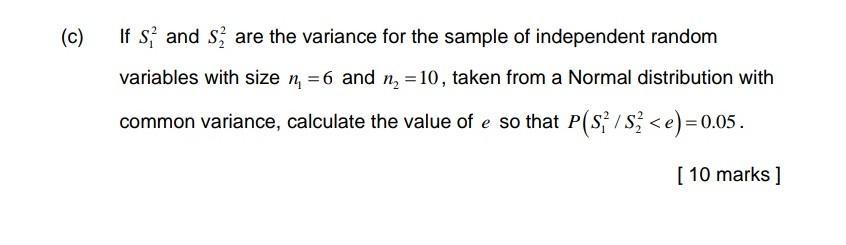
(a) (b) Suppose that the random variables X,..., X, form a random sample from a standard normal distribution. Let Y = (X + X + X + X) + (X + X6 + X + Xg). 7 Obtain the c value so that the random variable cY has a x distribution. [10 marks] Suppose that X,..., X, are independent and identically distributed random variables from a standard normal distribution. Obtain the value of d so that the random variable has at distribution. d(X + X + X3) 2 2 2 (X + X + X + X)/ 4 7 [10 marks] (c) If S and S are the variance for the sample of independent random variables with size n =6 and n =10, taken from a Normal distribution with common variance, calculate the value of e so that P(S/S