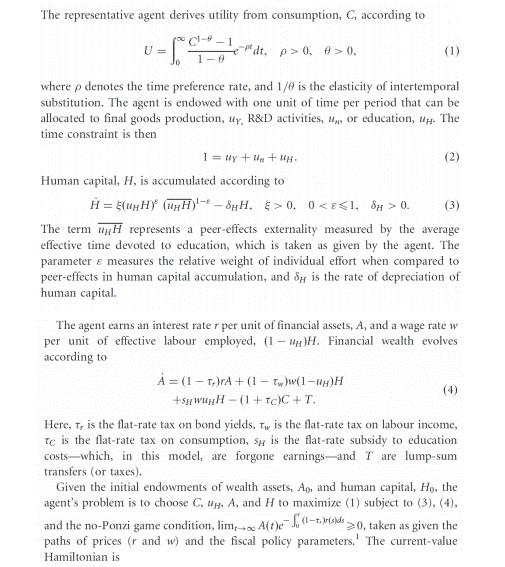
a) Complete the sentence above writing down the Hamiltonian.

b) Apply the maximum principle and write down the conditions that it yields.

The representative agent derives utility from consumption, C, according to C--1 pedt. p>0, > 0 1- U-14 where p denotes the time preference rate, and 1/@ is the elasticity of intertemporal substitution. The agent is endowed with one unit of time per period that can be allocated to final goods production, uy, R&D activities, or education, up. The time constraint is then 1 = y + + (2) Human capital, H, is accumulated according to H = $(UHY (UH) -- 8H. > 0. 00. (3) The term WH represents a peer-effects externality measured by the avera effective time devoted to education, which is taken as given by the agent. The parameter e measures the relative weight of individual effort when compared to peer-effects in human capital accumulation, and 8y is the rate of depreciation of human capital. The agent earns an interest rater per unit of financial assets, A, and a wage rate w per unit of effective labour employed, (1 W). Financial wealth evolves according to A = (1 - 1)/A+ (1 - Tw(1-WH +5WH - (1 + Tc/C+T. Here, T, is the flat-rate tax on bond yields, T, is the flat-rate tax on labour income, Tc is the flat-rate tax on consumption, sy is the flat-rate subsidy to education costswhich, in this model, are forgone earningsand T are lump sum transfers (or taxes). Given the initial endowments of wealth assets, Am, and human capital, Ho, the agent's problem is to choose C, A, and H to maximize (1) subject to (3), (4), and the no-Ponzi game condition, lim Alte-La-Plak>0, taken as given the paths of prices (r and w) and the fiscal policy parameters. The current-value Hamiltonian is The representative agent derives utility from consumption, C, according to C--1 pedt. p>0, > 0 1- U-14 where p denotes the time preference rate, and 1/@ is the elasticity of intertemporal substitution. The agent is endowed with one unit of time per period that can be allocated to final goods production, uy, R&D activities, or education, up. The time constraint is then 1 = y + + (2) Human capital, H, is accumulated according to H = $(UHY (UH) -- 8H. > 0. 00. (3) The term WH represents a peer-effects externality measured by the avera effective time devoted to education, which is taken as given by the agent. The parameter e measures the relative weight of individual effort when compared to peer-effects in human capital accumulation, and 8y is the rate of depreciation of human capital. The agent earns an interest rater per unit of financial assets, A, and a wage rate w per unit of effective labour employed, (1 W). Financial wealth evolves according to A = (1 - 1)/A+ (1 - Tw(1-WH +5WH - (1 + Tc/C+T. Here, T, is the flat-rate tax on bond yields, T, is the flat-rate tax on labour income, Tc is the flat-rate tax on consumption, sy is the flat-rate subsidy to education costswhich, in this model, are forgone earningsand T are lump sum transfers (or taxes). Given the initial endowments of wealth assets, Am, and human capital, Ho, the agent's problem is to choose C, A, and H to maximize (1) subject to (3), (4), and the no-Ponzi game condition, lim Alte-La-Plak>0, taken as given the paths of prices (r and w) and the fiscal policy parameters. The current-value Hamiltonian is