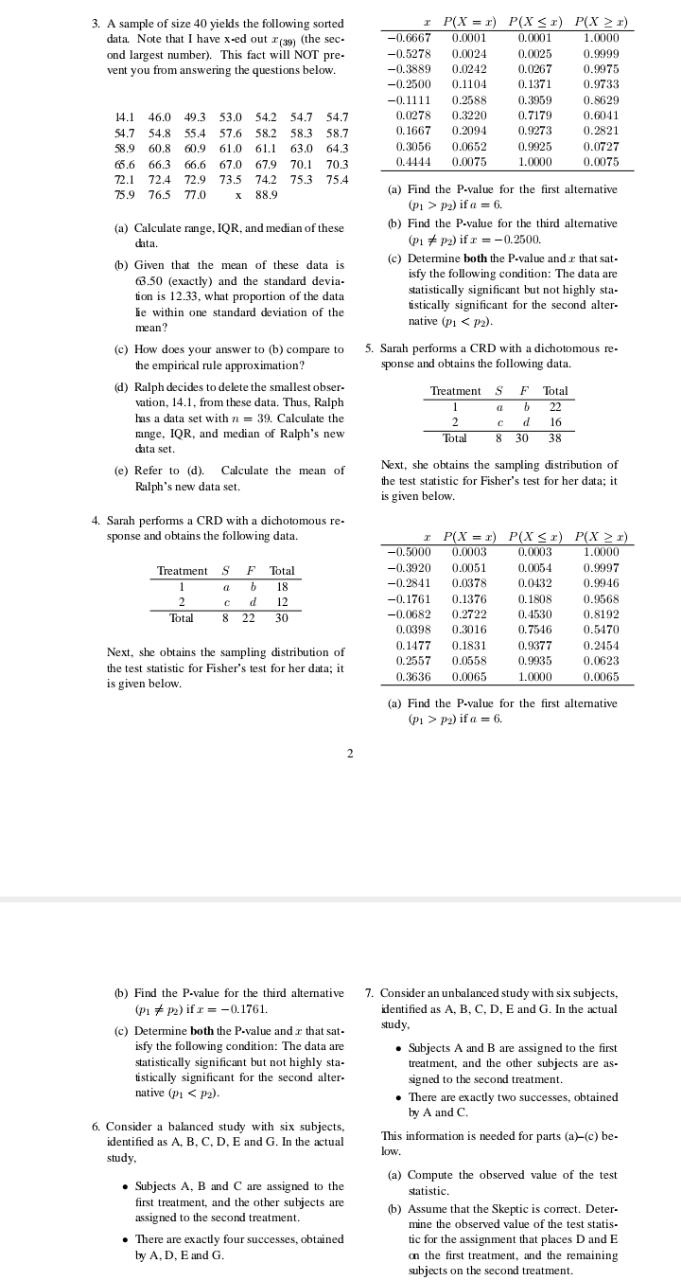
(a) Compute a 95% t-confidence interval for 8. Does 95% confidence mean that the probability # is in the interval you just found is 95%? (b) Now suppose that you learn that of = 25. Compute a 95% 2-confidence interval for 0. How does this compare to the interval in (a)? c) Let X be the number of points scored in a game. Suppose that your friend is a confirmed Bayesian with a priori belief e ~ N(60, 16) and that X ~ N(0, 25). He computes a 95% probability interval for 0, given the data in part (a). How does this interval compare to the intervals in (a) and (b)? (d) Which of the three intervals constructed above do you prefer? Why? 2. The volume in a set of wine bottles is known to follow a N(#, 25) distribution. You take a sample of the bottles and measure their volumes. How many bottles do you have to sample to have a 95% confidence interval for / with width 1? 3. Suppose data ri,..., In are i.i.d. and drawn from N(, o'), where a and o are unknown. Suppose a data set is taken and we have n = 49, sample mean i = 92 and sample standard deviation s = 0.75. Find a 90% confidence interval for p. 4. You do a poll to see what fraction p of the population supports candidate A over candidate B. (a) How many people do you need to poll to know p to within 1%% with 95% confidence. (b) Let p be the fraction of the population who prefer candidate A. If you poll 400 people, how many have to prefer candidate A to so that the 90% confidence interval is entirely above p = 0.5. Post-exam 2 practice , Spring 2014 19 5. Suppose you made 40 confidence intervals with confidence level 95%%. About how many of them would you expect to be "wrong'? That is, how many would not actually contain the parameter being estimated? Should you be surprised if 10 of them are wrong?3. A sample of size 40 yields the following sorted P(X = x) P(X x) P(X 2 1) data. Note that I have x-ed out I(39) (the sec- -0.6667 0.0001 0.0001 1.0000 ond largest number). This fact will NOT pre- -0.5278 0.0024 0.0025 0.9999 vent you from answering the questions below. -0.3889 0.0242 0.0267 0.9975 -0.2500 0.1104 0.1371 0.9733 -0.1111 0.2588 0.3959 0.8629 14.1 46.0 493 53.0 54.2 54.7 54.7 0.0278 0.3220 0.7179 0.6041 7 54.8 55.4 57.6 58.2 58.3 58.7 0.1667 0.2094 0.9273 0.2821 58.9 60.8 60.9 61.0 61.1 63.0 64.3 0.3056 0.0652 0.9925 0.0727 65.6 66.3 66.6 67.0 67.9 70.1 70.3 0.4444 0.0075 1.0000 0.0075 72.1 72.4 72.9 73.5 742 75.3 754 75.9 765 77.0 X 88.9 (a) Find the P-value for the first alternative (pi > p2) if a = 6. (a) Calculate range. IQR. and median of these (b) Find the P-value for the third alternative data. (pi # p2) ifr = -0.2500, (b) Given that the mean of these data is (c) Determine both the P-value and r that sat- 63.50 (exactly) and the standard devia- isfy the following condition: The data are tion is 12.33, what proportion of the data statistically significant but not highly sta- he within one standard deviation of the istically significant for the second alter- mean? native (p1 >) -0.5000 0.0003 0.0003 1.0000 Treatment S F Total -0.3920 0.0051 0.0054 0.9997 b 18 -0.2841 0.0378 0.0432 0.9946 2 C d 12 -0.1761 0.1376 0.1808 0.9568 Total 8 22 30 -0.0682 0.2722 0.4530 0.8192 0.0398 0.3016 0.7546 0.5470 Next, she obtains the sampling distribution of 0.1477 0.1831 0.9377 0.2454 the test statistic for Fisher's test for her data; it 0.2557 0.0558 0.9935 0.0623 is given below. 0.3636 0.0065 1.0000 0.0065 (a) Find the P-value for the first alternative (p1 > pz) ifa = 6. 2 (b) Find the P-value for the third alternative 7. Consider an unbalanced study with six subjects, (Pi # p) ifx = -0.1761. identified as A, B, C, D. E and G. In the actual (c) Determine both the P-value and r that sat- study, isfy the following condition: The data are . Subjects A and B are assigned to the first statistically significant but not highly sta- treatment, and the other subjects are as- listically significant for the second alter- signed to the second treatment. native (pi