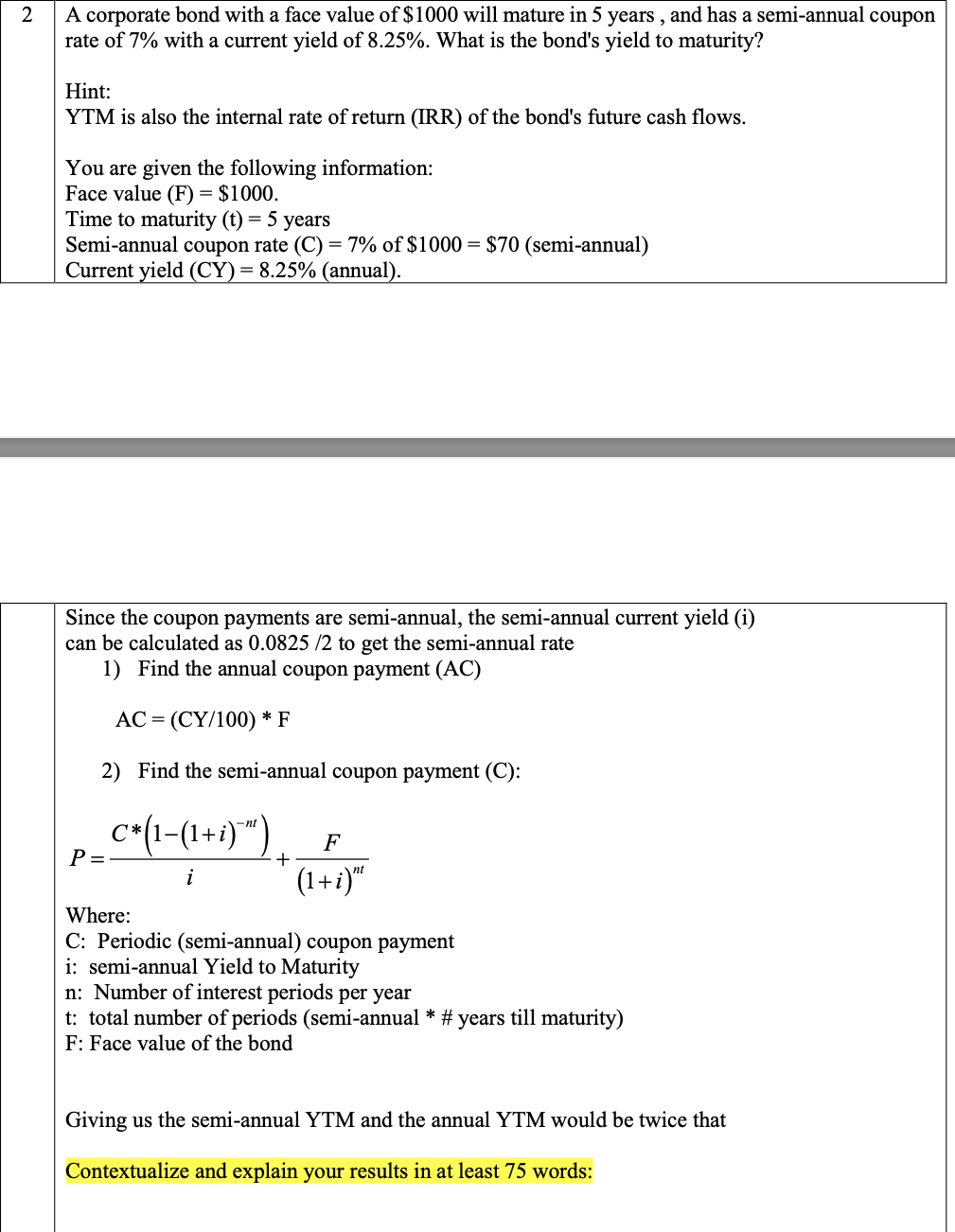
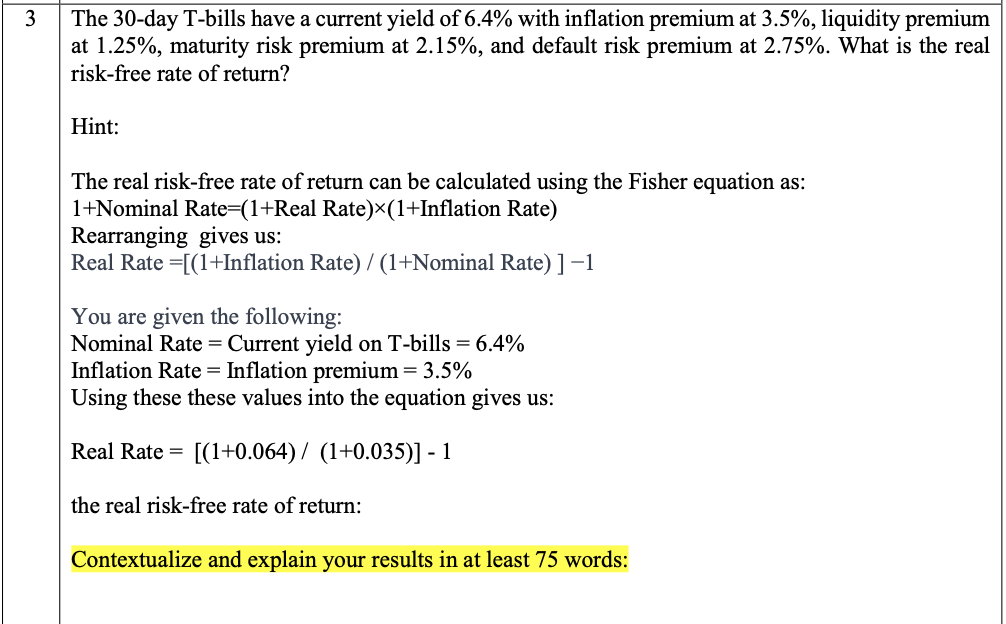
A corporate bond pays semi-annual coupons at 8% and will mature in 8 years. What is the price of the bond if its face value is $1000 and its yield to maturity is 9%? Hint: The price of a bond is the present value of its future cash flows, which include both the periodic coupon payments and the face value at maturity. The price (P) of a bond can be expressed as: P=- C*(1-(1+i)") F Where: + (1+i) C: Periodic coupon payment (semi-annual in this case) i: Yield to Maturity per period (semi-annual in this case) n: Number of interest periods per year t: Number of years to maturity F: Face value of the bond You are given the following: C (semi-annual coupon payment) = 8% of $1000 = $80 i (yield to maturity per period)=9%/2/100 = 0.045 (semi-annual) n (semi-annual periods per year) - 2 t (years to maturity)=8 F (face value) = $1000 Calculate the Bond Value: P=80(1-(1+0.045)-2) 0.045 1000 (1+0.045) Contextualize and explain your results in at least 75 words: 2 A corporate bond with a face value of $1000 will mature in 5 years, and has a semi-annual coupon rate of 7% with a current yield of 8.25%. What is the bond's yield to maturity? Hint: YTM is also the internal rate of return (IRR) of the bond's future cash flows. You are given the following information: Face value (F) = $1000. Time to maturity (t) = 5 years Semi-annual coupon rate (C) = 7% of $1000 = $70 (semi-annual) Current yield (CY) = 8.25% (annual). Since the coupon payments are semi-annual, the semi-annual current yield (i) can be calculated as 0.0825/2 to get the semi-annual rate P = 1) Find the annual coupon payment (AC) AC = (CY/100) * F 2) Find the semi-annual coupon payment (C): c(1-(1+i)) + Where: + F (1+i)" C: Periodic (semi-annual) coupon payment i: semi-annual Yield to Maturity n: Number of interest periods per year t: total number of periods (semi-annual * # years till maturity) F: Face value of the bond Giving us the semi-annual YTM and the annual YTM would be twice that Contextualize and explain your results in at least 75 words: 3 The 30-day T-bills have a current yield of 6.4% with inflation premium at 3.5%, liquidity premium at 1.25%, maturity risk premium at 2.15%, and default risk premium at 2.75%. What is the real risk-free rate of return? Hint: The real risk-free rate of return can be calculated using the Fisher equation as: 1+Nominal Rate=(1+Real Rate)(1+Inflation Rate) Rearranging gives us: Real Rate =[(1+Inflation Rate) / (1+Nominal Rate) ] -1 You are given the following: Nominal Rate = Current yield on T-bills = 6.4% Inflation Rate Inflation premium = 3.5% Using these these values into the equation gives us: = Real Rate [(1+0.064)/ (1+0.035)] - 1 the real risk-free rate of return: Contextualize and explain your results in at least 75 words: 4 ABC Corp. will generate $30 million in free cash flows next year, and expected to grow at a constant rate of 3.5% per year. The firm has no debt or preferred stock and its WACC is 11% with 20 million shares outstanding. What is its stock value per share? Hint: We can use the Gordon Growth Model (Dividend Discount Model) to calculate the stock value per share: Stock Value per Share = [Expected Dividends or Free Cash Flows] / [Required Rate of Return - Growth Rate] Since ABC Corp. has no debt or preferred stock, and the free cash flows are expected to grow at a constant rate, we can use them as if they were dividends. So, the modified formula becomes: Stock Value per Share [Expected Free Cash Flows * (1+ Growth Rate)]/[Required Rate of Return - Growth Rate] You are given the following information: Expected Free Cash Flows next year = $30 million Growth Rate = 3.5% Required Rate of Return (WACC) = 11% Plug in these values in the modified formula to get stock value per share: Stock Value per Share = [30(1+0.035)]/[0.11-0.035] Contextualize and explain your results in at least 75 words: 5 PQR Corp. shares currently sell for $37. It just paid a dividend of $2.25, and expected to grow at a constant rate of 4% per year. What will be the expected stock price next year and the required rate of return? Hint: We can use the Gordon Growth Model (Dividend Discount Model) to calculate the expected stock price next year : P D*(1+g) (r-g) Where: Do current dividend ($2.25) g=constant growth rate of dividends (4%) r = required rate of return Using DDM we can calculate expected stock price next year as: P = [2.25 * (1.04)]/ (r-0.04) We also know that expected stock price next year will increase with the growth in dividends as: P = P *(1+ g) = 37 *(1+0.04) Using the two equations through substitution will give us the required rate of return (r): Contextualize and explain your results in at least 75 words: