a) discuss the analysis of thin plate

b) i) Check for the admissibility of the assumed deflection function. ii) derive an expression for the maximum deflection and maximum moments using Navier Method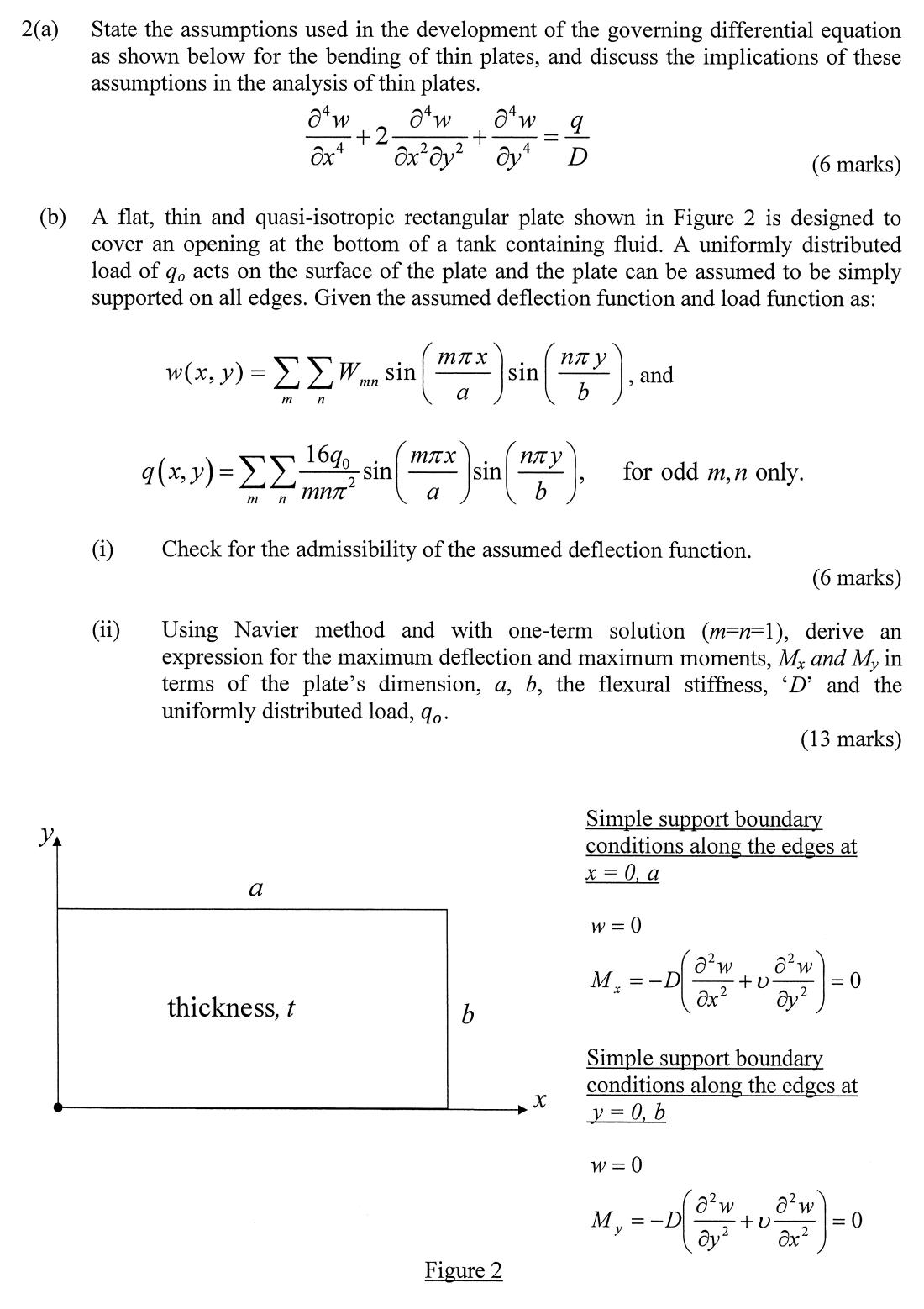

2(a) State the assumptions used in the development of the governing differential equation as shown below for the bending of thin plates, and discuss the implications of these assumptions in the analysis of thin plates. de w dw +2. 0x4 YA (6 marks) (b) A flat, thin and quasi-isotropic rectangular plate shown in Figure 2 is designed to cover an opening at the bottom of a tank containing fluid. A uniformly distributed load of qo acts on the surface of the plate and the plate can be assumed to be simply supported on all edges. Given the assumed deflection function and load function as: (i) (ii) w(x, y)= [Wn sin mn at w 9 + oxy dy D n a MTX q(x, y) = [[ 6 sin sin 16q MTX mn a m n thickness, t a - sin b Figure 2 nny b Check for the admissibility of the assumed deflection function. (6 marks) Using Navier method and with one-term solution (m=n=1), derive an expression for the maximum deflection and maximum moments, Mx and My in terms of the plate's dimension, a, b, the flexural stiffness, 'D' and the uniformly distributed load, 9o. (13 marks) nn y X and for odd m, n only. Simple support boundary conditions along the edges at x = 0, a w = 0 Mx =-D d w x +v w dy : 0 Simple support boundary conditions along the edges at y = 0, b W = 0 w M --D+1)-0 2