Answered step by step
Verified Expert Solution
Question
1 Approved Answer
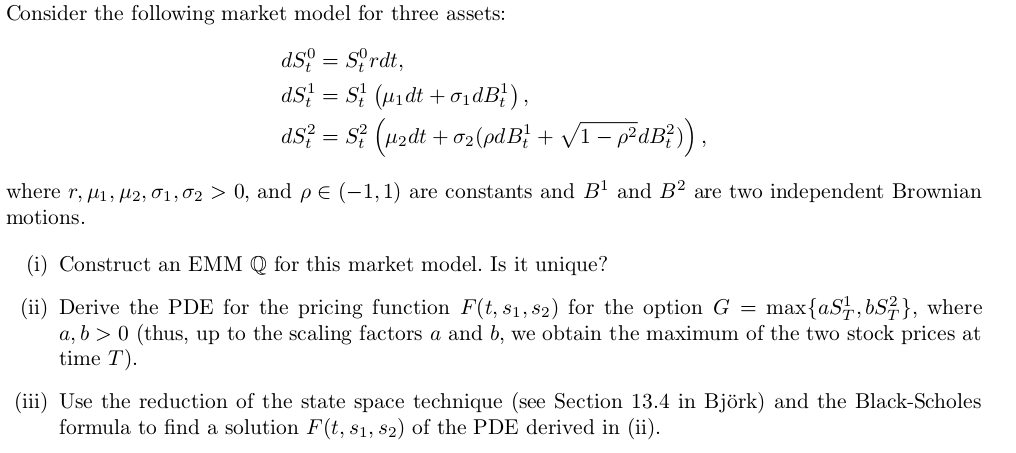
A financial math problem concerning brownian motion modeling. Consider the following market model for three assets: ds; = Sordt, dS7 = S; (pldt +0dB/), ds}
A financial math problem concerning brownian motion modeling.
Consider the following market model for three assets: ds; = Sordt, dS7 = S; (pldt +0dB/), ds} = $} (undt + 02 (pdB; + V1 p?dB})), where r, M1, M2,01,02 > 0, and pe (-1,1) are constants and Bl and B2 are two independent Brownian motions. (i) Construct an EMM Q for this market model. Is it unique? (ii) Derive the PDE for the pricing function F(t, 81, 82) for the option G max{aS1,6S{}, where a, b> 0 (thus, up to the scaling factors a and b, we obtain the maximum of the two stock prices at time T). (iii) Use the reduction of the state space technique (see Section 13.4 in Bjrk) and the Black-Scholes formula to find a solution F(t, 81, 82) of the PDE derived in (ii). Consider the following market model for three assets: ds; = Sordt, dS7 = S; (pldt +0dB/), ds} = $} (undt + 02 (pdB; + V1 p?dB})), where r, M1, M2,01,02 > 0, and pe (-1,1) are constants and Bl and B2 are two independent Brownian motions. (i) Construct an EMM Q for this market model. Is it unique? (ii) Derive the PDE for the pricing function F(t, 81, 82) for the option G max{aS1,6S{}, where a, b> 0 (thus, up to the scaling factors a and b, we obtain the maximum of the two stock prices at time T). (iii) Use the reduction of the state space technique (see Section 13.4 in Bjrk) and the Black-Scholes formula to find a solution F(t, 81, 82) of the PDE derived in (ii)Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


