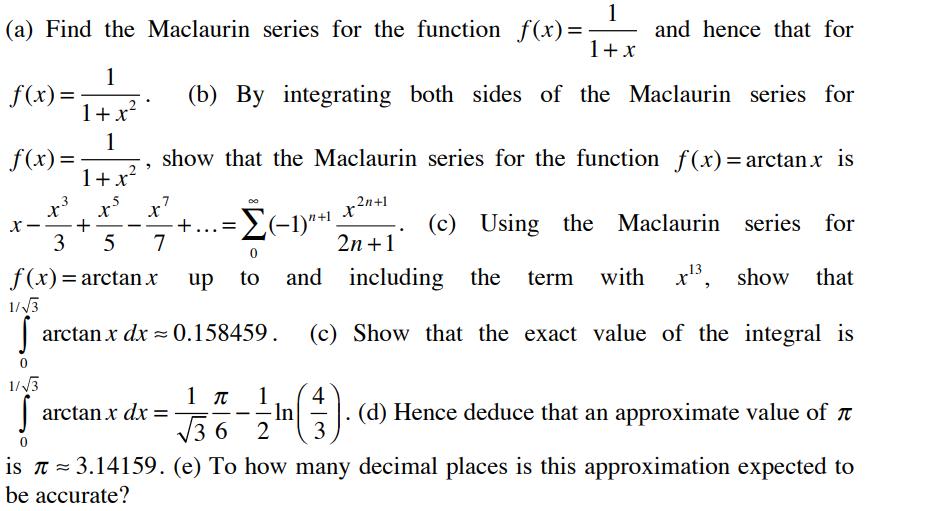
(a) Find the Maclaurin series for the function f(x)= 1 f(x) = - f(x)= 1+x. 1 X - 1 1 + x (b) By integrating both sides of the Maclaurin series for 1+x show that the Maclaurin series for the function f(x)= arctanx is x xn+1 - + ... = [(-1)"+ (c) Using the Maclaurin series for 7 2n+1 up to and including the term with x, show that (c) Show that the exact value of the integral is x x + 3 5 f(x) = arctan x 1/3 | arctan.x dx = 0.158459. 1/3 farctan x dx = 1 Do 1 - and hence that for In 2 4 . (d) Hence deduce that an approximate value of 3 36 = is 3.14159. (e) To how many decimal places is this approximation expected to be accurate?