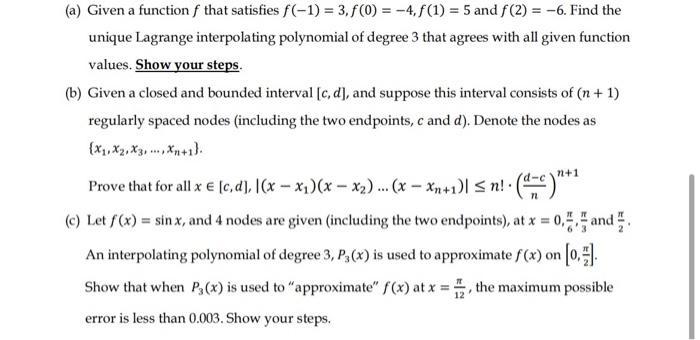
(a) Given a function f that satisfies f(-1) = 3,f(0) = -4,f(1) = 5 and f(2)=-6. Find the unique Lagrange interpolating polynomial of degree 3 that agrees with all given function values. Show your steps. (b) Given a closed and bounded interval [c, d], and suppose this interval consists of (n + 1) regularly spaced nodes (including the two endpoints, c and d). Denote the nodes as Prove that for all x [c,d], [(x-x)(x x) ... (x - xn+1)] n!. (d=)n+ (C) Let f(x) = sinx, and 4 nodes are given (including the two endpoints), at x =0, and An interpolating polynomial of degree 3, P, (x) is used to approximate f(x) on [0]. Show that when P, (x) is used to "approximate" f(x) at x = =, the maximum possible error is less than 0.003. Show your steps.