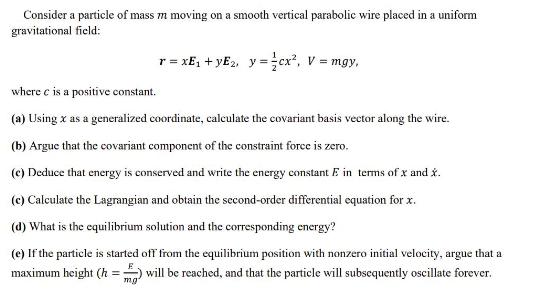
Consider a particle of mass m moving on a smooth vertical parabolic wire placed in a uniform gravitational field: r = xE+yE, y = cx, V = mgy, where c is a positive constant. (a) Using x as a generalized coordinate, calculate the covariant basis vector along the wire. (b) Argue that the covariant component of the constraint force is zero. (e) Deduce that energy is conserved and write the energy constant E in terms of x and X. (c) Calculate the Lagrangian and obtain the second-order differential equation for x. (d) What is the equilibrium solution and the corresponding energy? (e) If the particle is started off from the equilibrium position with nonzero initial velocity, argue that a maximum height (h=) will be reached, and that the particle will subsequently oscillate forever. mg