Question: (a) (i) Let f: JR be a real function defined on the non-empty open interval JC R. Define what it means for f to

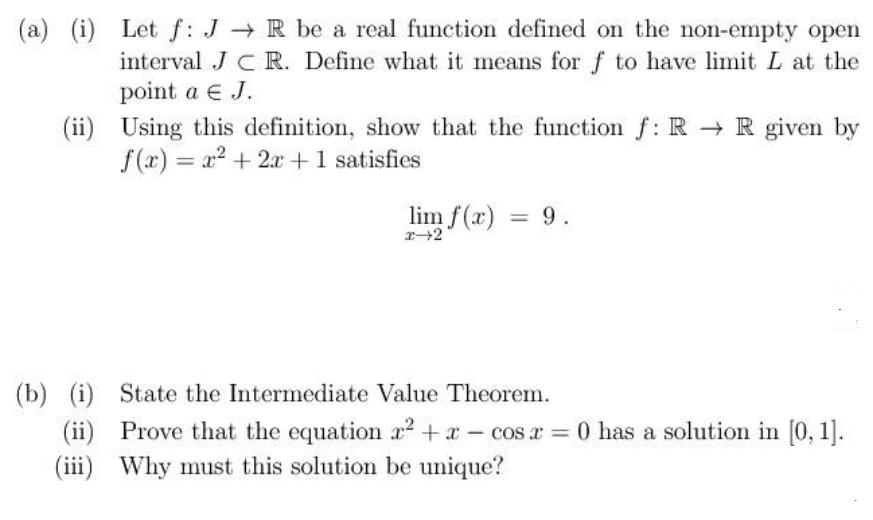
(a) (i) Let f: JR be a real function defined on the

(a) (i) Let f: JR be a real function defined on the non-empty open interval JC R. Define what it means for f to have limit L at the point a J. (ii) Using this definition, show that the function f: RR given by f(x)= x + 2x + 1 satisfies lim f(x) = 9. x-2 (b) (i) State the Intermediate Value Theorem. (ii) - Prove that the equation x + x = cos x = 0 has a solution in [0, 1]. (iii) Why must this solution be unique?

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

Define what it means to perform decision making under assumed certainty, risk, and uncertainty.

In your own words, describe what it means for a function to be continuous at a point.

Explain what it means for suppliers to have a credible threat of forward integration.

Use the Intermediate Value Theorem to prove that (cos t)t3 + 6 sin5 t - 3 = 0 has a real solution between 0 and 2.

Use the intermediate Value Theorem to prove that the equation x5 - 4x3 - 3x + 1 = 0 has at least one solution between x = 2 and x = 3.

Use the Intermediate Value Theorem to prove that each equation has a solution. Then use a graphing calculator or computer grapher to solve the equations. 2 sin x = x (three roots). Make sure you are...

Use the Intermediate Value Theorem to prove that each equation has a solution. Then use a graphing calculator or computer grapher to solve the equations. x + 1 + x = 4

Use the Intermediate Value Theorem to prove that x3 + 3x - 2 = 0 has a real solution between 0 and 1.

Use the Intermediate Value Theorem to prove that each equation has a solution. Then use a graphing calculator or computer grapher to solve the equations. x x = 2

A fixed point of a function f is a number in its domain such that f(c) = c. (The function doesnt move ; it stays fixed.) (a) Sketch the graph of a continuous function with domain [0, 1] whose range...

a) Describe the endbehaviour of the polynomialf(x)=3x^34x^42x^5 Explain how the degree and leading coefficient of the function help you identify the end behaviour of the polynomial thx

1. Is working at a job for $15 an hour a manageable earning wage? Explain. 2. If necessary, what would you adjust in this budget? For example, the monthly student loan amount, the mortgage payment,...

The sales budget for your company in the coming year is based on a quarterly growth rate of 20 percent with the first-quarter sales projection at $226 million. In addition to this basic trend, the...

An experienced male mentor is working, in a paid relationship with a younger female mentee, helping her to think through her career options, following a breakdown in her personal relationship. The...

Problem 1.1 Find the equivalent stiffness at point A. Answer: 9.20106 N/m Problem 1.2 Consider a stainless-steel metal ruler. Assume it is 12 in long, 1.25 in wide, and 0.018 in thick with an elastic...

In half a page, explain how the child protection reporting system operates. In your answer, consider reporting protocols, policies for working with other agencies and how the system responds to...

PUIDU CULTIL UIJPUJIUNT UJDX020neralne ZADUIF 1.On February 1, ABC Company sold goods to a customer for $300,000, terms 2/10,n/30. On February 4. the customer returned $100,000 of the goods. On...

Hardin Services Co. experienced the following events in 2016: 1. Provided services on account. 2. Collected cash for accounts receivable. 3. Attempted to collect an account and, when unsuccessful,...

Consider a disk with a sector size of 512 bytes, 2000 tracks per surface, 50 sectors per track, five double-sided platters, and average seek time of 10 msec. 1. What is the capacity of a track in...

If you were about to create an index on a relation, what considerations would guide your choice? Discuss: 1. The choice of primary index. 2. Clustered versus unclustered indexes. 3. Hash versus tree...

The algorithms for insertion and deletion into a B+ tree are presented as recursive algorithms. In the code for insert, for instance, a call is made at the parent of a node N to insert into (the...

Imagine representing your attitudes toward conflict visually rather than through language. What would your conflict art look like? What materials would you use? What kinds of colors would you use?...

Do you believe that if you have the right partner the two of you will live conflict free? Is it possible to find someone who presents no problems? Do you expect others to respect your property and...

Compare two conflicts in which avoiding worked in different ways one conflict you avoided eventually resolved itself, and another you avoided that ended up getting worse because you put off...