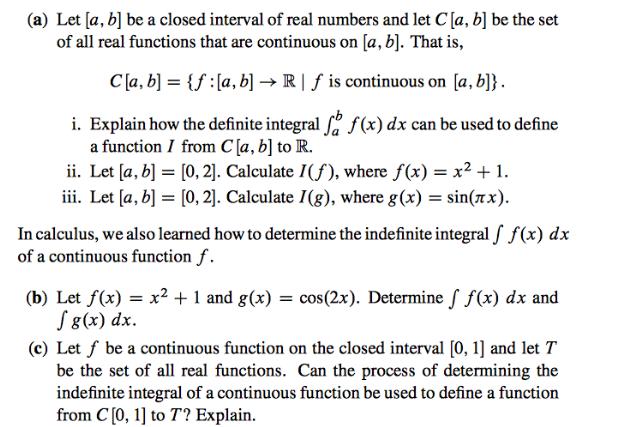
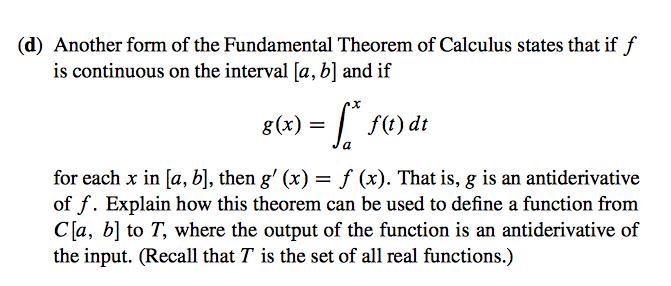
(a) Let [a, b] be a closed interval of real numbers and let C [a, b] be the set of all real functions that are continuous on [a, b]. That is, C[a, b] {f[a, b] Rf is continuous on [a, b]}. i. Explain how the definite integral fo f(x) dx can be used to define a function I from C[a, b] to R. ii. Let [a, b] = [0,2]. Calculate I(f), where f(x) = x + 1. iii. Let [a, b] = [0,2]. Calculate (g), where g(x)=sin(x). In calculus, we also learned how to determine the indefinite integral / f(x) dx of a continuous function f. (b) Let f(x) = x + 1 and g(x) = cos(2x). Determine ( f(x) dx and g(x) dx. (c) Let be a continuous function on the closed interval [0, 1] and let T be the set of all real functions. Can the process of determining the indefinite integral of a continuous function be used to define a function from C[0, 1] to T? Explain. (d) Another form of the Fundamental Theorem of Calculus states that if is continuous on the interval [a, b] and if x g(x) = ) = f s(t) dt a for each x in [a, b], then g' (x) = f (x). That is, g is an antiderivative of f. Explain how this theorem can be used to define a function from C[a, b] to T, where the output of the function is an antiderivative of the input. (Recall that T is the set of all real functions.)